Refer to our Texas Go Math Grade 8 Answer Key Pdf to score good marks in the exams. Test yourself by practicing the problems from Texas Go Math Grade 8 Lesson 11.2 Answer Key Equations with Rational Numbers.
Texas Go Math Grade 8 Lesson 11.2 Answer Key Equations with Rational Numbers
Example 1
Solve \(\frac{7}{10}\)n + \(\frac{3}{2}\) = \(\frac{3}{5}\)n + 2
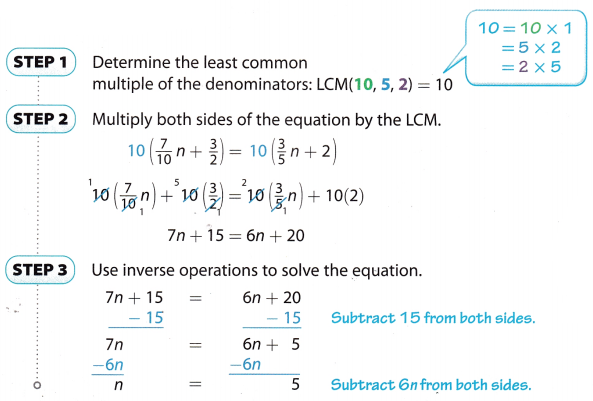
Reflect
Question 1.
What is the advantage of multiplying both sides of the equation by the least common multiple of the denominators in the first step?
Answer:
By multiplying both sides by the common multiple of the denominators in the first step, the denominator is completely divided.
Question 2.
What If? What happens in the first step if you multiply both sides by a common multiple of the denominators that are not the LCM?
Answer:
If we multiply both sides by a common multiple of the denominators that is not the LCM. we eliminate the fractions from the equation, but the coefficients in front of the variable and the constants will be greater.
Your Turn
Solve.
Question 3.
\(\frac{1}{7}\)k – 6 = \(\frac{3}{7}\)k + 4 ___________
Answer:
Given
\(\frac{1}{7}\)k – 6 = \(\frac{3}{7}\)k + 4
Determine the least common multiple of the denominators
LCM : 7
Multiply both sides of the equation by the LCM
7(\(\frac{1}{7}\)k – 6) = 7(\(\frac{3}{7}\)k + 4)
Simplify
k – 42 = 3k + 28
Subtract k from both sides
k – 42 – k = 3k + 28 – k
-42 = 2k + 28
Subtract 28 from both sides
-42 – 28 = 2k + 28 – 28
2k = -70
Divide both sides by 2
k = \(\frac{-70}{2}\) = -35
Question 4.
\(\frac{5}{6}\)y + 1 = –\(\frac{1}{2}\)y + \(\frac{1}{4}\) ___________
Answer:
Given
\(\frac{5}{6}\)y + 1 = –\(\frac{1}{2}\)y + \(\frac{1}{4}\)
Determine the least common multiple of the denominators
LCM : 12
MultipLy both sides of the equation by the LCM
12(\(\frac{5}{6}\)y + 1) = 12(-\(\frac{1}{2}\)y + \(\frac{1}{4}\))
Simplify
10y + 12 = -6y + 3
Add 6y to both sides
10y + 12 + 6y = -6y + 3 + 6y
16y + 12 = 3
Subtract 12 from both sides
16y + 12 – 12 = 3 – 12
16y = -9
Divide both sides by 16
y = \(\frac{-9}{16}=-\frac{9}{16}\)
Question 5.
Logan has two aquariums. One aquarium contains 1.3 cubic feet of water and the other contains 1.9 cubic feet of water. The water in the larger aquarium weighs 37.44 pounds more than the water in the smaller aquarium. Write an equation with a variable on both sides to represent the situation. Then find the weight of 1 cubic foot of water.
Answer:
Given
Weight of the smaller aquarium = Weight of the larger aquarium
Write the equation where x is the weight of cubic feet of water
1.3x + 37.44 = 1.9x
Subtract 1.3x from both sides
1.3x + 37.44 – 1.3x = 1.9x – 1.3x
0.6x = 37.44
Divide both sides by 0.6
x = \(\frac{37.44}{0.6}\) = 62.4
The weight of $1$ cubic foot of water is $62.4$ pounds.
Question 6.
Write a real-world problem that can be modeled by the equation
\(\frac{1}{3}\)x + 10 = \(\frac{3}{5}\)x.
Answer:
\(\frac{1}{3}\)x + 10 = \(\frac{3}{5}\)x
There is 10 cubic meter of water in pool A and water is added at a rate of \(\frac{1}{3}\) per minute
There is 0 cubic meter of water in pool B and water is added at a rate of \(\frac{3}{5}\) per minute
The equation gives the number of minutes after which the quantity of the water in two pools will be the same.
Texas Go Math Grade 8 Lesson 11.2 Guided Practice Answer Key
Question 1.
Sandy is upgrading her Internet service. Fast Internet charges $60 for installation and $50.45 per month. Quick Internet has free installation but charges $57.95 per month. (Example 2)
a. Write an equation that can be used to find the number of months after which the Internet service would cost the same.
Answer:
Write an equation for Fast Internet, where x is the number of months:
Charge per Month*Number of Month + Installation Fee
50.45x + 60
Write an equation for Quick Internet, where x is the number of months:
Charge per Month*Number of Month + Installation Fee
57.95x
Write an equation to find the number of months for which the total cost is same
50.45x + 60 = 57.95x
b. Solve the equation.
Answer:
Solve for x
50.45x + 60 = 57.95x
Subtract 50.45x from both sides
50.45x + 60 – 50.45x = 57.95x – 50.45x
7.5x = 60
Divide both sides by 7.5
x = \(\frac{60}{7.5}\) = 8
The total cost will be the same for $8 months.
Solve. (Example 1 and 2)
Question 2.
\(\frac{3}{4}\)n – 18 = \(\frac{1}{4}\)n – 4
Answer:
Given
\(\frac{3}{4}\)n – 18 = \(\frac{1}{4}\)n – 4
Determine the least common multiple of the denominators
LCM : 4
Multiply both sides of the equation by the LCM
4(\(\frac{3}{4}\)n – 18) = 4(\(\frac{1}{4}\)n – 4)
Simplify
3n – 72 = n – 16
Subtract n from both sides
3n – 72 – n = n – 16 – n
2n – 72 = 16
Add 72 from both sides
2n – 72 + 72 = 16 + 72
2n = 56
Divide both sides by 2
n = \(\frac{56}{2}\) = 28
Question 3.
6 + \(\frac{4}{5}\)b = \(\frac{9}{10}\)b
Answer:
Determine the least common multiple of the denominators:
LCM(10, 5) = 10
Multiply both sides of the equation by the LCM
10(6 – \(\frac{4}{5}\)b) = 10(\(\frac{9}{10}\))
10(6) + 10(\(\frac{4}{5}\)b) = 10(\(\frac{9}{10}\)b)
60 + 8b = 9b
60 + 8b – 8b = 9b – 8b (Subtract 8b from both sides)
60 = b
or
b = 60
Question 4.
\(\frac{2}{11}\)m + 16 = 4 + \(\frac{6}{11}\)m
Answer:
Determine the least common multiple of the denominators:
LCM = 11
Multiply both sides of the equation by the LCM
11(\(\frac{2}{11}\)m + 16) = 11(4 + \(\frac{6}{11}\)m)
11(\(\frac{2}{11}\)m) + 11(16) = 11(4) + 11(\(\frac{6}{11}\)m)
2m + 176 = 44 + 6m
(Subtract 2m from both sides) 2m + 176 – 2m = 44 + 6m – 2m
176 = 44 + 4m
(Subtract 44 from both sides.) 176 – 44 = 44 + 4m – 44
132 = 4m
(Divide both sides by 4) \(\frac{132}{4}\) = \(\frac{4m}{4}\)
33 = m
or
m = 33
Question 5.
2.25t + 5 = 13.5t + 14
Answer:
2.25t + 5 = 13.5t + 14
(Multiply both sides of the equation by 100. Multiplying by 100 clears the equation of decimals.) 100 (2.25t + 5) = 100(13.5t + 14)
225t + 500 = 1350t + 1400
(Subtract 500 from each side)225t + 500 – 500 = 1350t + 1400 – 500
225t = 1350t + 900
(Subtract 1350f from each side.)225t – 1350t = 1350t + 900 – 1350t
-1125t = 900
(Divide by -1125.) \(\frac{-1125}{-1125}\) = \(\frac{900}{-1125}\)
t = -0.8
Question 6.
3.6w = 1.6w + 24
Answer:
Given
3.6w = 1.6w + 24
Subtract 1.6w from both sides
3.6w – 1.6w = 1.6w + 24 – 1.6w
2w = 24
Divide both sides by 2
w = \(\frac{24}{2}\) = 12
Question 7.
-0.75p – 2 = 0.25p
Answer:
Given
0.75p – 2 = 0.25p
Add 0.75p from both sides
-0.75p + 0.75p – 2 = 0.25p + 0.75p
p = -2
Question 8.
Write a real-world problem that can be modeled by the equation 1.25x = 0.75x + 50. (Example 3)
Answer:
Given
1.25x = 0.75x + 50
Left side of the equation has variable minute
Cell offers Plan A for no base fee and $1.25 per minute.
The right side of the equation has variable and constant
Cell offer Plan B for $50 base fee and $0.75 per minute
The equation shows when the total cost of the plan would be equal.
Essential Question Check-In
Question 9.
How does the method for solving equations with fractional or decimal coefficients and constants compare with the method for solving equations with integer coefficients and constants?
Answer:
In solving for equations with fractional coefficients, we need to equate all fractions with their least common denominator (LCD). By multiplying the LCD in all sides of the equation, we can eliminate all fractions then proceed to solve the whole equation as integers.
Texas Go Math Grade 8 Lesson 11.2 Independent Practice Answer Key
Question 10.
Members of the Wide Waters Club pay $105 per summer season, plus $9.50 each time they rent a boat. Nonmembers must pay $14.75 each time they rent a boat. How many times would a member and a non-member have to rent a boat in order to pay the same amount?
Answer:
Members of the Wide Waters Club pay 105 doLLars per summer season, plus 9.50 dollars each time they rent a boat.
Write an expression:
105 + 9.50x
Nonmembers must pay 14.75 dollars each time they rent a boat.
Write an expression:
14.75x
Write an equation for the number of visits for which the member and nonmember would pay the same amount
105 + 9.50x = 14.75x
Solve the equation for x.
(Write the equation.) 105 + 9.50x = 14.75x
(Subtract 9.50 from both sides.) 105 + 9.5x – 9.5x = 14.75x – 9.50x
(MultipLy by 100.) 105 = 5.25x
10500 = 525x
(Divide both sides by 525.) \(\frac{10500}{525}\) = \(\frac{525x}{525}\)
20 = x
or
x = 20
The members and nonmembers will pay the same amount for 20 visits.
Question 11.
Margo can purchase tile at a store for $0.79 per tile and rent a tile saw for $24. At another store she can borrow the tile saw for free if she buys tiles therefor $1.19 per tile. How many tiles must she buy for the cost to be the same at both stores?
Answer:
Margo can purchase tile at a store for 0.79 dollars per tile and rent a tile saw for 24 dollars.
Write an expression:
0.79x + 24
At another store, she can borrow the tile saw for free if she buys tiles there for 1.19 dollars per tile.
Write an expression.
1.19x
Write an equation for the number of tiles for which costs is the same at both stores.
0.79x + 24 = 1.19x
Solve the equation for x.
(Write the equation.) 0.79x + 24 = 1.19x
(Multiply by 100.) 79x + 2400 = 119x
(Subtract 79x from both sides.) 79x + 2400 – 79x = 119x – 79x
2400 = 40x
(Divide both sides by 40.) \(\frac{2400}{40}\) = \(\frac{40x}{40}\)
60 = x
or
x = 60
Margo must buy 60 tiles for the cost to be the same at both stores.
Question 12.
The charges for two shuttle services are shown in the table. Find the number of miles for which the cost of both shuttles is the same.
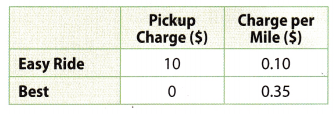
Answer:
Write an expression of the costs for the shuttles by Easy Ride.
Pickup Charge + Charge per Mile
10 + 0.10x
Write an expression of the costs for the shuttles by Best charge.
Pickup Charge + Charge per Mile
0 + 0.35x
Write an equation to find the number of miles for which the cost of both shuttles is the same.
Solve the equation for x.
(Write the equation.) 10 + 0.10x = 0.35x
(Multiply by 100) 1000 + 10x = 35x
(Subtract 10x from both sides.) 1000 + 10x – 10x = 35x – 10x
1000 = 25x
(Divide both sides by 25) \(\frac{1000}{25}\) = \(\frac{25x}{25}\)
40 = x
or
x = 40
The cost of shuttles would be the same for 40 miles.
Question 13.
Multistep Rapid Rental Car charges a $40 rental fee, $15 for gas, and $0.25 per mile driven. For the same car, Capital Cars charges $45 for rental and gas and $0.35 per mile.
a. For how many miles is the rental cost at both companies the same?
Answer:
Write the expression for cost of Rapid Rental Car for x mile
Charge per miLe*number of miles + rental fee + gas
0.25x + 40 + 15
0.25x + 55
Write the expression for cost of Capital Car for x mile
Charge per mile*number of miles + rental fee and gas
0.35x + 45
Write an equation for number of miles for which the cost of two rental cars would be same
0.25x + 55 = 0.35x + 45
Subtract 0.25x from both sides
0.25x + 55 – 0.25x = 0.35x + 45 – 0.25x
0.1x + 45 = 55
Subtract 45 from both sides
0.1x + 45 – 45 = 55 – 45
0.1x = 10
Divide both sides by 0.1
x = \(\frac{10}{0.1}\) = 100
The cost of car rentals would be the same for $100 miles
b. What is that cost?
Answer:
Let y be the total cost. Substitute 100 miles in any one of the two equations
y = 0.35x + 45
y= 0.35(100) + 45 = $80
Total cost would be $80.
Question 14.
Write an equation with the solution x = 20. The equation should have the variable on both sides, a fractional coefficient on the left side, and a fraction anywhere on the right side.
Answer:
To write the equation for the given solution, we will perform different operations going backwards from the solution to the equation.
x = 20
Multiply both sides by \(\frac{1}{3}\).
\(\frac{1}{3}\)x = \(\frac{1}{3}\) ∙ 20
Add x on both sides.
\(\frac{1}{3}\)x + x = \(\frac{20}{3}\) + x
Add 10 on both sides.
\(\frac{4}{3}\)x + 10 = \(\frac{20}{3}\) + x + 10
\(\frac{4}{3}\)x + 10 = \(\frac{50}{3}\) + x
Question 15.
Write an equation with the solution x = 25. The equation should have the variable on both sides, a decimal coefficient on the left side, and a decimal anywhere on the right side. One of the decimals should be written in tenths, the other in hundredths.
Answer:
x = 25
(Divide both sides by 50) \(\frac{x}{50}\) = \(\frac{25}{50}\)
0.02x = 0.5
(Add x to both sides.) 0.02x + x = 0.5 + x
1.02x = 0.5 + x
(Add 0.4 to both sides.) 1.02x + 0.4 = 0.5 + x + 0.4
1.02x + 0.4 = x + 0.9
Check for x = 25:
1.02(25) + 0.4 = 25 + 0.9
25.5 + 0.4 = 25.9
25.9 = 25.9
1.02x + 0.4 = x + 0.9
Question 16.
Geometry The perimeters of the rectangles shown are equal. What is the perimeter of each rectangle?
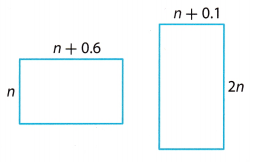
Answer:
Perimeter of the first rectangle
P = 2(n + n + 0.6) = 2(2n + 0.6) = 4 + 1.2
Perimeter of the second rectangle
P = 2(n + 0.1 + 2n) = 2(3n + 0.1) = 6n + 0.2
Since the perimeter is equal
4n + 1.2 = 6n + 0.2
Solve the equation
4n + 1.2 – 4n = 6n + 0.2 – 4n
1.2 = 2n + 0.2
2n + 0.2 – 0.2 = 1.2 – 0.2
2n = 1
n = 0.5
Since the perimeter is equal the perimeter for both rectangles would be same.
P = 4n + 1.2 = 4(0.5) + 1.2 = 3.2
Question 17.
Analyze Relationships The formula F = 1.8C + 32 gives the temperature in degrees Fahrenheit (F) for a given temperature in degrees Celsius (C). There is one temperature for which the number of degrees Fahrenheit is equal to the number of degrees Celsius. Write an equation you can solve to find that temperature and then use it to find the temperature.
Answer:
F = 1.8C + 32
let x be the temperature such that it is same in both celsius and in Fahrenheit
Then the required equation is
x = 18x + 32
subtract 1.8x from both sides
-0.8x = 32
divide by -0.8 on both sides
x = -40
So -40 degree celsius
Question 18.
Explain the Error Agustin solved an equation as shown. What error did Agustin make? What is the correct answer?
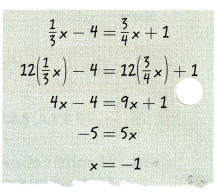
Answer:
Agustin didn’t multiply by 12 on both sides in step 2.
The correct answer is:
\(\frac{1}{3}\)x – 4 = \(\frac{3}{4}\)x + 1
(Multiply by 12.) 12(\(\frac{1}{3}\)x – 4) = 12(\(\frac{3}{4}\)x + 1)
4x – 48 = 9x + 12
(Subtract 4x from both sides.) 4x – 48 – 4x = 9x + 12 – 4x
-48 = 5x + 12
(Subtract 12 from both sides) -48 – 12 = 5x + 12 -12
(Divide by 5.) – 60 = 5x
-12 = x
or
x = -12
H.O.T. Focus on Higher Order Thinking
Question 19.
Draw Conclusions Solve the equation \(\frac{1}{2}\)x – 5 + \(\frac{2}{3}\)x = \(\frac{7}{6}\)x + 4. Explain your results.
Answer:
We are given the equation
\(\frac{1}{2}\)x – 5 + \(\frac{2}{3}\)x = \(\frac{7}{6}\)x + 4
The least common multiple of the denominators: LCM(2, 3, 6) = 6
6 ∙ (\(\frac{1}{2}\)x – 5 + \(\frac{2}{3}\)x) = (\(\frac{7}{6}\)x + 4) ∙ 6
6 ∙ \(\frac{1}{2}\)x – 6 ∙ 5 + 6 ∙ \(\frac{2}{3}\)x = 6 ∙ \(\frac{7}{6}\)x + 6 ∙ 4
3x – 30 + 4x = 7x + 24
7x – 30 = 7x + 24
Subtract 7x from both sides
7x – 30 – 7x = 7x + 24 – 7x
-30 = 24
This is not true. As we can see, the equation has no solution.
Question 20.
Look for a Pattern Describe the pattern in the equation. Then solve the equation.
0.3x + 0.03x + 0.003x + 0.0003x + …. = 3
Answer:
Firstly, to solve given task we have to understand the pattern in the equation. Looking at the equation, we can conclude that every coefficient next to variable x is 10 times smaller then the previous one. Also, we can notice that all the terms containing x are on the left side.
Therefore, we can factorize as following:
x(0.3 + 0.03 + 0.003 + …….) = 3
Looking at the expression in the parentheses, we can notice that the sum is \(0 . \overline{3}\). As we are calculating further and further, threes will pile up, so we can write the sum as \(\frac{1}{3}\).
By inserting \(\frac{1}{3}\) in the equation obtained in step 2, we get:
x ∙ \(\frac{1}{3}\) = 3 (multiplying by 3)
x = 9
Question 21.
Critique Reasoning Jared wanted to find three consecutive even integers whose sum was 4 times the first of those integers. He let k represent the first integer, then wrote and solved this equation: k + (k + 1) + (k + 2) = 4k. Did he get the correct answer? Explain.
Answer:
Using Jared’s formulated equation, k + (k + 1) + (k + 2) = 4k, the three integers that can be solved are
k + (k + 1) + (k + 2) = 4k
3k + 3 = 4k
4k – 3k = 3
k = 3 → first integer
k + 1 = 3 + 1
= 4 → second integer
k + 2 = 3 + 2
= 5 → third integer
Jared did not get the correct answer because the problem is looking for the three consecutive even integers, the obtained integers from his equation are 3, 4, and 5 which are not consecutive even integers.
Since Jared’s representation of the three consecutive integers whose sum is 4 times the first of those integers is incorrect, he should represent the following integers needed in the problem, instead.
k as the first even integer,
k + 2 as the second even integer, and
k + 4 as the third even integer
From the representation, we can formuLate an equation that is
(k + 2) + (k + 4) = 4k