Refer to our Texas Go Math Grade 7 Answer Key Pdf to score good marks in the exams. Test yourself by practicing the problems from Texas Go Math Grade 7 Lesson 1.3 Answer Key Adding Rational Numbers.
Texas Go Math Grade 7 Lesson 1.3 Answer Key Adding Rational Numbers
Reflect
Question 1.
Explain how to determine whether to move right or left on the number line when adding rational numbers.
Answer:
If the second addend is positive, we move to the right If not, we move to the left.
Your Turn
Use a number line to find each sum.
Question 2.
3 + 1\(\frac{1}{2}\) = ___________

Answer:
Start at 3
Move 1\(\frac{1}{2}\) units to the right because the second addend is positive
The result is 4.5

Question 3.
-2.5 + (-4.5) = ____________
![]()
Answer:
Start at 2.5.
Move |-4.5| = |4.5| units to the left because the second addend is negative.
The result is -7.

Reflect
Question 4.
Do -3 + 2 and 2 + (-3) have the same sum? Does it matter if the negative number is the first addend or the second addend?
Answer:
Yes, they have the same sum equal to 6. It doesn’t matter since adding is commutative.
(a + b = b + a)
Question 5.
Make a Conjecture Do you think the sum of a negative number and a positive number will always be negative? Explain your reasoning.
Answer:
No, it will not always be negative.
If the absolute value of a negative number is greater than the absolute value of a positive number, then the sum will be negative.
The sum will be positive ¡n other cases, expect when the absolute values are the same, then the sum will be equal to zero.
Your Turn
Use a number line to find each sum.
Question 6.
-8 + 5 = __________
![]()
Answer:
Start at -8.
Move 5 units to the right because the second addend is positive
The result is -3.
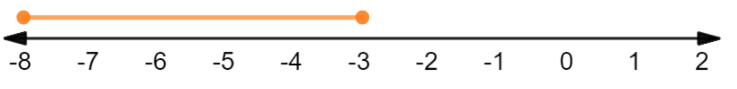
Question 7.
\(\frac{1}{2}\) + (-\(\frac{3}{4}\)) __________
Answer:
Start at \(\frac{1}{2}\).
Move |-\(\frac{3}{4}\)| = \(\frac{3}{4}\) units to the left because the second addend ¡s negative.
The result is –\(\frac{1}{4}\).
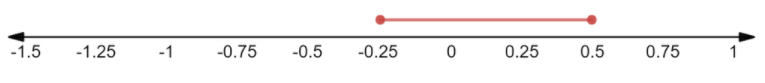
Question 8.
-1 + 7 = ____________
![]()
Answer:
Start at -1.
Move 7 units to the right because the second addend ¡s posifive
The result is 6.
Your Turn
Use a number line to find each sum.
Question 9.
2\(\frac{1}{2}\) + (-2\(\frac{1}{2}\)) = ___________
![]()
Answer:
Start at 2\(\frac{1}{2}\).
Move |-2\(\frac{1}{2}\)| = 2\(\frac{1}{2}\) units to the left because the second addend is negative.
The result is 0.
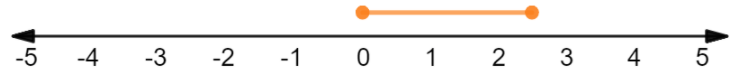
Question 10.
-4.5 + 4.5 = _________
![]()
Answer:
Start at -4.5.
Move 4.5 units to the right because the second addend is positive.
The result is 0.
Your Turn
Find each sum.
Question 11.
-1.5 + 3.5 + 2 = ____________
Answer:
Start by grouping numbers with the same sign.
= -1.5 – (3.5 + 2) ………… (1)
= -1.5 – 5.5 …………. (2)
= 4 …………. (3)
(1) Associative property
(2) Add the numbers inside the parentheses.
Question 12.
3\(\frac{1}{4}\) + (-2) + (-2\(\frac{1}{4}\)) = __________
Answer:
Start by grouping numbers with the same sign.
= 3\(\frac{1}{4}\) + ((-2) + (-2\(\frac{1}{4}\))) …………. (1)
= 3\(\frac{1}{4}\) + (-4\(\frac{1}{4}\)) ………….. (2)
= -1 …………. (3)
(1) Associative property
(2) Add the numbers inside the parentheses.
Question 13.
-2.75 + (-3.25) + 5 = _________
Answer:
Start by grouping numbers with the same sign.
= (-2.75 + (-3.25)) + 5 …………….. (1)
= -6 + 5 ………… (2)
= -1 ………… (3)
(1) Associative property
(2) Add the numbers inside the parentheses.
Question 14.
15 + 8 + (-3) = ___________
Answer:
Start by grouping numbers with the same sign.
= (15 + 8) + (-3) ……………. (1)
= 23 + (-3) ………… (2)
= 20 …………… (3)
(1) Associative property
(2) Add the numbers inside the parentheses.
Texas Go Math Grade 7 Lesson 1.3 Guided Practice Answer Key
Use a number line to find each sum. (Example 1 and Example 2)
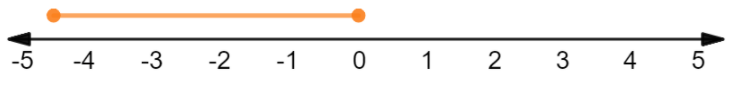
Question 1.
-3 + (-1.5) = ___________
![]()
Answer:
Start at -3.
Move |-1.5| = 1.5 units to the left because the second addend is negative.
The result is -4.5.
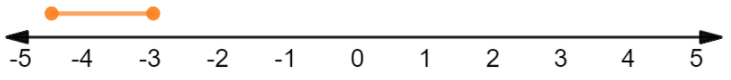
Question 2.
1.5 + 3.5 = ___________
![]()
Answer:
Start at 1.5.
Move 3.5 units to the right because the second addend is positive.
The result is 5.
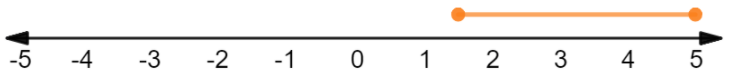
Question 3.
\(\frac{1}{4}\) + \(\frac{1}{2}\) = ___________
Answer:
Start at \(\frac{1}{4}\)
Move \(\frac{1}{2}\) units to the right because the second addend is positive.
The result is \(\frac{3}{4}\).
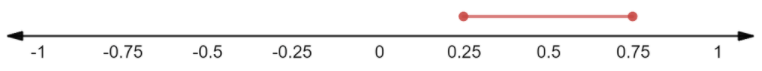
Question 4.
-1\(\frac{1}{2}\) + (-1\(\frac{1}{2}\)) = ____________
![]()
Answer:
Start at -1\(\frac{1}{2}\).
Move |-1\(\frac{1}{2}\)| = 1\(\frac{1}{2}\) units to the left because the second addend is negative.
The result is -3.
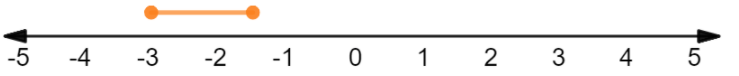
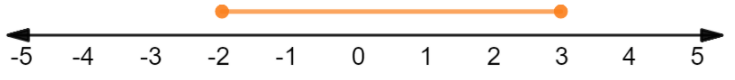
Question 5.
3 + (-5) = __________
![]()
Answer:
Start at 3.
Move |-5 | = 5 units to the left because the second addend is negative.
The resuLt is -2.
Question 6.
-1.5 + 4 = ___________
![]()
Answer:
Start at -1.5
Move 4 units to the right because the second addend ¡s posit ive
The result is 2.5.
Question 7.
Victor borrowed $21.50 from his mother to go to the theater. A week later, he paid her $21.50 back. How much does he still owe her? (Example 3)
Answer:
Use a positive number to represent money Victor borrowed from his mother, and a negative number to represent money Victor paid back.
Find 21.5 + (-21.5).
Start at 21.5.
Move |-21.5| = 21.5 units to the left because the second addend is negative.
The result is 0. That means he no longer owes her money.
Victor owes his mother $0.
Question 8.
Sandra used her debit card to buy lunch for $8.74 on Monday. On Tuesday, she deposited $8.74 back into her account. What is the overall increase or decrease in her bank account? (Example 3)
Answer:
We use a positive number to represent money she deposited into her account, and a negative number to represent money she spent to buy Lunch.
Find -8.74 + 8.74.
Start at – 8.74.
Move 8.74 units to the right because the second addend is positive.
The result is 0. That means there is no increase or decrease.
There is no increase or decrease.
Find each sum without using a number line. (Example 4)
Question 9.
2.75 + (-2) + (-5.25) = __________
Answer:
Start by grouping numbers with the same sign.
= 2.75 + ((-2) + (-5.25)) …………. (1)
= 2.75 + (-7.25) …………. (2)
= -4.5 ………… (3)
(1) Associative property
(2) Add the numbers inside the parentheses.
Question 10.
-3 + (1\(\frac{1}{2}\)) + (2\(\frac{1}{2}\)) = ____________
Answer:
Start by grouping numbers with the same sign.
= -3+ ((1\(\frac{1}{2}\)) + (2\(\frac{1}{2}\)) ………… (1)
= -3 + (4) ………….(2)
= 1 …………. (3)
(1) Associative property
(2) Add the numbers inside the parentheses.
Question 11.
-12.4 + 9.2 + 1 = ___________
Answer:
Start by grouping numbers with the same sign.
= -12.4 + (9.2 + 1) ………… (1)
= -12.4 + (10.2) ………….(2)
= -2.2 …………. (3)
(1) Associative property
(2) Add the numbers inside the parentheses.
Question 12.
-12 + 8 + 13 = ____________
Answer:
Start by grouping numbers with the same sign.
= -12 + (8 + 13) ………… (1)
= -12 + (21) ………….(2)
= 9 …………. (3)
(1) Associative property
(2) Add the numbers inside the parentheses.
Question 13.
4.5 + (-12) + (-4.5) = ____________
Answer:
Start by grouping numbers with the same sign.
= 4.5 + ((-12) + (-4.5)) ………… (1)
= 4.5 + (-16.5) ………….(2)
= -12 …………. (3)
(1) Associative property
(2) Add the numbers inside the parentheses.
Question 14.
\(\frac{1}{4}\) + (-\(\frac{3}{4}\)) = __________
Answer:
= – \(\frac{2}{4}\) …………… (1)
= – \(\frac{1}{2}\) …………… (2)
(1) Cancel fraction.
Question 15.
-4\(\frac{1}{2}\) + 2 = _____________
Answer:
= -2\(\frac{1}{2}\)
Question 16.
-8 + (-1\(\frac{1}{8}\)) = ___________
Answer:
= -9\(\frac{1}{8}\)
Essential Question Check-In
Question 17.
How can you use a number line to find the sum of -4 and 6?
Answer:
Start at the -4.
Move |6| = 6 units to the right because the second added is positive.
The result is 2.
Texas Go Math Grade 7 Lesson 1.3 Independent Practice Answer Key
Question 18.
Samuel walks forward 19 steps. He represents this movement with a positive 19. How would he represent the opposite of this number?
Answer:
He would represent the opposite of positive 19 with negative 19: -19
Question 19.
Julia spends $2.25 on gas for her lawn mower. She earns $15.00 mowing her neighbor’s yard. What is Julia’s profit?
Answer:
Julia earns $15.00, and spends/Loses $2.25. That means we have to add $15 to her gas expenses of $2.25.
-2.25 + 15 = 12.75
Julia’s profit is $12.75
Question 20.
A submarine submerged at a depth of -35.25 meters dives an additional 8.5 meters. What is the new depth of the submarine?
Answer:
If a submarine is at a depth of -35.25 meters, and dives an additional 8.5 meters, that means we have to add another -8.5 meters to original depth.
-35.25 + (-8.5) = -43.75
New depth of the submarine is -43.75 meters.
Question 21.
Renee hiked for 4\(\frac{3}{4}\) miles. After resting, Renee hiked back along the same route for 3\(\frac{1}{4}\) miles. How many more miles does Renee need to hike to return to the place where she started?
Answer:
We conclude that Renee has to hike 4\(\frac{3}{4}\) miles back. She already hiked 3\(\frac{1}{4}\) in opposite direction. To find out how many miles more Renee has to hike, we have to add negative 3\(\frac{1}{4}\) to 4\(\frac{3}{4}\).
4\(\frac{3}{4}\) + (-3\(\frac{1}{4}\)) = 1\(\frac{2}{4}\)
= 1\(\frac{1}{2}\) miles
(1) Cancel fraction.
Question 22.
Geography The average elevation of the city of New Orleans, Louisiana, is 0.5 m below sea level. The highest point in Louisiana is Driskill Mountain at about 163.5 m higher than New Orleans. How high is Driskill Mountain?
Answer:
The elevation of New Orleans is -0.5 meters. Driskill Mountain is at 163.5 m higher than New OrLeans To find out how high is Driskitl Mountain, we have to add -0.5 to 163.5.
163.5 + (-0.5) = 163
Driskill mountain is 163 m high.
Question 23.
Problem Solving A contestant on a game show has 30 points. She answers a question correctly to win 15 points. Then she answers a question incorrectly and loses 25 points. What is the contestant’s final score?
Answer:
If the contestant starts with 30 points, then wins 15 points and Lastly loses 25 points, or wins -25 points, we add 30, 15 and -25 together
30 + 15 + (-25) = 45 + (-25)
= 20
Contestant’s final score is 20 points.
Financial Literacy Use the table for 24-26. Kameh owns a bakery. He recorded the bakery income and expenses in a table.

Question 24.
In which months were the expenses greater than the income? Name the month and find how much money was lost.
Answer:
We can easily Look at the table and find where the expenses are greater than the income.
These months are: January, February.
Calculate each month separately by adding expence and negative income.
January:
1290.60 + (-1205) = 85.60
February:
1345.44 + (-1183) = 162.44
January: $85.60, February: $162.44
Question 25.
In which months was the income greater than the expenses? Name the months and find how much money was gained each of those months.
Answer:
We can easiLy Look at the tabLe and find where the income is greater than the expenses.
These months are: June, JuLy, August
CaLcuLate each month separately by adding income and negative expense.
June: 2413 + (-2106.23) = 309.77
JuLy: 2260 + (-1958.50) = 301.50
August: 2183 + (-1845.12) = 337.88
June: $309.77, July: $301.50, August: $337.88
Question 26.
Communicate Mathematical Ideas If the bakery started with an extra $250 from the profits in December, describe how to use the information in the table to figure out the profit or loss of money at the bakery by the end of August. Then calculate the profit or loss.
Answer:
To determine the profit of these 6 months. we add how much has bakery gained/lost money per month.
-85.60 + (- 162.44) + 0+ 309.77 + 301.50 + 337.88 = 218.01 + 0 + 309.7 + 301.50 – 337.88
= -248.04 + 309.77 + 301.50 + 337.88
= 61.73 + 301.50 + 337.88
= 363.23 + 337.88
= 710.11
Finally, we add profit from december ($250) and profit/loss of these 6 months.
250 + 710.11 = 951.11
Bakery has a profit of $951.11.
Question 27.
Vocabulary -2 is the ______________ of 2.
Answer:
-2 is the opposite of 2.
Question 28.
The basketball coach made up a game to play where each player takes 10 shots at the basket. For every basket made, the player gains 10 points. For every basket missed, the player loses 15 points.
a. The player with the highest score sank 7 baskets and missed 3. What was the highest score?
Answer:
First, we have to add 10 points 7 times, then add -15 points 3 times.
10 + 10 + 10 + 10 + 10 + 10 + 10 + (-15) + (-15) + (-15) = ………. = 70 + (-35)
= 35
b. The player with the lowest score sank 2 baskets and missed 8. What was the lowest score?
Answer:
10 + 10 + (-15) + (-15) + (-15) + (-15) + (-15) + (-15) + (-15) + (-15) = ……. = 20 + (-120)
= -100
c. Write an expression using addition to find out what the score would be if a player sank 5 baskets and missed 5 baskets.
Answer:
First, we have to add 10 points 5 times, then add -15 points 5 times.
10 + 10 + 10 + 10 + 10 + (-15) + (-15) + (-15) + (-15) + (-15) = ….. = 50 + (-105)
= -65
H.O.T.S Focus on Higher Order Thinking
Question 29.
Communicate Mathematical Ideas Explain the different ways it is possible to add two rational numbers and get a negative number.
Answer:
We can get a negative number if both addends are negative. Also, if only one addend is negative, then its absolute value must be greater than the second addend’s absolute value.
Question 30.
Explain the Error A student evaluated -4 + x for x = -9\(\frac{1}{2}\) and got the answer of 5\(\frac{1}{2}\). What might the student have done wrong?
Answer:
The student might have overseen that the x is negative and calculated as the x was equal to 9\(\frac{1}{2}\).
Question 31.
Draw Conclusions Can you find the sum [5.5 + (-2.3)] + (-5.5 + 2.3) without performing any additions?
Answer:
Yes, we can find the sum without performing any addition.
We can see that the square brackets don’t have any use in the expression.
Then, we can move -5.5 to its own bracket and separate it from 2.3, nothing will change.
Now we have:
5.5 + (-2.3) + (-5.5) + 2.3
Now, reorganize the expression by changing places of the 2 inner addends.
5.5 + (-5.5) + (-2.3) + 2.3
It’s easy to see that we are adding 2 pairs of opposite numbers. Since the sum of opposite numbers is equal to zero, the sum of our expression is equal to zero.