Refer to our Texas Go Math Grade 6 Answer Key Pdf to score good marks in the exams. Test yourself by practicing the problems from Texas Go Math Grade 6 Lesson 5.4 Answer Key Applying Addition and Subtraction of Integers.
Texas Go Math Grade 6 Lesson 5.4 Answer Key Applying Addition and Subtraction of Integers
Your Turn
Question 1.
Anna is in a cave 40 feet below the cave entrance. She descends 13 feet, then ascends 18 feet, Find her new position relative to the cave entrance.
Answer:
The expression that models Anna’s change of position is:
– 40 – 13 + 18
First find the difference:
– 40 – 13
Subtracting 13 is equal to adding its additive inverse -13 so you can find sum:
– 40 + (- 13)
Find absolute values of given integers:
|- 40| = 40 and |- 13| = 13
Sum that absolute values:
40 + 13 = 53
Result would be 53 or – 53, depending on sign of given integers (both positive then +, both negative then -).
Given integers are negative so result is – 53.
Now, find the sum:
– 53 + 18
Find absolute values and subtract lesser absolute value from greater:
|- 53| – |18| = 35
Sum would be 35 or – 35, depending on signs of given integers.
Use the sign of integer with greater absolute value to find the sum:
– 53 + 18 = – 35
Therefore, Anna’s new position iS:
– 40 – 13 + 18 = – 35
Anna’s new position is – 35 feet
Find the difference – 40 – 13 using rules for subtracting integers and then add 18 using rules for adding integers.
Reflect
Question 2.
Communicate Mathematical Ideas Describe a different way to find the change in Irene’s account.
Answer:
You can find the change in Irenne’s account {without using the Commutative Property.
First, find sum:
– 160 + 125
Find absolute values and subtract lesser absolute value from greater:
|- 160| – |125| = 35
Sum would be 35 or – 35, depending on signs of given integers.
Use the sign of number with greater absolute value to find the sum:
– 160 + 125 = – 35
Then, calculate:
– 35 + (- 40)
Find absolute values of given integers:
|- 35| = 35 and |- 40| = 40
Sum that absolute values:
35 + 40 = 75
Given integers are negative so final result is – 75.
You can find the change in Irenne’s account without using the Commutative Property.
Find sum – 160 + 125 using rules for adding integers and then add – 40 to that sum.
Your Turn
Question 3.
Alex wrote checks on Tuesday for $35 and $45. He also made a deposit in his checking account of $180. Find the overall change in the amount in his checking account.
Answer:
Use negative integers to represent the checks that Alex wrote and positive integers to represent the deposit he made.
The expression that modeLs change in his account is:
– 35 + (- 45) + 180
First, find sum:
– 35 + (- 45)
Find absolute values of given integers:
|- 35| = 35 and |- 45| = 45
Sum that absolute values:
35 + 45 = 80
Given integers are negative so final result is -80.
Then, calculate:
– 80 + 180
Find absolute values and subtract lesser absolute value from greater:
|180| – |- 80| = 100
Sum would be 100 or – 100. depending on signs of given integers.
Use the sign of number with greater absoLute vaLue to find the sum:
– 80 + 180 = 100
Therefore, the change in his account is
$100
The overall change in his account is $100.
Find the difference – 35 – 45 using rules for subtracting integers and then add 180 to the result using rules for adding integers.
Your Turn
Question 4.
Jim and Carla are scuba diving. Jim started out 10 feet below the surface. He descended 18 feet, rose 5 feet, and descended 12 more feet. Then he rested. Carla started out at the surface. She descended 20 feet, rose 5 feet, and descended another 18 feet. Then she rested. Which person rested at a greater depth? Explain.
Answer:
Use negative integer to represent Jim’s start because he started out below the surface.
Subtract integer if he descended and add integer if he rose.
The expression you get is:
– 10 – 18 + 5 – 12
Subtracting 18 and 12 is equal to adding additive inverses – 18 and – 12 so you can find sum:
– 10 + (- 18) + 5 + (- 12)
Use Commutative Property and Associative Property to simplify addition:
– 10 + (- 18) + (- 12) + 5
– 10 – ((- 18) + (- 12)) + 5
Use rules for adding integers to get the sum in brackets and to get the final result:
– 10 + (-30) + 5
– 40 + 5 = – 35
Therefore, Jim’s position is:
– 10 – 18 + 5 – 12 = – 35
0 represents Carla’s start because she started out at the surface.
Subtract integer if she descended and add integer if she rose.
The expression you get is:
0 – 20 + 5 – 18
Subtracting 20 and 18 is equal to adding additive inverses – 20 and – 18 so you can find sum:
0 + (- 20) + 5 + (- 18)
Use Commutative Property to simplify addition:
o + (- 20) + (- 18) + 5
Use rules for adding integers to get the final result:
– 20 + (- 18) + 5
– 38 + 5 = – 33
therefore, Carla’s position is:
0 – 20 + 5 – 18 = – 33
– 35 < – 33
Jim rested at a greater depth because his position white resting was 35 feet below the surface.
Carla’s position while resting was 33 feet below the surface.
Texas Go Math Grade 6 Lesson 5.4 Guided Practice Answer Key
Write an expression. Then find the value of the expression.
Question 1.
Tomas works as an underwater photographer. He starts at a position that is 15 feet below sea level. He rises 9 feet, then descends 12 feet to take a photo of a coral reef. Write and evaluate an expression to find his position relative to sea level when he took the photo.
Answer:
Use negative integer to represent his position at start because he starts out below sea level.
Subtract integer if he descends and add integer if he rises.
The expression you get is:
– 15 + 9 – 12
Subtracting 12 is equal to adding its additive inverse – 12 so you can find sum:
– 15 + 9 + (- 12)
Use Commutative Property to simplify addition:
– 15 + (- 12) + 9
Use rules for adding integers to get the final result:
– 15 + (- 12) + 9
– 27 + 9 = – 18
Therefore, his position is:
– 15 + 9 – 12 = – 18
Tomas was 18 feet below sea level when he took the photo.
The expression that models his position is – 15 + 9 – 12.
Question 2.
The temperature on a winter night was – 23 °F. The temperature rose by 5 °F when the sun came up. When the sun set again, the temperature dropped by 7 °F. Write and evaluate an expression to find the temperature after the sun set.
Answer:
Add integer if temperature rose and subtract integer if temperature dropped.
The expression you get is:
– 23 + 5 – 7
Subtracting 7 is equal to adding its additive inverse -7 so you can find sum:
– 23 + 5 + (- 7)
Use Commutative Property to simplify addition:
– 23 + (- 7) + 5
Use rules for adding integers to get the final result:
– 23 + (- 7) + 5
– 30 + 5 = – 25
Therefore, the temperature after sun set was:
– 23 + 5 – 7 = – 25
The temperature after sun set was – 25 °F.
The expression that models temperature is – 23 + 5 – 7.
Question 3.
Jose earned 50 points in a video game. He lost 40 points, earned 87 points, then lost 30 more points. Write and evaluate an expression to find his final score in the video game.
Answer:
Add integer if Jose earned points and subtract integer if he lost points.
The expression you get is:
50 – 40 + 87 – 30
Subtracting 40 and 30 is equal to adding additive inverses – 40 and – 30 so you can find sum:
50 + (- 40) + 87 + (- 30)
Use Commutative Property and Associative Property to simplify addition:
50 + 87 + (- 40) + (- 30)
(50 + 87) + ((- 40) + (- 30))
Use rules for adding integers to get the final result:
137 + (- 70) = 67
Therefore, his final score is:
50 – 40 + 87 – 30 = 67
Jose’s final score is 67 points
The expression that models his score is 50 – 40 + 87 – 30.
Find the value of each expression.
Question 4.
– 6 + 15 + 15 = ____________
Answer:
The expression is:
– 6 + 15 + 15
Use Associative Property to simplify addition:
– 6 + (15 + 15)
Find the result in brackets first:
– 6 – 30
Use rules for adding integers to get the final result:
– 6 + 30 = 24
The value is 24.
Use Associative Property and rules for adding integers to find the result
Question 5.
9 – 4 – 17 = _______
Answer:
The expression is:
9 – 4 – 17
Subtracting 4 and 17 is equal to adding additive inverses – 4 and – 17 so you can find sum:
9 + (- 4) + (- 17)
Use Associative Property to simplify addition:
9 + ((- 4) + (- 17))
Find the result in brackets first:
9 + (- 21)
Use rules for adding integers to get the final result:
9 + (- 21) = – 12
The value is – 12.
Use Associative Property and rules for adding integers to find the value of expression.
Question 6.
50 – 42 + 10 = _________
Answer:
The expression is:
50 – 42 + 10
Subtracting 42 is equal to adding its additive inverse -42 so you can find sum:
50 + (- 42) + 10
Use Commutative Property to simplify addition:
50 + 10 + (- 42)
Use Associative Property and rules for adding integers to get the final result:
60 + (- 42) = 18
The value is 18.
Use Commutative Property, Associative Property and rules for adding integers to find the value of expression.
Question 7.
6 + 13 + 7 – 5 = ____________
Answer:
The expression is:
6 + 13 + 7 – 5
Subtracting 5 is equal to adding its additive inverse – 5 so you can find sum:
6 + 13 + 7 + (- 5)
Use Associative Property to simplify addition:
6 + (13 + 7) + (- 5)
Find the result in brackets first:
6 + 20 + (- 5)
Use Associative Property and rules for adding integers to get the final result:
26 + (- 5) = 21
The value is 21.
Use Associative Property and rules for adding integers to find the value of expression.
Question 8.
65 + 43 – 11 = _____________
Answer:
The expression is:
65 + 43 – 11
Subtracting 11 is equal to adding its additive inverse – 11 so you can find sum:
65 + 43 + (- 11)
Use Associative Property and rules for adding integers to get the final result:
108 + (- 11) = 97
The value is 97.
Use Associative Property and rules for adding integers to find the value of expression.
Question 9.
– 35 – 14 + 45 + 31 = _____________
Answer:
The expression is:
– 35 – 14 + 45 + 31
Subtracting 14 is equal to adding its additive inverse – 14 so you can find sum:
– 35 + (- 14) + 45 + 31
use Associative Property to simplify addition:
(- 35 + (- 14)) + (45 + 31)
Find results in brackets using rules for adding integers:
– 49 + 76
Use rules for adding integers to get the final result:
– 49 + 76 = 27
The value is 27.
Use Associative Property and rules for adding integers to find the value of expression.
Determine which expression has a greater value.
Question 10.
– 12 + 6 – 4 or – 34 – 3 + 39
Answer:
First expression is:
-12 + 6 – 4
Subtracting 4 is equal to adding its additive inverse – 4 so you can find sum:
– 12 + 6 + (- 4)
Use Commutative Property to simplify addition:
– 12 + (- 4) + 6
Use Associative Property and rules for adding integers to get the final result:
– 16 + 6 = – 10
Second expression is:
– 34 – 3 + 39
Subtracting 3 is equal to adding its additive inverse – 3 so you can find sum:
– 34 + (- 3) + 39
Use Associative Property and rules for adding integers to get the final result:
– 37 + 39 = 2
Compare values of first and second expression:
– 10 < 2
The expression – 34 – 3 + 39 has greater value.
The expression – 34 – 3 + 39 has greater value, its value is 2.
VaLue of expression – 12 + 6 – 4 is – 10.
Use Associative Property, Commutative Property and rules for adding integers to find values of expressions.
Question 11.
21 – 3 + 8 or – 14 + 31 – 6
Answer:
First expression is:
21 – 3 + 8
Subtracting 3 is equal to adding its additive inverse -3 so you can find sum:
21 + (- 3) + 8
Use Commutative Property to simplify addition:
21 + 8 + (- 3)
Use Associative Property and rules for adding integers to get the final result:
29 + (- 3) = 26
Second expression is:
– 14 + 31 – 6
Subtracting 6 is equal to adding its additive inverse – 6 so you can find sum:
– 14 + 31 + (- 6)
Use Commutative Property to simplify addition:
– 14 + (- 6) + 31
Use Associative Property and rules for adding integers to get the final result
– 20 + 31 = 11
Compare values of first and second expression:
26 > 11
The expression 21 – 3 + 8 has greater value
The expression 21 – 3 + 8 has greater value, its value is 26.
Value of expression 14 + 31 – 6 is 11.
Use Commutative Property Associative Property and rules for adding integers to find values of expressions.
Essential Question Check-In
Question 12.
Explain how you can find the value of the expression – 5 + 12 + 10 – 7.
Answer:
The expression is:
– 5 + 12 + 10 – 7
You can use Commutative Property, Associative Property and rules for adding and subtracting integers to find value of that expression.
Subtracting 7 is equal to adding its additive inverse – 7 so you can find sum:
– 5 + 12 + 10 + (- 7)
Use Associative Property:
– 5 + (12 + 10) + (- 7)
Calculate sum in brackets first:
– 5 + 22 (- 7)
Now, use Commutative Property once again:
– 5 + (- 7) + 22
Use Associative Property and rules for adding integers to get the final result:
– 12 + 22 = 10
You can use Commutative Property, Associative Property and rules for adding and subtracting integers to find value of that expression.
Its value is 10.
Question 13.
Sports Cameron is playing 9 holes of golf. He needs to score a total of at most 15 over par on the last four holes to beat his best golf score. On the last four holes, he scores 5 over par, 1 under par, 6 over par, and 1 under par.
a. Write and find the value of an expression that gives Cameron’s score for 4 holes of golf.
Answer:
Add integer if he scores over par and subtract integer if he scores under par.
The expression you get S:
5 – 1 + 6 – 1
Subtracting 1 is equal to adding its additive inverse – 1 so you can find sum:
5 + (- 1) + 6 + (- 1)
Use Commutative Property to simpLify addition:
5 + 6 + (- 1) + (- 1)
Use Associative Property and rules for adding integers to get the final result:
(5 + 6) + ((- 1) + (- 1))
11 + (- 2) = 9
His total score is 9 over par.
b. Is Cameron’s score on the last four holes over or under par?
Answer:
Cameron’s score on those four holes is over par.
c. Did Cameron beat his best golf score?
Answer:
Compare his score with given condition
15 over par
9 < 15
He did not beat his best score.
Question 14.
Herman is standing on a ladder that is partly in a hole. He starts out on a rung that is 6 feet under ground, climbs up 14 feet, then climbs down 11 feet. What is Herman’s final position, relative to ground level?
Answer:
Start with negative integer because he is under ground. Add integer if he climbs up and subtract integer if he
climbs down
The expression you get is:
– 6 + 14 – 11
Subtracting 11 is equal to adding its additive inverse -11 so you can find sum:
– 6 + 14 + (- 11)
Use Commutative Property to simplify addition:
– 6 + (- 11) + 14
Use Associative Property and rules for adding integers to get the final result:
– 17 + 14 = – 3
Herman’s final position is 3 feet under ground
Find value of expression – 6 + 14 – 11 using rules for adding integers.
Question 15.
Explain the Error Jerome tries to find the value of the expression 3 – 6 + 5 by first applying the Commutative Property. He rewrites the expression as 3 – 5 + 6. Explain what is wrong with Jerome’s approach.
Answer:
He tried to find value of expression:
3 – 6 + 5
Jerome applied the Commutative Property only on absolute values 6 and 5 without including signs of given numbers.
He was supposed to change places of – 6 and 5. In that case, he would get the expression:
3 + 5 – 6
Then he would be able to find the correct result.
To find the result, use Associative Property and rules for adding integers:
8 + (- 6) = 2
Jerome applied the Commutative Property only on absoLute vaLues 6 and 5 without including signs of given numbers.
He was supposed to change places of – 6 and 5.
Question 16.
Lee and Barry play a trivia game in which questions are worth different numbers of points. If a question is answered correctly, a player earns points. If a question is answered incorrectly, the player loses points. Lee currently has – 350 points.
a. Before the game ends, Lee answers a 275-point question correctly, a 70-point question correctly, and a 50-point question incorrectly. Write and find the value of an expression to find Lee’s final score.
Answer:
Start with – 350, add integer if lees answer is correct and subtract integer if his answer is incorrect.
The expression you get is:
– 350 + 275 – 70 – 50
Subtracting 50 is equal to adding its additive inverse – 50 so you can find sum:
– 350 + 275 + 70 + (- 50)
Use Associative Property to simplify addition:
– 350 + (275 + 70) + (- 50)
Find the sum in brackets first:
– 350 + 345 + (- 50)
Use Commutative Property
– 350 + (- 50) + 345
Use Associative Property and rules for adding integers to get the final result:
-400 + 345 = -55
Lee’s final score is – 55 points.
b. Barry’s final score is 45. Which player had the greater final score?
Answer:
Compare Lees and Barry’s scores:
– 55 < 45
Barry had greater final score.
Question 17.
Multistep Rob collects data about how many customers enter and leave a store every hour. He records a positive number for customers entering the store each hour and a negative number for customers leaving the store each hour.
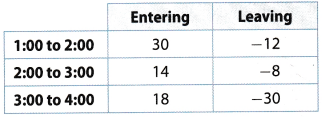
a. During which hour did more customers leave than arrive?
Answer:
Sum number of customers entering and number of customers leaving the store for each hour.
First expression is:
30 + (-12)
Use rules for adding integers to find the sum:
30 + (- 12) = 18
Second expression is:
14 +(- 8)
Use rules for adding integers to find the sum:
14 + (- 8) = 6
Third expression is:
18 + (- 30)
Use rules for adding integers to find the sum:
18 + (- 30) = – 12
Negative number represents number of customers leaving the store so during the last hour more customers left store than arrived.
b. There were 75 customers in the store at 1:00. The store must be emptied of customers when it closes at 5:00. How many customers must leave the store between 4:00 and 5:00?
Answer:
Start with 75 and add numbers you got in part a for each hour to calculate number of customers that are in the store at 4 : 00.
the expression you get is:
75 + 18 + 6 + (- 12)
Use Associative Property and rules for adding integers to get the final result:
(75 + 18) + 6 + (- 12)
93 – 6 + (- 12)
99 + (- 12) = 87
87 customers must leave the store between 4 : 00 and 5 : 00.
The table shows the changes in the values of two friends savings accounts since the previous month.
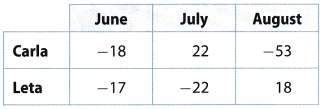
Question 18.
Carla had $100 in her account in May. How much money does she have in her account in August?
Answer:
Start with 100 and add integers that represent change in Carla’s account for each month.
The expression you get is:
100 + (- 18) + 22 + (- 53)
Use Commutative Property to simplify addition:
100 + 22 + (- 18) + (- 53)
Use Associative Property and rules for adding integers to get the final result:
(100 + 22) + ((-18) + (- 53))
122 + (- 71) = 51
Carla has $51 in her account in August.
Carla has $51 in her account in August.
Use Commutative Property, Associative Property and rules for adding integers to find the result.
Question 19.
Leta had $45 in her account in May. How much money does she have in her account in August?
Answer:
Start with 45 and add integers that represent change in Leta’s account for each month.
The expression you get is:
45 + (- 17) + (- 22) + 18
Use Associative Property to simplify addition:
45 + ((- 17) + (- 22)) + 18
Find the sum in brackets first:
45 + (- 39) + 18
Use Commutative Property:
45 + 18 + (- 39)
Use Associative Property and rules for adding integers to get the finaL resuLt:
(45 + 18) – (- 39)
63 + (- 39) = 24
Leta has $24 in her account in August
Leta has $24 in her account in August
Use Commutative Property, Associative Property and rules for adding integers to find the result.
Question 20.
Analyze Relationships Whose account had the greatest decrease in value from May to August?
Answer:
Carla had $ 100 in her account in May and she has $ 51 in August. Find the difference:
100 – 51
to calculate decrease from May to August.
Subtracting 51 is equal to adding its additive inverse – 51 so you can find sum:
100 + (- 51)
Use rules for adding integers to get the final result:
100 + (- 51) = 49
Leta bad $ 45 in her account in May and she has $ 24 in August. Find the difference:
45 – 24
to calculate decrease from May to August.
Subtracting 24 is equal to adding its additive inverse – 24 so you can find sum:
45 + (- 24)
Use rules for adding integers to get the final result:
45 +(- 24) = 21
49 > 21
Carla’s count had the greatest decrease in value.
Carla’s account had the greatest decrease in value.
Carla’s account had $49 decrease in value and Leta’s account had $21 decrease.
H.O.T Focus On Higher Order Thinking
Question 21.
Represent Real-World Problems Write and solve a word problem that matches the diagram shown.
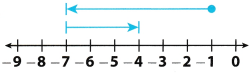
Answer:
Tom played a game in which a player gets or loses points in each turn. His score after first turn was – 1 point. He lost 6 points in his second turn and got 3 points in last, third turn. What was his final score after third turn?
Start with – 1, subtract integer if he lost points and add integer if he got points.
The expression you get is:
– 1 – 6 + 3
Subtracting 6 is equal to adding its additive inverse – 6 so you can find sum:
– 1 + (- 6) + 3
Use Associative Property to simplify addition:
(- 1 + (- 6)) + 3
Find the sum in brackets and then add 3. Use number line to find the result.
Start at – 1 and move 6 units to the left to represent adding – 6.
Then, move 3 units to the right to represent adding 3.
Read the result from number line below.
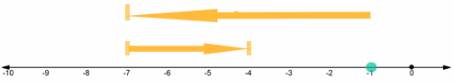
Tom’s final score after third turn was – 4 points.
Tom played a game in which a player gets or loses points in each turn. His score after first turn was – 1 point. He lost 6 points in his second turn and got 3 points in last, third run. What was his final score after three turns?
His final score after third turn was 4 points.
Question 22.
Critical Thinking Mary has $10 in savings. She owes her parents $50. She does some chores and her parents pay her $12. She also gets $25 for her birthday from her grandmother. Does Mary have enough money to pay her parents what she owes them? If not, how much more money does she need? Explain.
Answer:
Calculate the sum of amount Mary has in savings and amounts she gets from parents and grandmother and compare that sum with the amount she owes her parents.
The sum you need to find is:
10 + 12 + 25
Use Associative Property and rules for adding integers to get the final result:
(10 + 12) + 25
22 + 25 = 47
Compare that sum with $50:
47 < 50
Miry doesn’t have enough money to pay her parents.
She needs $3 more to pay her parents because she has $3 less than $50, the amount she owes.
Mary doesn’t have enough money to pay her parents because she has $3 less than $50, the amount she owes.
She needs $3 more to pay her parents.
Question 23.
Draw Conclusions An expression involves subtracting two numbers from a given first number. Under what circumstances will the value of the expression be negative? Give an example.
Answer:
The expression is:
a – b – c
The value of this expression will be negative if the sum:
b + c
is greater than first number, a.
For example, find the value of an expression:
4 – 5 – 1
Subtracting 5 and 1 is equal to adding additive inverses – 5 – 1 so you can find sum:
4 + (- 5) + (- 1)
Use Associative Property and rules for adding integers to find that sum:
4 + (- 5 + (- 1))
4 + (- 6) = – 2
Compare 4 and sum 5 + 1:
4 < 6
The value of the expression will be negative if the sum b + c is greater than first number, a.
For example, the value of an expression 4 – 5 – 1 is – 2 where 4 is lesser than 5 + 1.