Refer to our Texas Go Math Grade 3 Answer Key Pdf to score good marks in the exams. Test yourself by practicing the problems from Texas Go Math Grade 3 Lesson 2.5 Answer Key Write Fractions.
Texas Go Math Grade 3 Lesson 2.5 Answer Key Write Fractions
Essential Question
How can you write a fraction as a sum of unit fractions with the some denominator?
Unlock the Problem
Liz made a carrot cake and cut it into 4 equal slices. She ate 1 slice, or \(\frac{1}{4}\) of the cake. Her friends, Tommy and Renee, each ate \(\frac{1}{4}\) of the cake. What fraction of the cake did Liz and her friends eat?
Answer:
\(\frac{2}{4}\)
Explanation:
Liz ate \(\frac{1}{4}\) and her friends Tommy, Renee each ate \(\frac{2}{4}\) of the cake. The fraction of the cake Liz and her friends eat is \(\frac{3}{4}\).
Remember
A unit fraction names 1 equal part of a whole. It has 1 as its numerator. \(\frac{1}{6}\) is a unit fraction.
Write the fraction represented by the sum of unit fractions.
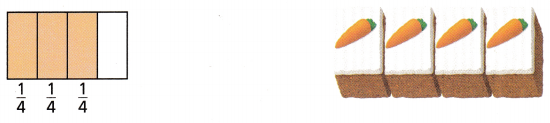
3 fourths of the rectangle is shaded.
\(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\) = ____
So, Liz and her friends ate ___ of the cake.
Answer:
\(\frac{3}{4}\)
So, Liz and her friends ate \(\frac{3}{4}\) of the cake.
Answer:
\(\frac{3}{4}\) of the rectangle is shaded.
The fraction represented by the sum of unit fractions is \(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\) = \(\frac{3}{4}\)
Hence Liz and her friends ate \(\frac{3}{4}\) of the cake.
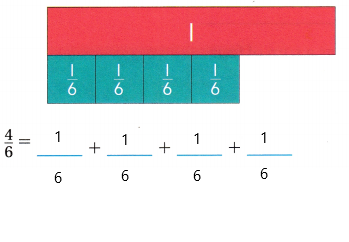
Mr. Rivera made a pan of brownies. He cut the pan into 6 equal pieces. Each piece is \(\frac{1}{6}\) of the whole. His son Alex ate 2 pieces, so \(\frac{4}{6}\) of the pan of brownies is left.

Write \(\frac{4}{6}\) as a sum of unit fractions.
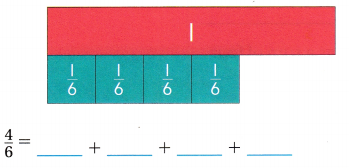
Answer:
\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)
Explanation:
Share and Show
Math talk
Mathematical Processes
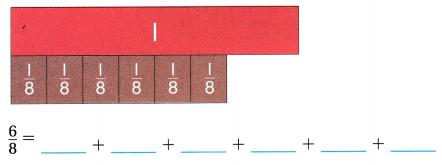
Explain how the numerator \(\frac{6}{8}\) is related to the number of unit fractions in the sum.
Answer:
\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\).
Question 1.
Write \(\frac{1}{6}\) as a sum of unit fractions.
Answer:
\(\frac{1}{3}\)+\(\frac{1}{3}\)+\(\frac{1}{3}\)+\(\frac{1}{3}\)+\(\frac{1}{3}\)+\(\frac{2}{3}\)
Explanation:
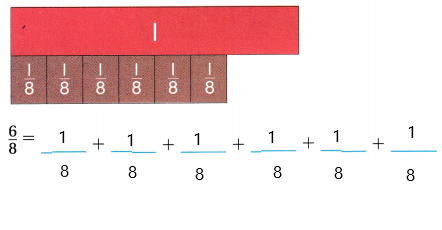
The sum of unit fractions is \(\frac{1}{3}\)+\(\frac{1}{3}\)+\(\frac{1}{3}\)+\(\frac{1}{3}\)+\(\frac{1}{3}\)+\(\frac{1}{3}\) = \(\frac{6}{8}\)
Question 2.
\(\frac{2}{3}\) = _____
Answer:
\(\frac{1}{3}\)+\(\frac{1}{3}\)
Explanation:
The sum of unit fractions for the given question is \(\frac{1}{3}\)+\(\frac{1}{3}\).
Question 3.
\(\frac{3}{6}\) = ______
Answer:
\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)
Explanation:
The sum of unit fractions for the given question is \(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\).
Write the fraction represented by the sum of unit fractions.
Question 4.
\(\frac{1}{4}\) + \(\frac{1}{4}\) = _____
Answer:
\(\frac{2}{4}\)
Explanation:
The sum of unit fractions for the given question is \(\frac{2}{4}\).
Question 5.
\(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) = ____
Answer:
\(\frac{5}{8}\)
Explanation:
The sum of unit fractions for the given question is \(\frac{5}{8}\).
Problem Solving
Question 6.
Mrs. Collins cut a piece of yarn into 8 equal pieces. She used 1 piece for a craft project, so \(\frac{7}{8}\) is left. How can you write \(\frac{7}{8}\) as a sum of unit fractions?
Answer:
\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\)
Explanation:
The sum of unit fractions for the given question is \(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\)+\(\frac{1}{8}\).
Question 7.
H.O.T. Can you write the number 1 as a sum of four-unit fractions? Explain.
Answer:
\(\frac{1}{2}\)+\(\frac{1}{2}\)+\(\frac{1}{2}\)+\(\frac{1}{2}\).
Explanation:
I have written the number 1 as a sum of four-unit fractions is \(\frac{1}{2}\)+\(\frac{1}{2}\)+\(\frac{1}{2}\)+\(\frac{1}{2}\).
Problem Solving
H.O.T. Pose a Problem
Question 8.
Taylor made a loaf of pumpkin bread and cut it into 8 equal pieces.
Look back at the carrot cake example on page 61. Write a similar problem that can be solved by using the picture of Taylor’s pumpkin loaf shown at the right. Then solve the problem.

Answer:
Question 9.
Multi-Step Write a similar problem by changing the number of equal slices in the loaf of pumpkin bread. Then solve the problem.
Answer:
Daily Assessment Task
Fill in the bubble for the correct answer choice.
Question 10.
Mrs. Davis made a large pot of soup for dinner. In all, she and her family ate \(\frac{3}{4}\) of the soup. Which is \(\frac{3}{4}\) written as a sum of unit fractions?
(A) \(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\)
(B) \(\frac{3}{1}\) + \(\frac{3}{1}\) + \(\frac{3}{1}\) + \(\frac{3}{1}\)
(C) \(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\)
(D) \(\frac{3}{4}\) + \(\frac{3}{4}\) + \(\frac{3}{4}\)
Answer:
\(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\)
Explanation:
The \(\frac{3}{4}\) written as a sum of unit fractions is \(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\).
Question 11.
Representations What fraction is represented by the sum \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\)?
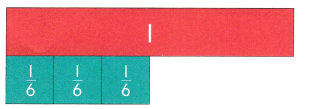
(A) \(\frac{2}{6}\)
(B)\(\frac{3}{6}\)
(C) \(\frac{4}{6}\)
(D) \(\frac{6}{6}\)
Answer:
\(\frac{3}{6}\)
Explanation:
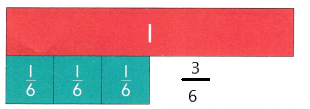
The fraction that is represented by the sum is \(\frac{3}{6}\).
Question 12.
Multi-Step Penny cut a banana into eight equal pieces. She ate six pieces. Which is equal to the fraction of the banana Penny did not eat?
(A) \(\frac{2}{8}\) + \(\frac{2}{8}\)
(B) \(\frac{1}{8}\) + \(\frac{2}{8}\)
(C) \(\frac{1}{8}\) + \(\frac{1}{8}\)
(D) \(\frac{1}{2}\) + \(\frac{1}{2}\)
Answer:
\(\frac{1}{8}\) + \(\frac{1}{8}\)
Explanation:
\(\frac{1}{8}\) + \(\frac{1}{8}\) is equal to the fraction of the banana Penny did not eat.
Texas Test Prep
Question 13.
Which is \(\frac{3}{8}\) written as a sum of unit fractions?
(A) \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\)
(B) \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\)
(C) \(\frac{1}{8}\) + \(\frac{1}{8}\)
(D) \(\frac{3}{8}\) + \(\frac{3}{8}\) + \(\frac{3}{8}\)
Answer:
\(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\)
Explanation:
The \(\frac{3}{8}\) written as a sum of unit fractions is \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\).
Texas Go Math Grade 3 Lesson 2.5 Homework and Practice Answer Key
Write the fraction as a sum of unit fractions.
Question 1.
\(\frac{4}{6}\)
Answer:
\(\frac{2}{6}\) + \(\frac{2}{6}\)
Explanation:
The sum of unit fractions is \(\frac{2}{6}\) + \(\frac{2}{6}\)=\(\frac{4}{6}\)
Question 2.
\(\frac{5}{8}\)
Answer:
\(\frac{1}{8}\) + \(\frac{2}{8}\) + \(\frac{2}{8}\)
Explanation:
The sum of unit fractions is \(\frac{1}{8}\) + \(\frac{2}{8}\) + \(\frac{2}{8}\)=\(\frac{5}{8}\)
Question 3.
\(\frac{2}{3}\)
Answer:
\(\frac{1}{3}\) + \(\frac{1}{3}\)
Explanation:
The sum of unit fractions is \(\frac{1}{3}\) + \(\frac{1}{3}\)=\(\frac{2}{3}\)
Question 4.
\(\frac{6}{6}\)
Answer:
\(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\)+\(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\).
Explanation:
The sum of unit fraction is \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\)+\(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\).
Write the fraction as a sum of unit fractions.
Question 5.
\(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\)
Answer:
\(\frac{3}{4}\)
Explanation:
The sum of unit fraction for the given question is \(\frac{3}{4}\).
Question 6.
\(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\)
Answer:
\(\frac{3}{8}\)
Write the fraction represented by the sum of unit fractions.
Question 5.
\(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\)
Answer:
\(\frac{3}{4}\)
Explanation:
The sum of unit fractions for the given fraction is \(\frac{3}{4}\).
Question 6.
\(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\)
Answer:
\(\frac{3}{8}\)
Explanation:
The sum of unit fractions for the given fraction is \(\frac{3}{8}\).
Problem Solving
Question 7.
Meg uses \(\frac{1}{4}\) stick of butter to make breakfast for the family. That means that there is \(\frac{3}{4}\) stick of butter left. How can you write \(\frac{3}{4}\) as the sum of unit fractions?
Answer:
\(\frac{3}{8}\)+\(\frac{3}{8}\)+\(\frac{3}{8}\)
Question 8.
Mr. Ayer cuts one melon into 8 equal pieces. Write the number 1 as a sum of unit fractions that name each piece of melon.
Answer:
\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)
Explanation:
Mr. Ayer cuts melon into 8 equal pieces. So to write 1 as a sum ofunit fractions that name each piece of melon is \(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\)+\(\frac{1}{6}\).
Texas Test Prep
Lesson Check
Fill in the bubble completely to show your answer.
Question 9.
What is \(\frac{5}{6}\) written as the sum of unit fractions?
(A) \(\frac{5}{6}\) + \(\frac{5}{6}\) + \(\frac{5}{6}\) + \(\frac{5}{6}\) + \(\frac{5}{6}\)
(B) \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\)
(C) \(\frac{1}{6}\)
(D) \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\)
Answer:
\(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\)
Explanation:
The \(\frac{5}{6}\) written as the sum of unit fractions is \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\).
Question 10.
Ross walks mile \(\frac{3}{8}\) to school. What is \(\frac{3}{8}\) written as the sum of unit fractions?
(A) \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\)
(B) \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\)
(C) \(\frac{1}{8}\)
(D) \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\)
Answer:
\(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\)
Explanation:
The \(\frac{3}{8}\) written as the sum of unit fractions is \(\frac{1}{8}\) + \(\frac{1}{8}\) + \(\frac{1}{8}\).
Question 11.
What fraction is represented by the sum \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\)?
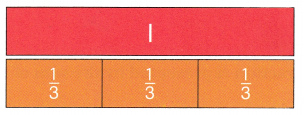
(A) \(\frac{0}{3}\)
(B) \(\frac{3}{3}\)
(C) \(\frac{1}{3}\)
(D) \(\frac{2}{3}\)
Answer:
\(\frac{3}{3}\)
Explanation:
The sum of \(\frac{1}{3}\) + \(\frac{1}{3}\) + \(\frac{1}{3}\) is \(\frac{3}{3}\).
Question 12.
Multi-Step Jamal sliced an orange into four equal pieces. He ate one slice and gave the rest to his friends. Which is equal to the fraction of the orange that Jamal gave to his friends?
(A) \(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\)
(B) \(\frac{1}{4}\) + \(\frac{1}{4}\)
(C) \(\frac{1}{4}\)
(D) \(\frac{4}{1}\) + \(\frac{4}{1}\) + \(\frac{4}{1}\)
Answer:
\(\frac{1}{4}\) + \(\frac{1}{4}\) + \(\frac{1}{4}\)
Explanation:
Jamal sliced orange into four equal pieces and ate one slice and gave the rest to his friends. Hence Jamal gave to his friends \(\frac{3}{4}\) fraction of the orange.
Question 13.
Multi-Step A bookshelf is divided into six equal cubbies. Two of the cubbies are filled with DVDs. The rest are empty. Which is equal to the fraction of the empty cubbies?
(A) \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\)
(B) \(\frac{1}{6}\) + \(\frac{1}{6}\)
(C) \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\) + \(\frac{1}{6}\)
(D) \(\frac{1}{6}\)
Answer:
\(\frac{1}{6}\) + \(\frac{1}{6}\)
Explanation:
A bookshelf has six equal cubbies and filled two of the cubbies with DVDs.
The fraction of the empty cubbies is \(\frac{2}{6}\).