Guided Practice – Page No. 268
Find the circumference of each circle.
Question 1.
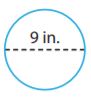
________ in
Answer: 56.57 in
Explanation:
Circumference of the circle = 2πr = 2 x 22/7 x 9 = 56.57 in
Question 2.
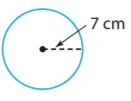
________ cm
Answer: 44 cm
Explanation:
Circumference of the circle = 2πr = 2 x 22/7 x 7 = 44 cm
Find the circumference of each circle. Use 3.14 or \(\frac{22}{7}\) for π. Round to the nearest hundredth, if necessary.
Question 3.
______ m
Question 4.
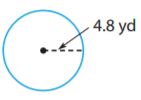
______ yd
Answer: 30.15 yd
Explanation:
Circumference of the circle = 2πr = 2 x 3.14 x 4.8 = 30.144 yd
Question 5.
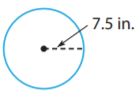
______ in
Answer: 7.5 in
Explanation:
Circumference of the circle = 2πr = 2 x 3.14 x 7.5 = 47.1 in
Question 6.
A round swimming pool has a circumference of 66 feet. Carlos wants to buy a rope to put across the diameter of the pool. The rope costs $0.45 per foot, and Carlos needs 4 feet more than the diameter of the pool. How much will Carlos pay for the rope?
$ ______
Answer: $6.525
Explanation:
Circumference of the swimming pool = 66 feet
πd = 66
22/7 x d = 66
d = 66 x 7/ 22 = 10.5
The diameter of the pool = 10.5 feet
Carlos needs 4 feet more than the diameter of the pool.
Total rope needed = 10.5 + 4 = 14.5 feet
Cost of rope per foot = $0.45
Total cost of the rope = 14.5 x $0.45 = $6.525
Therefore the total cost of the rope = $6.525
Find each missing measurement to the nearest hundredth. Use 3.14 for π.
Question 7.
r =
d =
C = π yd
r = ________ yd
d = ________ yd
Answer:
r = 0.5 yd
d = 1 yd
Explanation:
Circumference = π yd
2πr = π yd
r = 1/2 yd = 0.5 yd
d = 2r = 2 [1/2] = 1 yd
Question 8.
r ≈
d ≈
C = 78.8 ft
r ≈ ________ ft
d ≈ ________ ft
Answer:
r = 495.31 ft
d = 990.62 ft
Explanation:
Circumference = 78.8 ft
2πr = 78.8 ft
r = 2 x 22/7 x 78.8 = 495.31 ft
d = 2 x 495.31 = 990.62 ft
Question 9.
r ≈
d ≈ 3.4 in
C =
r ≈ ________ in
C = ________ in
Answer:
r = 1.7 in
c = 10.68 in
Explanation:
Diameter = 3.4 in
Circumference = πd = 22/7 x 3.4 in = 10.68 in
r = d/2 = 1.7 in
Essential Question Check-In
Question 10.
Norah knows that the diameter of a circle is 13 meters. How would you tell her to find the circumference?
Type below:
____________
Answer: Circumference = 16.82 meters
Explanation:
Given,
Diameter = 13 meters
Circumference = πd = 22/7 x 13 = 16.82 meters
Independent Practice – Page No. 269
For 11–13, find the circumference of each circle. Use 3.14 or \(\frac{22}{7}\) for π. Round to the nearest hundredth, if necessary.
Question 11.
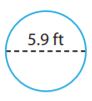
_______ ft
Answer:
Cicumference = 18.526 ft = 19 ft (approx)
Explanation:
Given:
Diameter = 5.9 ft
Cicumference = πd = 3.14 x 5.9 = 18.526 ft = 19 ft (approx)
Question 12.
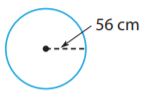
_______ cm
Answer:
Cicumference =176 cm
Explanation:
Given:
Radius = 56 cm
Cicumference = πd = 22/7 x 56 = 176 cm
Question 13.

_______ in
Answer:
Cicumference = 110 in
Explanation:
Given:
Diameter = 35 in
Cicumference = πd = 22/7 x 35 = 110 in
Question 14.
In Exercises 11–13, for which problems did you use \(\frac{22}{7}\) for π? Explain your choice.
Type below:
_____________
Answer:
11th question as 3.14 and the 12 and 13 questions as π
Explanation:
We can take 3.14 as π for 11 th question because the diameter is given in decimal points.
And in questions 12 and 13 we need to take π because the radius and diameter are given in whole number form.
Question 15.
A circular fountain has a radius of 9.4 feet. Find its diameter and circumference to the nearest tenth.
d = _________ ft
C = _________ ft
Answer:
d = 19 ft
C = 59 ft
Explanation:
Given:
Radius = 9.4 ft
Diameter = 2r = 2 x 9.4 = 18.8 ft = 19 ft (approx)
Circumference = πd = 22/7 x 18.8 = 59.08 = 59 ft (approx)
Question 16.
Find the radius and circumference of a CD with a diameter of 4.75 inches.
r = _________ in
C = _________ in
Answer:
r = 2.4 in
C = 15 in
Explanation:
Given:
Diameter = 4.75 in
Radius = r/2 = 4.75/2 = 2.37 in = 2.4 in (approx)
Circumference = πd = 22/7 x 4.75 = 14.92 in =15 in (approx)
Question 17.
A dartboard has a diameter of 18 inches. What are its radius and circumference?
r = _________ in
C = _________ in
Answer:
r = 9 in
C = 56.6 in
Explanation:
Given:
Diameter = 18 in
Radius = r/2 = 18/2 = 9 in
Circumference = πd = 22/7 x 18 = 56.57 in = 56.6 in (approx)
Question 18.
Multistep
Randy’s circular garden has a radius of 1.5 feet. He wants to enclose the garden with edging that costs $0.75 per foot. About how much will the edging cost? Explain.
$ _______
Answer:
Explanation:
Given:
The radius of the garden= 1.5 ft
Circumference of the garden = 2πr = 2 x 22/7 x 1.5 = 9.42 ft
Cost of enclosing the garden per foot = $0.75
Total cost of edging = 9.42 x $0.75 = $7.06 = $7 (approx)
Question 19.
Represent Real-World Problems
The Ferris wheel shown makes 12 revolutions per ride. How far would someone travel during one ride?

_______ ft
Answer: Total distance travelled in one ride is 4,752 ft
Explanation:
Given:
The diameter of the Ferris wheel= 63 ft
Circumference of the Ferris wheel = 2πr = 2 x 22/7 x 63 = 396 ft
Total number of revolutions = 12
Total distance travelled = 12 x 396 = 4,752 ft
Question 20.
The diameter of a bicycle wheel is 2 feet. About how many revolutions does the wheel make to travel 2 kilometres? Explain. Hint: 1 km ≈ 3,280 ft
_______ revolutions
Answer:
1044 revolutions
Explanation:
Given:
Diametre of the bicycle wheel = 2 feet
Total distance travelled = 2 kilometres
We know that,
1 km ≈ 3,280 ft
2 km = 2 x 3,280 = 6,560 ft
Circumference of the bicycle = Distance travelled in one revolution = πd = 22/7 x 2 = 6.28 ft = 6.3 ft
Total number of revolutions = Total distance travelled / distance travelled in one revolution
= 6560 / 6.28 = 1044 revolutions
Question 21.
Multistep
A map of a public park shows a circular pond. There is a bridge along a diameter of the pond that is 0.25 mi long. You walk across the bridge, while your friend walks halfway around the pond to meet you at the other side of the bridge. How much farther does your friend walk?
_______ mi
Answer:
Explanation:
Given,
The diameter of the pond = 0.25 mi
The length of the bridge = The diameter of the pond = 0.25 mi
Then the distance walked by the man = 0.25 mi
Distance travelled by the friend = Halfway around the pond to meet you at the other side of the bridge = πd/2
= 22/7 x 0.25/2 = 0.39 = 0.4 mi
The friend travelled more distance compared to the man
The more distance travelled by the friend = 0.39 – 0.25 = 0.14 mi
Page No. 270
Question 22.
Architecture
The Capitol Rotunda connects the House and the Senate sides of the U.S. Capitol. Complete the table. Round your answers to the nearest foot.
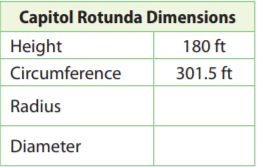
Type below:
_____________
Answer:
Radius = 48 ft
Diameter = 96 ft
Explanation:
Given
Height = 180 ft
Circumference = 301.5 ft
πd = 301.5
22/7 x d = 301.5
d = 95.93 = 96 ft
r = d/2 = 96/2 = 48 ft
H.O.T.
Focus on Higher Order Thinking
Question 23.
Multistep
A museum groundskeeper is creating a semicircular statuary garden with a diameter of 30 feet. There will be a fence around the garden. The fencing costs $9.25 per linear foot. About how much will the fencing cost altogether?
$ _______
Answer:
The total cost of fencing = $712
Explanation:
Given,
The diameter = 30 ft
Circumference of the garden in the shape of circle = 2πr
Circumference of the semicircle = πr = πd/2 = 22/7 x 30/2 = 47.14ft
Cost of fencing for each foot = $9.25
The total cost of fencing the semicircular garden = 47.14 x $9.25 + 30 x $9.25 = $712 (approx)
Question 24.
Critical Thinking
Sam is placing rope lights around the edge of a circular patio with a diameter of 18 feet. The lights come in lengths of 54 inches. How many strands of lights does he need to surround the patio edge?
_______ strands
Answer: 12 and a half strands of light = 13 strands (approx)
Explanation:
Given,
The diameter of the circular patio = 18 ft = 216 inch
Circumference of the circular patio = πd = 22/7 x 216 = 678.85 inch
The lights will come in a length (in one strand)= 54 inches
Total number of strands of light required for the circular patio
= Circumference of the circular patio/ The lights will come in a length (in one strand) = 678.85/54 = 12.57 = 12 and a half strands of light
Question 25.
Represent Real-World Problems
A circular path 2 feet wide has an inner diameter of 150 feet. How much farther is it around the outer edge of the path than around the inner edge?
_______ feet
Answer: about 12.6 ft
Explanation:
Given,
Width of the circular path = 2 ft
The inner diameter of the circular path = 150 ft
The outer diameter of the circular path = 150 + 2(2) = 154 ft
Inner circumference = πd = 150 π
Outer circumference = πd = 154π
Distance between the outer and inner edge = 154 π – 150 π = 4 π = 12.6 ft
Question 26.
Critique Reasoning
Gear on a bicycle has the shape of a circle. One gear has a diameter of 4 inches, and a smaller one has a diameter of 2 inches. Justin says that the circumference of the larger gear is 2 inches more than the circumference of the smaller gear. Do you agree? Explain your answer.
_______
Answer:
Justin statement is incorrect.
Explanation:
The circumference of the larger gear = πd = 4π
The circumference of the smaller gear = πd = 2π
Since, 2 x 2π = 4π, the circumference of the larger gear is two times the circumference of the smaller gear.
Since = 4π – 2π = 2π = 6.28
Therefore, The larger circumference is not 2 inches more than the smaller circumference
Question 27.
Persevere in Problem Solving
Consider two circular swimming pools. Pool A has a radius of 12 feet, and Pool B has a diameter of 7.5 meters. Which pool has a greater circumference? How much greater? Justify your answers.
_______
Answer:
Pool B about 0.9 meters
Explanation:
Given,
Pool A has a diameter = 24 ft
Pool B has a diameter = 7.5 m
We know that,
1 ft = 0.3 metres
24 ft = 7.2 metres
The pool B has a greater diameter so it has a greater circumference.
Circumference of the pool A = 7.2π
Circumference of the pool B = 7.5π
Difference between the circumferences = 7.5π – 7.2π = 0.9 meters.
Guided Practice – Page No. 274
Find the area of each circle. Round to the nearest tenth if necessary. Use 3.14 for π.
Question 1.
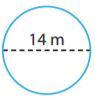
_______ m2
Answer: 153.9 m2
Explanation:
Given:
Diameter = 14 m
Radius = 14/2 = 7 m
Area of the circle = πr2
= 3.14 x 7 x 7 = 153.86 = 153.9 m2
Question 2.
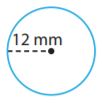
_______ mm2
Answer: 452.2 mm2
Explanation:
Given:
Radius =12mm
Area of the circle = πr2
= 3.14 x 12 x 12 = 3.14(144) = 452.2mm2
Question 3.
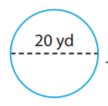
_______ yd2
Answer: 314 yd2
Explanation:
Given:
Diameter = 20yd
Radius = 20/2 = 10yd
Area of the circle = πr2
= 3.14 x 10 x 10 = 3.14(100) = 314yd2
Solve. Use 3.14 for π.
Question 4.
A clock face has a radius of 8 inches. What is the area of the clock face? Round your answer to the nearest hundredth.
_______ in2
Answer: 200.96 in2
Explanation:
Given:
Radius = 8inches
Area of the clock face = πr2
= 3.14 x 8 x 8= 3.14(64) = 200.96 in2
Question 5.
A DVD has a diameter of 12 centimeters. What is the area of the DVD? Round your answer to the nearest hundredth.
_______ cm2
Answer: 113.04 cm2
Explanation:
Given:
Diameter = 12 centimeters
Radius = 12/2 = 6 centimeters
Area of the DVD= πr2
= 3.14 x 6 x 6 = 3.14(36) = 113.04 cm2
Question 6.
A company makes steel lids that have a diameter of 13 inches. What is the area of each lid? Round your answer to the nearest hundredth.
_______ in2
Answer: 132.67 in2
Explanation:
Given:
Diameter = 13 inches
Radius = 13/2 = 6.5 inches
Area of each lid= πr2
= 3.14 x 6.5 x 6.5 = 3.14(42.25) = 132.67 in2
Find the area of each circle. Give your answers in terms of π.
Question 7.
C = 4π
A =
Type below:
______________
Answer: 4π
Explanation:
Given:
Circumcenter = 4π
2πr = 4π
Radius = 4/2 = 2 units
Area of the circle = πr2
= π x 2 x 2 = π(4) = 4π square units
Question 8.
C = 12π
A =
Type below:
______________
Answer: 36π
Explanation:
Given:
Circumcenter = 12π
2πr = 12π
Radius =6 units
Area of the circle = πr2
= π x 6 x 6 = π(36) = 36π square units
Question 9.
C = \(\frac{π}{2}\)
A =
Type below:
______________
Answer: π/16
Explanation:
Given:
Circumcenter = \(\frac{π}{2}\)
2πr = \(\frac{π}{2}\)
Radius = 1/4 units
Area of the circle = πr2
= π x 1/4 x 1/4 = π(1/16) = π/16 square units
Question 10.
A circular pen has an area of 64π square yards. What is the circumference of the pen? Give your answer in terms of π
Type below:
______________
Answer: 16π
Explanation:
Given:
Area of the circular pen = 64π square yards
πr2 = 64π
r = 8 yards
Circumference of the circle = 2πr = 2 x 8 x π = 16π yards
Essential Question Check-In
Question 11.
What is the formula for the area A of a circle in terms of the radius r?
Type below:
______________
Answer: πr2
Explanation:
Area of a circle = πr2
Independent Practice – Page No. 275
Question 12.
The most popular pizza at Pavone’s Pizza is the 10-inch personal pizza with one topping. What is the area of a pizza with a diameter of 10 inches? Round your answer to the nearest hundredth.
_______ in2
Answer: 78.5 in2
Explanation:
Given:
Diameter = 10 inches
Radius = 10/2 = 5 inches
Area of a pizza = πr2
= 3.14 x 5 x 5 = 3.14(25) = 78.5 in2
Question 13.
A hubcap has a radius of 16 centimeters. What is the area of the hubcap? Round your answer to the nearest hundredth.
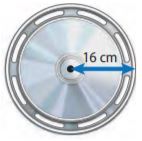
_______ cm2
Answer: 803.84 cm2
Explanation:
Given:
Radius = 16 cm
Area of the circle = πr2
= 3.14 x 16 x 16 = 3.14(256) = 803.84 cm2
Question 14.
A stained glass window is shaped like a semicircle. The bottom edge of the window is 36 inches long. What is the area of the stained glass window? Round your answer to the nearest hundredth.
_______ in2
Answer: 508.68 in2
Explanation:
Area of the semicircle = 1/2 πr2 = 1/2(3.14)(18)(18) = 1/2 (3.14)(324) = 1.57(324) = 508.68 in 2
Question 15.
Analyze Relationships
The point (3,0) lies on a circle with the centre at the origin. What is the area of the circle to the nearest hundredth?
_______ units2
Answer: 28.26 units2
Explanation:
Radius = 3
Area of the circle = πr2 = π(3)2 = 3.14(9) = 28.26 units2
Question 16.
Multistep
A radio station broadcasts a signal over an area with a radius of 50 miles. The station can relay the signal and broadcast over an area with a radius of 75 miles. How much greater is the area of the broadcast region when the signal is relayed? Round your answer to the nearest square mile.
_______ mi2
Answer: 9813 mi2
Explanation:
Given:
The radius of a radio station broadcasted the signal (r) = 50 miles
The greatest radius to which the broadcast can be relayed (R) = 75 miles
The greatest area of the broadcast region when the signal is relayed = πR2-πr2 = π(75) (75) – π (50) (50)
= 5625π – 2500π
= 3125π
= 3125(3.14) = 9813 mi2(approx)
Question 17.
Multistep
The sides of a square field are 12 meters. A sprinkler in the center of the field sprays a circular area with a diameter that corresponds to a side of the field. How much of the field is not reached by the sprinkler? Round your answer to the nearest hundredth.
_______ m2
Answer:30.96 m2
Explanation:
Given:
The side of the square = 12 meters
The diameter circular area of the field in the centre = The side of the square = 12 meters
The radius of the field = 12/2 = 6 meters
Area of the field which is not reached by the sprinkler = Area of the square – Area of the circular area
= (side)2-πr2 = (12)(12) – π (6) (6)
= 144 – 36 (3.14)
= 144 – 113.04
= 30.96 m2
Question 18.
Justify Reasoning
A small silver dollar pancake served at a restaurant has a circumference of 2π inches. A regular pancake has a circumference of 4π inches. Is the area of the regular pancake twice the area of the silver dollar pancake? Explain.
_______
Answer: No, the area of the regular pancake is 4 times the area of the silver dollar pancake
Explanation:
Silver Dollar pancake:
Circumference of the silver Dollar pancake = 2π inches
2πr = 2π
r = 1 inch
Area of the silver dollar pancake = πr2 = π (1) (1) = π in2
Regular pancake:
Circumference of the regular pancake = 4π inches
2πr = 4π
r = 2 inch
Area of the silver dollar pancake = πr2 = π (2) (2) = 4π in2
Therefore, the area of the regular pancake is 4 times the area of the silver dollar pancake
Question 19.
Analyze Relationships
A bakery offers a small circular cake with a diameter of 8 inches. It also offers a large circular cake with a diameter of 24 inches. Does the top of the large cake have three times the area of that of the small cake? If not, how much greater is its area? Explain.
_______
Answer: No, the area of the large cake is 9 times the area of the small cake
Explanation:
Small Cake:
The diameter of the small cake= 8 inches
The radius of the small cake = 8/2 = 4 inches
Area of the small cake = πr2 = π (4) (4) = 16 π in2
Large Cake:
The diameter of the large cake= 24 inches
The radius of the large cake = 24/2 = 12 inches
Area of the large cake = πr2 = π (12) (12) = 144 π in2
Since 144 π/ 16 π = 9
Therefore the
area of the large cake is 9 times the area of the small cake.
Page No. 276
Question 20.
Communicate Mathematical Ideas
You can use the formula A = \(\frac{C^{2}}{4π}\) to find the area of a circle given the circumference. Describe another way to find the area of a circle when given the circumference.
Type below:
____________
Answer: Area = C2/4π
Explanation:
Circumference of the circle = 2πr
C = 2πr
Divide both sides by 2π
then, r = C/2π
Area of the circle = πr2
Substitute C/2π for r:
Area = π(c/2π)2 = C2/4π
Question 21.
Draw Conclusions
Mark wants to order a pizza. Which is the better deal? Explain.
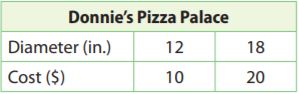
_____
Answer: The pizza of 18 inches is a better deal
Explanation:
Given:
The diameter of the pizza = 12 inches
The radius of the pizza = 12/2= 6 inches
Area of the circle = πr2
= (3.14)(6)(6) = 113 (approx) in2
The total cost of the pizza = $10
Cost of the pizza per inch = $10/113 = $0.09 per square inch
The diameter of the pizza = 18 inches
The radius of the pizza = 18/2= 9 inches
Area of the circle = πr2
= (3.14)(9)(9) = 254 (approx) in2
The total cost of the pizza = $20
Cost of the pizza per inch = $20/254 = $0.08 per inch
Question 22.
Multistep
A bear was seen near a campground. Searchers were dispatched to the region to find the bear.
a. Assume the bear can walk in any direction at a rate of 2 miles per hour. Suppose the bear was last seen 4 hours ago. How large an area must the searchers cover? Use 3.14 for π. Round your answer to the nearest square mile.
_____ mi2
Answer: 201mi2
Explanation:
The bear can walk a distance = 2 x 4 = 8 miles
Since it is walking 2 miles per hour for 4 hours
The radius of the bear = 8 miles
Area of the circle = πr2
= (3.14)(8)(8) = 201 (approx) mi2
Question 22.
b. What If? How much additional area would the searchers have to cover if the bear were last seen 5 hours ago?
_____ mi2
Answer: 113mi2
Explanation:
If the bear for 5 hours then,
The bear can walk a distance = 2 x 5 = 10 miles
Since it is walking 2 miles per hour for 5 hours
The radius of the bear = 10 miles
Area of the circle = πr2
= (3.14)(10)(10) = 314 (approx) mi2
The additional area covered by the searches = 314 – 201 = 113 mi2
H.O.T.
Focus on Higher Order Thinking
Question 23.
Analyze Relationships
Two circles have the same radius. Is the combined area of the two circles the same as the area of a circle with twice the radius? Explain.
_____
Answer: No
Explanation:
If the radius of two circles is the same.
then, Let the radii of the circles be 1.
The area of each circle = π square units
The combined area of 2 circles =π+π = 2π square units
If the radius is doubled.
then, Let the radii of the circles be 2
The area of each circle = 4π square units
The combined area of 2 circles = 4π+4π = 8π square units
Therefore the areas of both cases are not the same.
Question 24.
Look for a Pattern
How does the area of a circle change if the radius is multiplied by a factor of n, where n is a whole number?
Type below:
____________
Answer: The new area is then n2 times the area of the original circle.
Explanation:
If the radius is multiplied by a factor “n”
then, the new radius = rn
The area of the circle (with radius rn) = π(rn)2 = n2 (πr2).
Therefore the new area is n2 times the area of the original circle.
Question 25.
Represent Real World Problems
The bull’s-eye on a target has a diameter of 3 inches. The whole target has a diameter of 15 inches. What part of the whole target is the bull’s-eye? Explain.
Type below:
____________
Answer: 1/25 of the target
Explanation:
Bull’s eye:
Diameter of Bull’s eye = 3 inches
Radius of Bull’s eye = 3/2 = 1.5 inches
Area of the Bull’s eye = π(r)2 = π(1.5)2 = 2.25π
Target:
Diameter of the target = 15 inches
Radius of the target = 15/2 = 7.5 inches
Area of the target = π(r)2 = π(7.5)2 = 56.25π
The part of Bull’s eye in the whole target = 2.25π/ 56.25π = 1/25
Therefore the 1/25th part of the whole target is the Bull’s eye.
Guided Practice – Page No. 280
Question 1.
A tile installer plots an irregular shape on grid paper. Each square on the grid represents 1 square centimeter. What is the area of the irregular shape?

_____ cm2
Answer: Area of the irregular shape = 34 cm2
Explanation:
STEP1 First divide the irregular shapes into polygons.
STEP2 The irregular shape can be divided into a triangle, rectangle, parallelogram
STEP3 Areas of the polygons
Area of triangle = 1/2 (base x height) = 1/2 (4 x 2) = 4 cm2
Area of the rectangle = length x breadth = 5 x 3 = 15 cm2
Area of the parallelogram = base x height = 5 x 3 = 15 cm2
Area of the irregular shape = (15+15+5) cm2= 34cm2
Question 2.
Show two different ways to divide the composite figure. Find the area both ways. Show your work below.
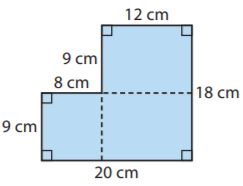
_____ cm2
Answer: Area of the figure in both ways = 288 cm2
Explanation:
The first way to divide up the composite shape is to divide it into an 8 by 9 rectangle and a 12 by 18 rectangle.
The area of the first rectangle = Length x breadth = 9 x 8 = 72 cm2
The area of the second rectangle = Length x breadth = 18 x 12 = 216 cm2
The total area of the figure = 72 + 216 = 288 cm2
Question 3.
Sal is tiling his entryway. The floor plan is drawn on a unit grid. Each unit length represents 1 foot. Tile costs $2.25 per square foot. How much will Sal pay to tile his entryway?

$ _____
Answer: Sal will pay $97.875
Explanation:
Separate this figure into trapezium and parallelogram.
Area of the trapezium = 1/2 (a+b)h = 1/2 (7+4) 5 = 1/2 (11) 5 = 27.5 ft2
Area of the parallelogram = base x height = 4 x 4 = 16 ft2
The total area of the figure = 27.5 + 16 = 43.5ft2
Cost of each square foot = $2.25
Amount paid by Sal = 43.5 x 2.25 = $97.875
Essential Question Check-In
Question 4.
What is the first step in finding the area of a composite figure?
Type below:
______________
Answer:
The first step in finding the area of a composite figure is to divide it up into smaller basic shapes.
Explanation:
The first step in finding the area of a composite figure is to divide it up into smaller basic shapes such as triangles, squares, rectangles, parallelograms, circles and trapezium.
Then calculate the area of each figure and add them to find the area of the figure.
Independent Practice – Page No. 281
Question 5.
A banner is made of a square and a semicircle. The square has side lengths of 26 inches. One side of the square is also the diameter of the semicircle. What is the total area of the banner? Use 3.14 for π.
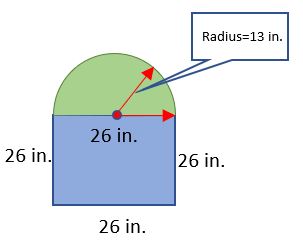
_____ in2
Answer: 941.33 in2
Explanation:
Area of the square = side x side = 26 x 26 = 676 in2
Area of the semicircle =1/2 πr2= 1/2 (3.14) (13) (13) = 1/2 (3.14) (169) = 265.33 in2
Area of the figure = 676 + 265.33 = 941.33 in2
Question 6.
Multistep
Erin wants to carpet the floor of her closet. A floor plan of the closet is shown.
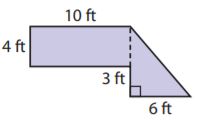
a. How much carpet does Erin need?
_____ ft2
Answer: 61 ft2
Explanation:
Area of the rectangle = length x breadth = 4 x 10 = 40 ft
Area of the triangle = 1/2 x base x height = 1/2 x 6 x 7 = 21 ft
The total area of the figure = 40+21 = 61 ft2
Question 6.
b. The carpet Erin has chosen costs $2.50 per square foot. How much will it cost her to carpet the floor?
$ _____
Answer: $152.50
Explanation:
Cost per square foot of the carpet = $2.50
The total cost of the carpet on the floor = 61 x $2.50 =$152.50
Question 7.
Multiple Representations
Hexagon ABCDEF has vertices A(-2, 4), B(0, 4), C(2, 1), D(5, 1), E(5, -2), and F(-2, -2). Sketch the figure on a coordinate plane. What is the area of the hexagon?
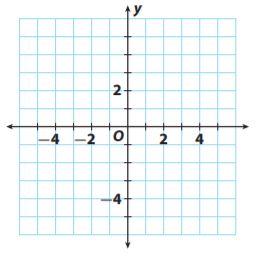
_____ units2
Answer: The area of the figure is 30 square units
Explanation:
Separate the figure into a trapezium and a rectangle.
Area of a trapezium = 1/2 (a+b) h= 1/2 (2+4) x 3 = 1/2 (6) 3 = 9 square units
Area of a rectangle = length x breadth = 7 x 3 = 21 square units
The total area of the figure = 9+21 = 30 square units
Question 8.
A field is shaped like the figure shown. What is the area of the field? Use 3.14 for π.
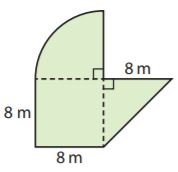
_____ m2
Answer: 146.24 m2
Explanation:
Divide the figure into a square, triangle and a quarter of a circle.
Area of a square = side x side = 8 x 8 = 64 m2
Area of a quarter of a circle = 1/4 (πr2) = 1/4 (3.14 x 82)
= 1/4 (200.96) = 50.24 m2
Area of the triangle = 1/2 x base x height = 1/2 x 8 x 8 = 32 m2
Total area of the figure = 64+32+50.24 = 146.24 m2
Question 9.
A bookmark is shaped like a rectangle with a semicircle attached at both ends. The rectangle is 12 cm long and 4 cm wide. The diameter of each semicircle is the width of the rectangle. What is the area of the bookmark? Use 3.14 for π.
_____ cm2
Answer: 60.56 cm2
Explanation:
The bookmark is divided into a rectangle, semicircle.
Area of the rectangle = length x breadth = 12 x 4 = 48 cm2
The diameter of the semicircle = The width of the rectangle = 4 cm
The radius of the semicircle = 4/2 = 2 cm
The area of the semicircle = πr2 = 3.14 x 2 x 2 = 12.56 cm2
The total area of the bookmark = 12.56 + 48 = 60.56 cm2
Question 10.
Multistep
Alex is making 12 pendants for the school fair. The pattern he is using to make the pennants is shown in the figure. The fabric for the pennants costs $1.25 per square foot. How much will it cost Alex to make 12 pennants?

$ _____
Answer: $52.50
Explanation:
Each pendant is made up of a rectangle and a triangle.
Area of the rectangle = length x breadth = 3 x 1 = 3 ft2
Area of the triangle = 1/2 x base x height = 1/2 x 1 x 1 = 0.5 ft2
The total area of the pendant = 3+0.5 = 3.5 ft2
Number of pendants = 12
Area of the pendants = 12 x 3.5 = 42 ft2
Cost of each square feet of the pendant = $1.25
Total cost for all the 12 pendants = 12 x $1.25 = $52.50
Question 11.
Reasoning
A composite figure is formed by combining a square and a triangle. Its total area is 32.5 ft2. The area of the triangle is 7.5 ft2. What is the length of each side of the square? Explain.
_____ ft
Answer: 5 ft
Explanation:
Given:
The area of the composite figure = 32.5 ft2
The area of the triangle = 7.5 ft2
The area of the square = 32.5 – 7.5 = 25
side x side = 25
side2 = 25
side = root 25 = 5 ft
H.O.T. – Page No. 282
Focus on Higher Order Thinking
Question 12.
Represent Real-World Problems
Christina plotted the shape of her garden on graph paper. She estimates that she will get about 15 carrots from each square unit. She plans to use the entire garden for carrots. About how many carrots can she expect to grow? Explain.

______ carrots
Answer: 300 carrots
Explanation:
This shape is divided into two triangles and a square.
Area of figure = 2(1/2 x 2 x 2) + 4(4) = 4 + 16 = 20 square units
Number of carrots per square unit = 300
Total number of carrots = 20 x 15 = 300
Question 13.
Analyze Relationships
The figure shown is made up of a triangle and a square. The perimeter of the figure is 56 inches. What is the area of the figure? Explain.
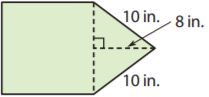
_____ in2
Answer: 192 in2
Explanation:
Given:
The perimeter of the figure = 56 inches
The figure is divided into a square and a triangle.
10 + 10 + 3s = 56
3s = 36
s = 12
The area of a triangle = 1/2 x 12 x 8 = 48 in2
The area of a square = 12 x 12 = 144 in2
Total area of the figure = 144 + 48 = 192 in2
Question 14.
Critical Thinking
The pattern for a scarf is shown at right. What is the area of the scarf? Use 3.14 for π.
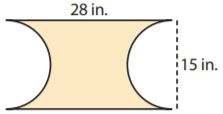
_____ in2
Answer: 243 in2
Explanation:
Area of the rectangle in the given figure = 28 x 15 = 420 in2
Area of two semicircles = 2 (1/2 πr2 ) = 3.14 x 7.5 x 7.5 = 176.625 in2
Area of the shaded region = 420 – 176.625 = 243 in2(approx)
Question 15.
Persevere in Problem Solving
The design for the palladium window shown includes a semicircular shape at the top. The bottom is formed by squares of equal size. A shade for the window will extend 4 inches beyond the perimeter of the window, shown by the dashed line around the window. Each square in the window has an area of 100 in2.
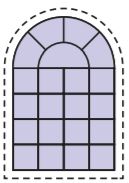
a. What is the area of the window? Use 3.14 for π.
_____ in2
Answer: a) 2228 in2
Explanation:
Area of the square = 100 in2
side x side = 100
Side = 10 in
Since the side of each square is 10 in and there are 4 squares.
The side length of the larger square (s) = 40 in
Area of the larger square = side x side = 40 x 40 = 1600 in2
Since the side of each square is 10 in and there are 2 squares.
The radius of the semi-circle = 20 in
Area of the semi-circle = 1/2(πr2) = 1/2(3.14 x 202) = 628 in2
The area of the window = 1600 + 628 = 2228 in2
Question 15.
b. What is the area of the shade? Round your answer to the nearest whole number.
_____ in2
Answer: b) 3016 in2
Explanation:
The shade extends 4 inches beyond the shapes so the length of the bottom rectangle is 40+4+4 = 48 in
The length extends below the original square.
The height is now = 40+4 = 44 in
The radius of the semi-circle = 20+4 = 24 in
The new area of the figure = 48(44) + 1/2(3.14 x 242) = 2112 + 904.32 = 3016.32 = 3016 in2
Guided Practice – Page No. 286
Find the surface area of each solid figure.
Question 1.
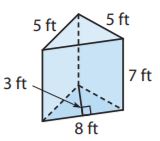
Total surface area: _____ ft2
Answer: 150 ft2
Explanation:
The base is a triangle with side lengths of 8 ft, 5 ft, 5 ft so the perimeter of the base = P = 8+5+5 = 18 ft
The height of the prism = 7 ft
The base is a triangle.
Area of the triangle = 1/2 (8) (3) = 12 ft2
The surface area formula for a prism is S = Ph + 2b
P = Perimeter = 18 h = height = 7 b = base = area of the triangle = 12
The surface area of the prism = 18(7) + 2(12) = 126 + 24 = 150 ft2
Question 2.
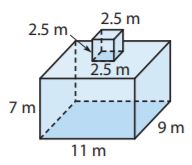
Total surface area: _____ m2
Answer: 503 m2
Explanation:
Given:
Dimensions of the cuboid:
Length = 11 m
Breadth = 9 m
Height = 7 m
The surface area of the cuboid = 2(lb+bh+hl) = 2(11 x 9 + 9 x 7 + 7 x 11) = 478m2
The dimensions of the cube:
Length of the side = 2.5 m
The surface area of the cube = 6a2 = 6 x 2.5 x 2.5 = 37.5 m2
The surface area of the rectangular prism = 2.5 x 2.5 = 6.25
The surface area of the figure = The overlapping area is the area of the base of the cube
= 37.5 + 478 – 2(6.25) = 503 m2
Essential Question Check-In
Question 3.
How can you find the surface area of a composite solid made up of prisms?
Type below:
_____________
Answer: The surface area of the prisms, add them up, and then subtract the overlapping areas twice.
Explanation:
The surface area of a composite solid is made up of prisms by finding the surface areas of the prisms, adding them up, and then up, and then subtracting the overlapping areas.
Independent Practice – Page No. 287
Question 4.
Carla is wrapping a present in the box shown. How much wrapping paper does she need, not including overlap?
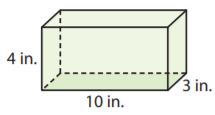
_____ in2
Answer: 164 in2
Explanation:
The surface area of the cuboid excluding the top = 2h(l+b) + lb = 2 x 4 ( 13 ) + 10 x 3 = 164 in2
The length of the wrapping paper = The surface area of the cuboid excluding the top = 164 in2
Question 5.
Dmitri wants to cover the top and sides of the box shown with glass tiles that are 5 mm square. How many tiles does he need?

_____ tiles
Answer: 3720 tiles
Explanation:
The surface area of the cuboid excluding the bottom = 2h(l+b) + lb = 2 x 9 (35) + 20 x 15 = 930 cm2
5mm = 0.5 cm
Area of a tile = Area of the square = a2 = 0.5cm x 0.5cm = 0.25 cm2
Total number of tiles = 930/0.25 = 3720 tiles
Question 6.
Shera is building a cabinet. She is making wooden braces for the corners of the cabinet. Find the surface area of each brace.
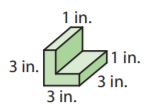
_____ in2
Answer: 45 in2
Explanation:
The perimeter of the figure = P = 3(3) + 2(1) = 11 in
Base = B = 3(2) = 6 in
Height = h = 3
The surface area of the figure = Ph + 2B = 11 x 3 +2(6) = 33 + 12 = 45 in2
Question 7.
The doghouse shown has a floor, but no windows. Find the total surface area of the doghouse, including the door.
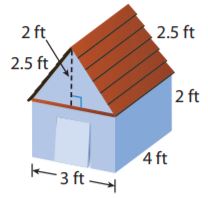
_____ ft2
Answer: 66ft2
Explanation:
Perimeter of the pentagon base (P) = 2(2.5) + 2(2) + 3 = 5 + 4 + 3 = 12
Area of the pentagon base by adding the area of the triangle and the area of the rectangle (B) = 1/2(3)(2) + 2(3) = 9
Height (h) = 2 + 2 = 4
The surface area of the figure = Ph + 2B = 12(4) + 2(9) = 48 + 18 = 66ft2
Eddie built the ramp shown to train his puppy to do tricks. Use the figure for 8–9.
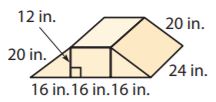
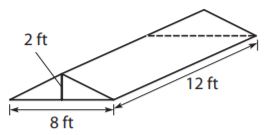
Question 8.
Analyze Relationships
Describe two ways to find the surface area of the ramp.
Type below:
____________
Answer: One way is to use the formula S = Ph + 2B. Another way is to find the area of each face of the prism and add them up to get the total surface area.
Explanation:
The very first way to use the formula S = Ph + 2B where the trapeziums are the base. The second way is to find the area of each face of the prism and then add them up to get the total surface area.
Question 9.
What is the surface area of the ramp?
_____ in2
Answer: 3264 in2
Explanation:
P = Perimeter of the figure = 16(3) + 2 (20) + 16 = 104
B = Base of the figure = 1/2 (12) (16 + 3(16)) = 6 (16 + 48) = 6 (64) = 384
h = Height of the figure = 2
Surface area of the figure = Ph + 2B = 104(2) + 2(384) = 2496 + 768 = 3264 in2
Marco and Elaine are building a stand like the one shown to display trophies. Use the figure for 10–11.
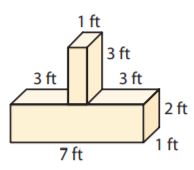
Question 10.
What is the surface area of the stand?
_____ ft2
Answer: 58 ft2
Explanation:
Top:
Perimeter = P = 4(1) = 4
Base = B = 1(1) = 1
Height = h = 3
Top surface area = Ph + 2B = 4(3) + 2(1) = 14 ft2
Bottom :
Perimeter = P = 2(7) + 2(1) = 14 + 2 = 16
Base = B = 7(1) = 7
Height = h = 2
Top surface area = Ph + 2B = 16(2) + 2(7) = 46 ft2
Overlapping area = 1(1) = 1
The surface area of the figure = The surface area of the top + The surface area of the bottom – the overlapping area = 14 + 46 – 2 = 60 – 2 = 58 ft2
Question 11.
Critique Reasoning
Marco and Elaine want to paint the entire stand silver. A can of paint covers 25 square feet and costs $6.79. They set aside $15 for paint. Is that enough? Explain.
_____
Answer: No
Explanation:
Since the surface area is 58 ft2, they will need 3 cans of paint. Since each can paints 25 ft2 and we cannot buy a fraction of cans.
3 cans would then cost 6.79 x 3 = 20.37 so this is not enough.
Page No. 288
Question 12.
Henry wants to cover the box shown with paper without any overlap. How many square centimeters will be covered with paper?
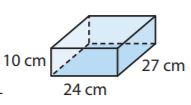
_____ cm2
Answer: 2316 cm2
Explanation:
Given:
Length = 24cm Breadth = 27cm Height = 10cm
P = Perimeter = 2(24) + 2(27) = 48 + 54 = 102
B = Base = 24(27) = 648
h = Height = 10
Surface area of the figure = Ph + 2B = 102(10) + 2(648) = 1020 + 1296 = 2316 cm2
Question 13.
What If?
Suppose the length and width of the box in Exercise 12 double. Does the surface area S double? Explain.
_____
Answer: No
Explanation:
Given :
Length = 24cm x 2 = 48 cm Breadth = 27cm x 2 = 54 cm Height = 10cm
P = 2(48) + 2(54) = 96 + 108 = 204
B = 48(54) = 2592
New Surface area = Ph + 2B = 204(10) + 2(2592) = 2040 + 5184 = 7224 cm2
Double of surface area = 2 (2316) = 4632 cm2
So the new surface area is not double of the initial area.
H.O.T.
Focus on Higher Order Thinking
Question 14.
Persevere in Problem Solving
Enya is building a storage cupboard in the shape of a rectangular prism. The rectangular prism has a square base with side lengths of 2.5 feet and a height of 3.5 feet. Compare the amount of paint she would use to paint all but the bottom surface of the prism to the amount she would use to paint the entire prism.
Type below:
______________
Answer: The difference would just be the area in the bottom surface. It would be 6.25 ft2 less.
Explanation:
The difference in the amount of paint would just be the area of the bottom surface. The area of the bottom surface is (2.5)2 = 6.25.
Therefore she would paint 6.25 ft2 less if she painted all but the bottom surface compared to painting the entire prism.
Question 15.
Interpret the Answer
The oatmeal box shown is shaped like a cylinder. Use a net to find the surface area S of the oatmeal box to the nearest tenth. Then find the number of square feet of cardboard needed for 1,500 oatmeal boxes. Round your answer to the nearest whole number
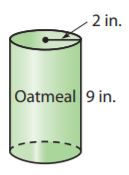
_____ ft2
Answer: 138.28 in2 , 1440 ft2
Explanation:
Given:
Dimensions of the cylinder:
Radius: 2 in
Height: 9 in
The total surface area of the cylinder = 2πr(r+h) = 2 x 22/7 x 2 (2 + 9) = 138.28 in2
The total number of square inches needed for 1,500 oatmeal boxes = 1,500 x 138.28 = 207,300 in2
1 ft = 12 in
(1 ft)2 = (12 in)2
1 ft2 = 144 in2
The total number of square feet needed for 1,500 oatmeal boxes (to the nearest whole number)
= 207,300/144 = 1440 ft2
Question 16.
Analyze Relationships
A prism is made of centimeter cubes. How can you find the surface area of the prism in Figure 1 without using a net or a formula? How does the surface area change in Figures 2, 3, and 4? Explain.
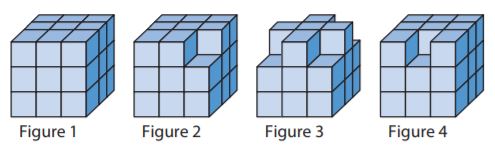
Type below:
______________
Answer: The surface area for the first 3 figures are the same. The surface area for figure 4 is greater than the surface area of the figures 1 – 3.
Explanation:
The surface area of the first 3 figures is the same. The 3 new faces on figure 2 have the same areas as the 3 visible faces that were removed when the top corner cube was removed. The surface area is then the same as it is for figure 1. Similarly, the areas of the new visible faces in figure 3 are equal to the areas of the visible faces removed from removing the corner cubes so the surface areas are the same as in figure 1. The surface area for figure 4 is greater than the surface areas of the figures 1 – 3. Removing the cube removed 2 of the visible faces (one from the top and one from the front side) but added 4 visible faces so the surface area increases.
Guided Practice – Solving Volume Problems – Page No. 292
Question 1.
Find the volume of the triangular prism.
_____ ft3
Answer: 84 ft3
Explanation:
Base area of the prism = 1/2 x 8 x 3 = 12 ft2
Height of the prism = 7 ft
Volume of the prism = (12 x 7) ft3
Question 2.
Find the volume of the trapezoidal prism.
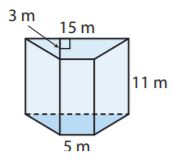
_____ m3
Answer: 330 m3
Explanation:
Base area of the prism = 1/2 x (15 + 5) x 3 = 30 m2
Height of the prism = 11 m
Volume of the prism = (30 x 11) m3 = 330 m3
Question 3.
Find the volume of the composite figure.
_____ ft2
Answer: Composite figure: 360 ft3
Explanation:
The volume of the triangular prism:
The base area of the prism = 1/2 x 4 x 6 = 12 ft2
Height = 6 ft
The volume of the triangular prism = 12 x 6 = 72 ft3
The volume of the rectangular prism:
The base area of the prism = 4 x 6 = 24 ft2
Height = 12 ft
The volume of the triangular prism = 12 x 24 = 288 ft3
Volume of the composite figure = (288 + 72)ft3 = 360 ft3
Find the volume of each figure.
Question 4.
The figure shows a barn that Mr. Fowler is building for his farm.
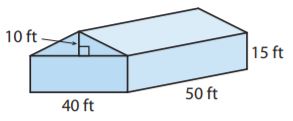
_____ ft3
Answer: 40,000 ft3
Explanation:
Triangular prism:
B = Base area = 1/2 x 10 (40) = 200 cm2
Height = 50 cm
The volume of the triangular prism = Bh = 200 x 50 = 10,000 cm3
Rectangular prism:
B = Base area =40 x 15 = 600 cm2
Height = 50 cm
The volume of the triangular prism = Bh = 600 x 50 = 30,000 cm3
Total volume of the prism = 10,000 + 30,000 = 40,000 cm3
Question 5.
The figure shows a container, in the shape of a trapezoidal prism, that Pete filled with sand.

_____ cm3
Answer: 385 cm3
Explanation:
B = Base area = 1/2 x 5 (10 + 12) = 55 cm2
Height = 7 cm
The volume of the container = Bh = 55 x 55 = 385 cm3
Essential Question Check-In
Question 6.
How do you find the volume of a composite solid formed by two or more prisms?
Type below:
______________
Answer: Finding the volume of each figure adding them up to get the volume of the composite solid.
Explanation:
To find the volume of the composite figure that can be divided into 2 or more prisms, find the volume of each prism and add them up to get the volume of the composite solid.
Independent Practice – Page No. 293
Question 7.
A trap for insects is in the shape of a triangular prism. The area of the base is 3.5 in2 and the height of the prism is 5 in. What is the volume of this trap?
_____ in3
Answer: 17.5 in3
Explanation:
The volume of the trap = Base area x height = 3.5 x 5 = 17.5 in3
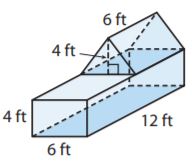
Question 8.
Arletta built a cardboard ramp for her little brothers’ toy cars. Identify the shape of the ramp. Then find its volume.
Shape: _________
Area: _________ in3
Answer: 525 in3
Explanation:
Base area = 1/2 x 6 x 25 = 75 in2
Height = 7 in
Volume of the figure = 75 x 7 = 525 in3
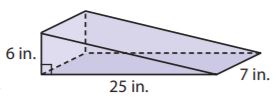
Question 9.
Alex made a sketch for a homemade soccer goal he plans to build. The goal will be in the shape of a triangular prism. The legs of the right triangles at the sides of his goal measure 4 ft and 8 ft, and the opening along the front is 24 ft. How much space is contained within this goal?

_____ ft3
Answer: 384 ft3
Explanation:
Base area = 1/2 x 4 x 8 = 16 ft2
Height = 24 ft
Volume of the figure = 16 x 24 = 384 ft3
Question 10.
A gift box is in the shape of a trapezoidal prism with base lengths of 7 inches and 5 inches and a height of 4 inches. The height of the gift box is 8 inches. What is the volume of the gift box?
_____ in3
Answer: 192 in3
Explanation:
Base area = 1/2 x 4 x (7+5) = 24 in2
Height = 8 in
Volume of the figure = 24 x 8 = 192 Base area = 1/2 x 6 x 25 = 75 in2
Height = 7 in
Volume of the figure = 75 x 7 = 525 in3
Question 11.
Explain the Error
A student wrote this statement: “A triangular prism has a height of 15 inches and a base area of 20 square inches. The volume of the prism is 300 square inches.” Identify and correct the error.
Type below:
____________
Answer: The error is measurement unit.
Explanation:
The volume of the prism is:
base area x height = 20 x 15 = 300 in3
Find the volume of each figure. Round to the nearest hundredth if necessary.
Question 12.
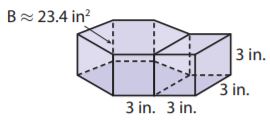
_____ in3
Answer: 97.2 in3
Explanation:
The volume of the hexagonal prism = 23.4 x 3 = 70.2 in3
Base area of the rectangular prism = 3 x 3 = 9 in2
The volume of the rectangular prism = Bh = 9 x 3 = 27 in3
Total volume of the figure = 70.2 + 27 = 97.2 in3
Question 13.
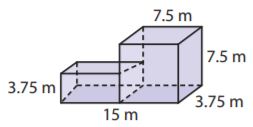
_____ m3
Answer: 316.41 m3
Explanation:
The volume of the rectangular prism on the left = Bh = [7.5 x 3.75] (3.75) = 105.47 m3
The volume of the rectangular prism on the right = Bh = [7.5 x 3.75](7.5) = 210.94 m3
Total volume of the composite figure = 105.47 + 210.94 = 316.41 m3
Question 14.
Multi-Step
Josie has 260 cubic centimeters of candle wax. She wants to make a hexagonal prism candle with a base area of 21 square centimeters and a height of 8 centimeters. She also wants to make a triangular prism candle with a height of 14 centimeters. Can the base area of the triangular prism candle be 7 square centimeters? Explain.
_____
Answer: No
Explanation:
The volume of the hexagonal prism = 21 x 8 = 168
The total volume of wax, 260 is equal to the sum of the volumes of each prism.
B is the base area of the triangular prism.
168 + 14B = 260 cm3
14B = 260 – 168
B = 6.6 cm3
Page No. 294
Question 15.
A movie theater offers popcorn in two different containers for the same price. One container is a trapezoidal prism with a base area of 36 square inches and a height of 5 inches. The other container is a triangular prism with a base area of 32 square inches and a height of 6 inches. Which container is the better deal? Explain.

Type below:
___________
Answer: The triangular prism is a better deal since it has a larger volume
Explanation:
The base area of the trapezoidal prism = 36 in2
The volume of the trapezoidal prism = Bh = 36 x 5 = 175 in3
The base area of the triangular prism = 32 in2
The volume of the rectangular prism = Bh = 32 x 6 = 192 in3
The triangular prism is a better deal since it has a larger volume.
H.O.T.
Focus on Higher Order Thinking
Question 16.
Critical Thinking
The wading pool shown is a trapezoidal prism with a total volume of 286 cubic feet. What is the missing dimension?
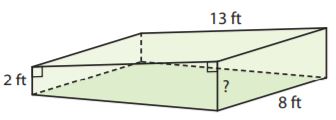
______ ft.
Answer: 3.5 ft
Explanation:
Area of the trapezoidal prism = B = 1/2 x 13 (2+x)
Volume of the figure = 286 cubic feet
V = Bh
286 = 1/2 x 13 (2+x)(8)
5.5 = (2+x)
x = 3.5 ft
Question 17.
Persevere in Problem Solving
Lynette has a metal doorstop with the dimensions shown. Each cubic centimeter of the metal in the doorstop has a mass of about 8.6 grams. Find the volume of the metal in the doorstop. Then find the mass of the doorstop.
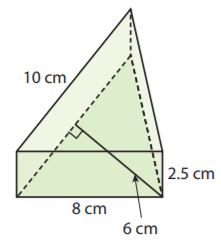
______ grams
Answer: 75 cubic centimeter, 645 grams
Explanation:
V = Bh
B = Area of the triangle of base = 10 cm , height = 6 cm = 1/2 x 10 x 6 = 30 square centimeter
V = 30 x 2.5 = 75 cubic centimeter
1 cubic centimeter = 8.6 grams in mass
V = 75 cubic centimeter x 8.6 = 645 grams
Question 18.
Analyze Relationships
What effect would tripling all the dimensions of a triangular prism have on the volume of the prism? Explain your reasoning.
Type below:
____________
Answer: The volume is 27 times the original volume.
Explanation:
The area of the base = B = 1/2 (3b) (3h) = 9/2 (bh)
H is the height of the prism
The volume would be = 9/2 (bh) x (3H) = 27 [ 1/2 (bhH) ]
Therefore, The volume is 27 times the original volume.
Question 19.
Persevere in Problem Solving
Each of two trapezoidal prisms has a volume of 120 cubic centimetres. The prisms have no dimensions in common. Give possible dimensions for each prism.
Type below:
____________
Answer: A possible combination of dimension could be the height at 8 cm, base at 2 cm and 3 cm
Explanation:
The numbers that multiply to get 120 are 20 and 6 so let the first prism have a base area of 20 square centimetres and the height of 6 cm.
If the base area is 20, the height of the trapezoid and the length of the bases could be 8,2 and 3 respectively.
The other numbers that multiply to get 120 are 4 and 30 so let the second prism have a base area of 30 square centimetres and the height of 4 cm.
If the base area is 30, the height of the trapezoid and the length of the bases could be 10,1 and 5 respectively.
9.1, 9.2 Circumference and Area of Circles – Page No. 295
Find the circumference and area of each circle. Use 3.14 for π. Round to the nearest hundredth if necessary.
Question 1.
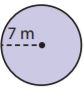
C = _________ m
A = _________ m2
Answer:
C = 43.96 m
A = 153.86 m2
Explanation:
C = 2 πr = 2 π(7) = 14 (3.14) = 43.96 m
A = πr2 = 3.14 (7)2 = 153.86 m2
Question 2.
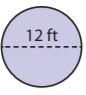
C = _________ ft
A = _________ ft2
Answer:
C = 37.68 ft
A = 113.04 ft2
Explanation:
Diameter = 12 ft
Radius = d/2 = 12/2 = 6 ft
C = 2 πr = 2 π(6) = 6 (3.14) = 37.68 ft
A = πr2 = 3.14 (6)2 = 113.04 ft2
9.3 Area of Composite Figures
Find the area of each figure. Use 3.14 for π.
Question 3.

______ m2
Answer: 180.48 m2
Explanation:
Area of the triangle = 1/2 x 16 x 10 = 80 m2
Area of the semicircle = 1/2 πr2 = 1/2 (3.14) (8)2 = 100.48 m2
The total area of the figure = 80 + 100.48 = 180.48 m2
Question 4.
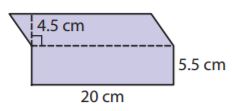
______ cm2
Answer: 200 cm2
Explanation:
Area of the parallelogram = 4.5(20) = 90 cm2
Area of the rectangle = 20(5.5) = 110 cm2
The total area of the figure = 90 + 110 = 200 cm2
9.4, 9.5 Solving Surface Area and Volume Problems
Find the surface area and volume of each figure.
Question 5.
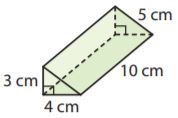
S = _________ cm2
V = _________ cm3
Answer:
S = 132 cm2
V = 60 cm3
Explanation:
Perimeter = 3+4+5 = 12 cm
Base area = Area of the triangle = 1/2 x 3 x 4 = 6
S = Ph + 2B = 12(10) + 2(6) = 120 +12 = 132 cm2
V = Bh = 6 x 10 = 60 cm3
Question 6.
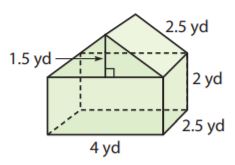
S = _________ yd2
V = _________ yd3
Answer:
S = 54.5 yd2
V = 27.5 yd3
Explanation:
Perimeter = 2(2.5) + 2(2) + 4 = 13 cm
Base area = Area of the triangle + Area of the rectangle = 1/2 x 1.5 x 4 + 4(2)= 11
S = Ph + 2B = 13(2.5) + 2(11) = 32.5 +22 = 54.5 yd2
V = Bh = 11 x 2.5 = 27.5 yd3
Essential Question
Question 7.
How can you use geometry figures to solve real-world problems?
Type below:
______________
Answer: We can solve real-world problems by finding surface area and volume.
Example: We can find the amount of liquid in a tank by calculating its volume.
Explanation:
Real-world problems by finding surface area and volume.
Example1: We can find the amount of liquid in a tank by calculating its volume.
Example2: We can find the surface area of the house and find the amount of paint required to paint the house.
Page No. 296
Question 1.
What is the circumference of the circle?
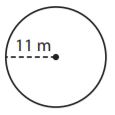
a. 34.54 m
b. 69.08 m
c. 379.94 m
d. 1519.76 m
Answer: b. 69.08 m
Explanation:
Circumference = 2 πr = 2 π(11) = 22 (3.14) = 69.08 m
Question 2.
What is the area of the circle?
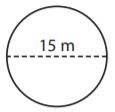
Options:
a. 23.55 m2
b. 47.1 m2
c. 176.625 m2
d. 706.5 m2
Answer: c. 176.625 m2
Explanation:
Diameter = 15 m
Radius = 7.5 m
Area of the circle = πr2 = 3.14 (7.5)2 = 176.625 m2
Question 3.
What is the area of the figure?
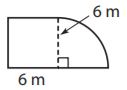
Options:
a. 28.26 m2
b. 36 m2
c. 64.26 m2
d. 92.52 m2
Answer: c. 64.26 m2
Explanation:
Area of the square = 6 x 6 = 36 m2
Radius = 6 m
Area of the quarter circle = 1/4 πr2 = 1/4 x 3.14 (6)2 = 28.26 m2
The total area of the figure = 36 + 28.26 = 64.26 m2
Question 4.
A one-year membership to a health club costs $480. This includes a $150 fee for new members that is paid when joining. Which equation represents the monthly cost x in dollars for a new member?
Options:
a. 12x + 150 = 480
b. \(\frac{x}{12}\) + 150 = 480
c. 12x + 480 = 150
d. \(\frac{x}{12}\) + 480 = 150
Answer: a. 12x + 150 = 480
Explanation:
If x is the monthly fee, then 12x is the total monthly fees.
The joining fee = $150
Total cost = $480
then,
12x + 150 = 480
Question 5.
What is the volume of the prism?
Options:
a. 192 ft3
b. 48 ft3
c. 69 ft3
d. 96 ft3
Answer: d. 96 ft3
Explanation:
B = Base area of the triangle = 1/2 x 8 x 2 = 8 ft2
Height = 12 ft
Volume of the triangular orism = Bh = 8(12) = 96 ft3
Question 6.
A school snack bar sells a mix of granola and raisins. The mix includes 2 pounds of granola for every 3 pounds of raisins. How many pounds of granola are needed for a mix that includes 24 pounds of raisins?
Options:
a. 16 pounds
b. 36 pounds
c. 48 pounds
d. 120 pounds
e. 120 pounds
Answer: a. 16 pounds
Explanation:
2/3 is equal to x/24 then 3 times 8 is equal to 24 and if 2 times 8 is equal to 16.
Question 7.
Find the percent change from $20 to $25.
Options:
a. 25% decrease
b. 25% increase
c. 20% decrease
d. 20% increase
Answer: b. 25% increase
Explanation:
25 – 20 = 5 divide by 20 = 1/4
When we find the percentage we get 25.
So we can say that there is an increase in 25%
Question 8.
Each dimension of the smaller prism is half the corresponding dimension of the larger prism.
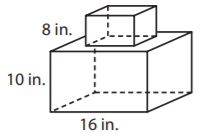
a. What is the surface area of the figure?
_____ in2
Answer: 856 in2
Explanation:
Height of the top prism = 10/2 = 5
Length of the top prism = 16/2 = 8
Width of the top prism = 8/2 = 4
Perimeter = 2l + 2w = 2(8) + 2(4) = 16 + 8 = 24 in
B = lw = 8(4) = 32 in
Surface area of top prism= Ph + 2B = 24(5) + 2(32) = 184 in2
Height of the prism = 10
Length of the prism = 16
Width of the prism = 8
Perimeter = 2l + 2w = 2(16) + 2(8) = 32 + 16 = 48 in
B = lw = 16(8) = 128 in
Surface area of bottom prism= Ph + 2B = 48(10) + 2(128) = 736 in2
Area of overlapping region = 32 in2
The total surface area of the prism
= Surface area of top prism + Surface area of bottom prism – 2[Area of overlapping region ]
= 184 + 736 – 2(32) = 856 in2
Question 8.
b. What is the volume of the figure?
_____ in3
Answer: 1440 in3
Explanation:
Volume of top prism = Bh = 32(5) = 160 in3
Volume of bottom prism = Bh = 128(10) = 1280 in3
The total volume of the figure = 160 + 1280 = 1440 in3
EXERCISES – Page No. 298
Question 1.
In the scale drawing of a park, the scale is 1 cm: 10 m. Find the area of the actual park.
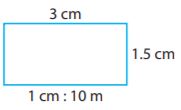
_____ m2
Answer: 450 m2
Explanation:
Multiply the dimensions of the scale drawing by 10 since 1 cm = 10 m
3cm by 1.5 cm = 30m by 15 m
Area = 30(15) = 450 m2
Question 2.
Find the value of y and the measure of ∠YPS.
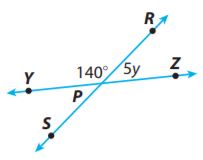
y = __________ °
mYPS = __________ °
Answer: y = 8
mYPS = 40 °
Explanation:
140 + 5y = 180 [sum of angle on a line = 180°]
5y = 40
y = 8
mYPS = mRPZ = 5y [vertically opposite angles]
mYPS = 5(8) = 40°
Question 3.
Kanye wants to make a triangular flower bed using logs with the lengths shown below to form the border. Can Kanye form a triangle with the logs without cutting any of them? Explain.
_____
Answer: No
Explanation:
A side of a triangle must be greater than the difference of the other two sides and smaller than the sum of the other 2 sides.
The sum of the first 2 sides = 3+4 = 7 < 8
Therefore, he cannot form a triangle unless he cuts the logs.
Question 4.
In shop class, Adriana makes a pyramid with a 4-inch square base and a height of 6 inches. She then cuts the pyramid vertically in half as shown. What is the area of each cut surface?
_____ in2
Answer: 12 in2
Explanation:
Base = 4 in
Height = 6 in
Area of the triangle = 1/2 x 6 x 4 = 12 in2
Page No. 300
Find the circumference and area of each circle. Round to the nearest hundredth.
Question 1.
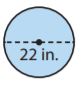
C = __________ in
A = __________ in2
Answer:
C = 69.08 in
A = 379.94 in2
Explanation:
Diameter = 22 in
Radius = d/2 = 22/2 = 11 in
C = 2 πr = 2 π(11) = 22 (3.14) = 69.08 in
A = πr2 = 3.14 (11)2 = 379.94 in2
Question 2.
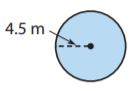
C = __________ m
A = __________ m2
Answer:
C = 28.26 m
A = 63.59m2
Explanation:
Radius = 4.5 m
C = 2 πr = 2 π(4.5) = 9 (3.14) = 28.26 m
A = πr2 = 3.14 (4.5)2 = 63.59 m2
Find the area of each composite figure. Round to the nearest hundredth if necessary.
Question 3.
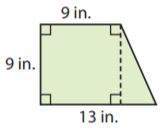
______ in2
Answer: 99 in2
Explanation:
Area of the square = 9 x 9 = 81 in2
Base of the triangle = 13 – 9 = 4 in
Area of the triangle = 1/2 x 4 x 9 = 18 in2
The total area of the figure = 81 + 18 = 99 in2
Question 4.
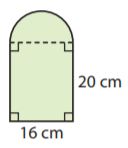
______ cm2
Answer: 420.48 cm2
Explanation:
Area of the rectangle = 16 x 20 = 320 cm2
Diameter = 16 cm
Radius = 16/2 = 8 cm
Area of the semi circle = 1/2 πr2 = 1/2 x 3.14 (8)2 = 100.48 cm2
The total area of the figure = 320 + 100.48 = 420.48 cm2
Find the volume of each figure.
Question 5.
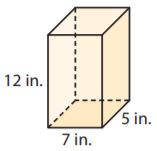
______ in3
Answer: 420 in3
Explanation:
B = 7(5) = 35 in2
V = Bh = 35 x 12 = 420 in3
Question 6.
The volume of a triangular prism is 264 cubic feet. The area of a base of the prism is 48 square feet. Find the height of the prism.
______ in
Answer: 5.5 ft
Explanation:
V = Bh
264 = 48h
h = 264/48 = 5.5ft
Page No. 301
A glass paperweight has a composite shape: a square pyramid fitting exactly on top of an 8 centimeter cube. The pyramid has a height of 3 cm. Each triangular face has a height of 5 centimeters.
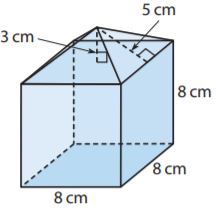
Question 7.
What is the volume of the paperweight?
______ cm3
Answer: 576 cm3
Explanation:
Pyramid:
B = 8 x 8 = 64 cm2
V = 1/3 Bh = 1/3 x 64 x 3 = 64 cm3
Prism:
B = 8 x 8 = 64 cm2
V = Bh = 64 x 8 = 512 cm3
The total volume of the figure = 64 + 512 = 576 cm3
Question 8.
What is the total surface area of the paperweight?
______ cm2
Answer: 400 cm2
Explanation:
Pyramid:
P = 4(8) = 32 cm
S = 1/2 Pl + B = 80 + 64 = 144 cm2
Prism:
P = 4(8) = 32 cm
S = Ph + 2B = 32(8) + 2(64) = 384 cm2
The total surface area of the prism
= Area of the prism + Area of the pyramid – 2[Area of the overlapping region]
= 144 + 384 – 2(64) = 400
Unit 4 Performance Tasks
Question 9.
Product Design Engineer
Miranda is a product design engineer working for a sporting goods company. She designs a tent in the shape of a triangular prism. The dimensions of the tent are shown in the diagram.
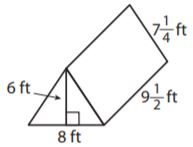
a. How many square feet of material does Miranda need to make the tent (including the floor)? Show your work.
______ ft2
Answer: 261 3/4 ft2
Explanation:
P = 2 x 7 1/2 + 8 = 22 1/2
B = 4/2 (8) (6) = 24
S = Ph + 2B = 22 1/2 x 9 1/2 + 2(24) = 213 3/4 + 48 = 261 3/4 ft2
Question 9.
b. What is the volume of the tent? Show your work.
______ ft3
Answer: 228 ft3
Explanation:
V = Bh = 24 x 9 1/2 = 228 ft3
Question 9.
c. Suppose Miranda wants to increase the volume of the tent by 10%. The specifications for the height (6 feet) and the width (8 feet) must stay the same. How can Miranda meet this new requirement? Explain
Type below:
____________
Answer: Increase the height to 10.45 ft
Explanation:
New volume = 1.10 x 228 = 250.8
250.8 = 24h
h = 10.45 ft
Unit 4 Performance Tasks (cont’d) – Page No. 302
Question 10.
Li is making a stand to display a sculpture made in art class. The stand will be 45 centimeters wide, 25 centimeters long, and 1.2 meters high.
a. What is the volume of the stand? Write your answer in cubic centimeters.
______ cm3
Answer: 135,000 cm3
Explanation:
B = 45 x 25 = 1125 cm2
V = Bh = 1125 x 120 = 135,000 cm3
Question 10.
b. Li needs to fill the stand with sand so that it is heavy and stable. Each piece of wood is 1 centimeter thick. The boards are put together as shown in the figure, which is not drawn to scale. How many cubic centimeters of sand does she need to fill the stand? Explain how you found your answer.
______ cm3
Answer: 116,702 cm3
Explanation:
Width = 45 – 2(1) = 43 ft
Length = 25 – 2(1) =23ft
Height = 120-2(1) = 118ft
B = 43 x 23 = 989 ft2
V = Bh = 989 x 118 = 116,702 ft3
Selected Response – Page No. 303
Question 1.
A school flag is in the shape of a rectangle with a triangle removed as shown.
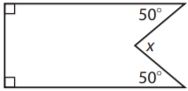
What is the measure of angle x?
Options:
a. 50°
b. 80°
c. 90°
d. 100°
Answer: d. 100°
Explanation:
x = 50 + 50 = 100° [ Sum of two angles created by the 2 lines]
Question 2.
On a map with a scale of 2 cm = 1 km, the distance from Beau’s house to the beach is 4.6 centimetres. What is the actual distance?
Options:
a. 2.3 km
b. 4.6 km
c. 6.5 km
d. 9.2 km
Answer: a. 2.3 km
Explanation:
2/1 = 4.6/x
x = 4.6/2 = 2.3 km
Question 3.
Lalasa and Yasmin are designing a triangular banner to hang in the school gymnasium. They first draw the design on paper. The triangle has a base of 5 inches and a height of 7 inches. If 1 inch on the drawing is equivalent to 1.5 feet on the actual banner, what will the area of the actual banner be?
Options:
a. 17.5 ft2
b. 52.5 ft2
c. 39.375 ft2
d. 78.75 ft2
Answer: c. 39.375 ft2
Explanation:
1in = 1.5ft
The base of the triangle = 5 in = 1.5(5) ft = 7.5 ft
Height = 7 in = 7(1.5) ft = 10.5 ft
Area of the triangle = 1/2 x 7.5 x 10.5 = 39.375 ft2
Question 4.
Sonya has four straws of different lengths: 2 cm, 8 cm, 14 cm, and 16 cm. How many triangles can she make using the straws?
Options:
a. no triangle
b. one triangle
c. two triangles
d. more than two triangles
Answer: b. one triangle
Explanation:
The third side of a triangle must be smaller than the sum of the other two sides to form a triangle.
2+8 = 10<14
2+8 = 10<16
8+14 = 22>14
8+14 = 22>16
2+14 = 16=16
2+16 = 18>16
Therefore, only one triangle can be formed using the sides 8, 14, 16.
Question 5.
A one-topping pizza costs $15.00. Each additional topping costs $1.25. Let x be the number of additional toppings. You have $20 to spend. Which equation can you solve to find the number of additional toppings you can get on your pizza?
Options:
a. 15x + 1.25 = 20
b. 1.25x + 15 = 20
c. 15x − 1.25 = 20
d. 1.25x − 15 = 20
Answer: b. 1.25x + 15 = 20
Explanation:
If x is the number of additional toppings, then 1.25 x is the cost of the additional toppings.
This gives the total cost is 1.25x + 15
then,
1.25x + 15 = 20
Question 6.
A bank offers a home improvement loan with simple interest at an annual rate of 12%. J.T. borrows $14,000 over a period of 3 years. How much will he pay back altogether?
Options:
a. $15680
b. $17360
c. $19040
d. $20720
Answer: c. $19040
Explanation:
Simple interest = 14,000 x 0.12 x 2 = $5,040
Amount = $14,000 + $5,040 = $19040
Question 7.
What is the volume of a triangular prism that is 75 centimeters long and that has a base with an area of 30 square centimeters?
Options:
a. 2.5 cm3
b. 750 cm3
c. 1125 cm3
d. 2250 cm3
Answer: d. 2250 cm3
Explanation:
V = Bh = 30(75) = 2250cm3
Question 8.
Consider the right circular cone shown.
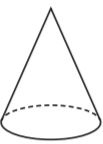
If a vertical plane slices through the cone to create two identical half cones, what is the shape of the cross section?
Options:
a. a rectangle
b. a square
c. a triangle
d. a circle
Answer: c. a triangle
Explanation:
Slicing through the vertex to create 2 identical half cones would create a cross-section that is a triangle.
Page No. 304
Question 9.
The radius of the circle is given in meters. What is the circumference of the circle? Use 3.14 for π.
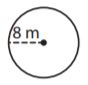
a. 25.12 m
b. 50.24 m
c. 200.96 m
d. 803.84 m
Answer: b. 50.24 m
Explanation:
Circumference = 2 πr = 2 π(8) = 16 (3.14) = 50.24 m
Question 10.
The dimensions of the figure are given in millimeters. What is the area of the two-dimensional figure?
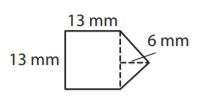
Options:
a. 39 mm2
b. 169 mm2
c. 208 mm2
d. 247 mm2
Answer: c. 208 mm2
Explanation:
Area of the square = 13 x 13 = 169 mm2
Area of the triangle = 1/2 x 13 x 6 = 39 mm2
The total area of the figure = 169 + 39 = 208 mm2
Question 11.
A forest ranger wants to determine the radius of the trunk of a tree. She measures the circumference to be 8.6 feet. What is the trunk’s radius to the nearest tenth of a foot?
Options:
a. 1.4 ft
b. 2.7 ft
c. 4.3 ft
d. 17.2 ft
Answer: a. 1.4 ft
Explanation:
Circumference = 2 πr = 8.6 ft
r = 8.6/2 π = 1.4 ft
Question 12.
What is the measure in degrees of an angle that is supplementary to a 74° angle?
Options:
a. 16°
b. 74°
c. 90°
d. 106°
Answer: d. 106°
Explanation:
Sum of supplementary angles = 180°
x + 74° = 180°
x = 106°
Question 13.
What is the volume in cubic centimeters of a rectangular prism that has a length of 6.2 centimeters, a width of 3.5 centimeters, and a height of 10 centimeters?
Options:
a. 19.7 cm3
b. 108.5 cm3
c. 217 cm3
d. 237.4 cm3
Answer: c. 217 cm3
Explanation:
V = Bh
B = 6.2 x 3.5 = 21.7 cm2
h = 10 cm
V = 21.7 x 10 = 217 cm3
Question 14.
A patio is the shape of a circle with diameter shown.
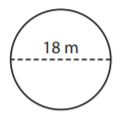
What is the area of the patio? Use 3.14 for π.
Options:
a. 9 m2
b. 28.26 m2
c. 254.34 m2
d. 1017.36 m2
Answer: c. 254.34 m2
Explanation:
Diameter = 18 m
Radius = 18/2 = 9 m
Area of the patio = πr2 = 3.14 (9)2 = 254.34 m2
Question 15.
Petra fills a small cardboard box with sand. The dimensions of the box are 3 inches by 4 inches by 2 inches.
a. What is the volume of the box?
______ in3
Answer: 24 in3
Explanation:
V = Bh
B = 3 x 4 = 12 in2
V = 12 x 2 = 24 in3
Question 15.
b. Petra decides to cover the box by gluing on wrapping paper. How much wrapping paper does she need to cover all six sides of the box?
______ in2
Answer: 76 in2
Explanation:
P = 2(3) + 2(4) = 6 + 8 = 14 in
S = Ph + 2B = 14 x 2 + 2 x 24 = 76 in2
Question 15.
c. Petra has a second, larger box that is 6 inches by 8 inches by 4 inches. How many times larger is the volume of this second box? The surface area?
Volume is _________ times greater.
Surface area is _________ times greater
Answer: Surface area is about 2.7 times larger
Explanation:
B = 6 x 8 = 48 in2
V = Bh = 48 x 4 = 192 in3
192/24 = 8
P = 2(6) + 2(8) = 12 + 16 = 28
S = Ph + 2B = 28(4) + 2(48) = 112 + 96 = 208 in2
208/76 = 2.7