Go Math Grade 6 Answer Key Chapter 11 Surface Area and Volume

Go Math Grade 6 Answer Key Chapter 11 Surface Area and Volume contains 6th Standard Go Math solutions which will make students understand the concepts easily help the students to score well in the exams. This Go Math Grade 6 Answer Key Chapter 11 Surface Area and Volume. And in this, each and every question was explained intimately. The answers in this chapter are explained in a simple way that anyone can understand easily.

Go Math Grade 6 Answer Key Chapter 11 Surface Area and Volume

This chapter 11 contains Three-Dimensional Figures and Nets, Explore Surface Area Using Nets, Surface Area of Prisms, etc. are explained clearly which makes the scholars learn quickly. Go Math Grade 6 Answer Key Chapter 11 Surface Area and Volume. questions are explained in a basic way that students will never feel any difficulty in learning. By this, students can gain good knowledge and this is helpful in finish student’s assignments also.

Lesson 1: Three-Dimensional Figures and Nets

• Share and Show – Page No. 599
• Problem Solving + Applications – Page No. 600
• Three-Dimensional Figures and Nets – Page No. 601
• Lesson Check – Page No. 602

Lesson 2: Investigate • Explore Surface Area Using Nets

• Share and Show – Page No. 605
• What’s the Error? – Page No. 606
• Explore Surface Area Using Nets – Page No. 607
• Lesson Check – Page No. 608

Lesson 3: Algebra • Surface Area of Prisms

• Share and Show – Page No. 611
• Unlock the Problem – Page No. 612
• Surface Area of Prisms – Page No. 613
• Lesson Check – Page No. 614

Lesson 4: Algebra • Surface Area of Pyramids

• Share and Show – Page No. 617
• Problem Solving + Applications – Page No. 618
• Surface Area of Pyramids – Page No. 619
• Lesson Check – Page No. 620

 Mid-Chapter Checkpoint

• Mid-Chapter Checkpoint – Vocabulary – Page No. 621
• Page No. 622

Lesson 5: Investigate • Fractions and Volume

• Share and Show – Page No. 625
• Problem Solving + Applications – Page No. 626
• Fractions and Volume – Page No. 627
• Lesson Check – Page No. 628

Lesson 6: Algebra • Volume of Rectangular Prisms

• Share and Show – Page No. 631
• Aquariums – Page No. 632
• Volume of Rectangular Prisms – Page No. 633
• Lesson Check – Page No. 634

Lesson 7: Problem Solving • Geometric Measurements

• Share and Show – Page No. 637
• On Your Own – Page No. 638
• Problem Solving Geometric Measurements – Page No. 639
• Lesson Check – Page No. 640

Chapter 11 Review/Test

• Chapter 11 Review/Test – Page No. 641
• Page No. 642
• Page No. 643
• Page No. 644
• Page No. 645
• Page No. 646

Share and Show – Page No. 599

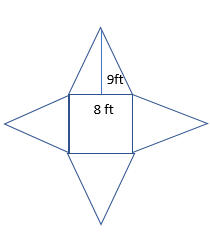
Identify and draw a net for the solid figure.

Question 1.

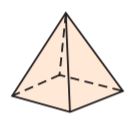
Answer: The base Square or Rectangle, and lateral faces are Triangle and the figure is a Square pyramid or Rectangular pyramid.

Explanation:

Question 2.

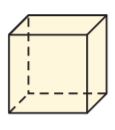
Answer: Cube or Rectangular prism.

Explanation: The base is a square or rectangle and lateral faces are squares are rectangle. The figure is a Cube or Rectangular prism.

Identify and sketch the solid figure that could be formed by the net.

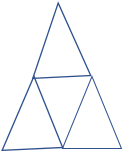
Question 3.

Answer: Triangular pyramid.

Explanation: The net has four triangles, so it is a triangular pyramid.

Question 4.

Answer: Cube

Explanation: The net has six squares.

On Your Own

Identify and draw a net for the solid figure.

Question 5.

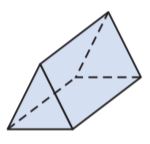
Answer: Triangular prism.

Explanation: The base is a rectangle and the lateral faces are triangle and rectangles, so it is a triangular prism.

Question 6.

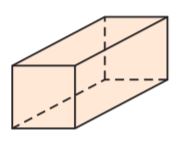
Answer:  Rectangular Prism.

Explanation: The base is a rectangle and the lateral faces are squares and rectangles. And it is a Rectangular prism.

Problem Solving + Applications – Page No. 600

Solve.

Question 7.
The lateral faces and bases of crystals of the mineral galena are congruent squares. Identify the shape of a galena crystal.

Answer: Cube

Explanation: The shape of the galena is Cube.

Question 8.
Rhianon draws the net below and labels each square. Can Rhianon fold her net into a cube that has letters A through G on its faces? Explain.

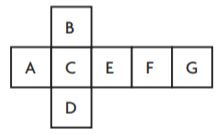
Answer: No, she cannot fold her net into a cube. Rhianon’s net has seven squares but there are only six squares in a net of a cube.

Question 9.
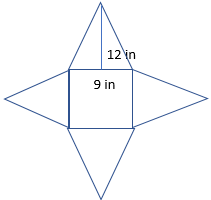
Describe A diamond crystal is shown. Describe the figure in terms of the solid figures you have seen in this lesson.

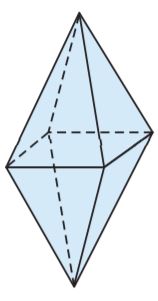
Answer: We can see that Diamond crystal consists of two square pyramids with congruent bases and the pyramids are reversed and placed base to base.

Question 10.
Sasha makes a triangular prism from paper.
The bases are _____.
The lateral faces are _____.

Answer:
The bases are Triangle
The lateral faces are Rectangle

Three-Dimensional Figures and Nets – Page No. 601

Identify and draw a net for the solid figure.

Question 1.

Answer: Rectangular Prism

Explanation:

Question 2.

Answer: Cube, Rectangular prism

Explanation:

Question 3.

Answer: Square Pyramid

Explanation:

Question 4.

Answer: Triangular Prism

Explanation:

Problem Solving

Question 5.
Hobie’s Candies are sold in triangular-pyramidshaped boxes. How many triangles are needed to make one box?

Answer: 4

Explanation: As triangled pyramids have four faces.

Question 6.
Nina used plastic rectangles to make 6 rectangular prisms. How many rectangles did she use?

Answer: 36

Explanation:

Question 7.
Describe how you could draw more than one net to represent the same three-dimensional figure. Give examples.

Answer:

Explanation:

Lesson Check – Page No. 602

Question 1.
How many vertices does a square pyramid have?

Answer: 5

Explanation:

Question 2.
Each box of Fred’s Fudge is constructed from 2 triangles and 3 rectangles. What is the shape of each box?

Answer: Triangular Prism

Explanation:

Spiral Review

Question 3.
Bryan jogged the same distance each day for 7 days. He ran a total of 22.4 miles. The equation 7d = 22.4 can be used to find the distance d in miles he jogged each day. How far did Bryan jog each day?

Answer: 3.2 miles

Explanation: As given equation 7d= 22.4,
d= 22.4÷7
= 3.2 miles.

Question 4.
A hot-air balloon is at an altitude of 240 feet. The balloon descends 30 feet per minute. What equation gives the altitude y, in feet, of the hot-air balloon after x minutes?

Answer: Y= 240- 30X.

Explanation: Given altitude Y, and the ballon was descended 30 feet per minute. So the equation is Y= 240- 30X.

Question 5.
A regular heptagon has sides measuring 26 mm and is divided into 7 congruent triangles. Each triangle has a height of 27 mm. What is the area of the heptagon?

Answer: 351 mm²

Explanation: Area of heptagon= 1/2 b×h
= 1/2 (26)×(27)
= 13×27
= 351 mm²

Question 6.
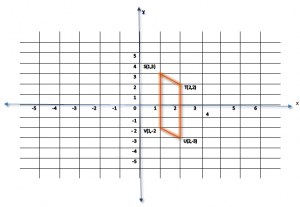
Alexis draws quadrilateral STUV with vertices S(1, 3), T(2, 2), U(2, –3), and V(1, –2). What name best classifies the quadrilateral?

Answer: Parallelogram

Explanation:

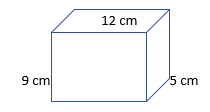
Share and Show – Page No. 605

Use the net to find the surface area of the prism.

Question 1.

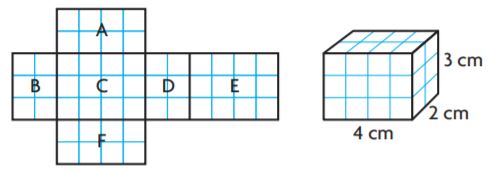
Answer:

Explanation: First we must find the area of each face
A= 4×3= 12
B= 4×3= 12
C= 5×4= 20
D= 5×4= 20
E= 5×3= 15
F= 5×3= 15
So, the surface area is 12+12+20+20+15+15= 94 cm²

Find the surface area of the rectangular prism.

Question 2.

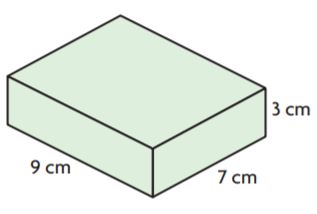
Answer: 222 cm²

Explanation: Area of a rectangular prism is 2(wl+hl+hw) = 2(7×9+ 3×9+ 3×7)
= 2(63+27+21)
= 2(111)
= 222 cm²

Question 3.

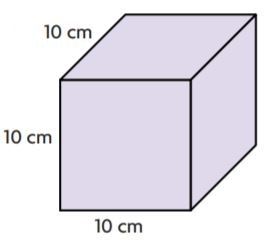
Answer:

Explanation: Area of a rectangular prism is 2(wl+hl+hw) = 2(10×10+ 10×10+ 10×10)
= 2(100+100+100)
= 2(300)
= 600 cm²

Question 4.

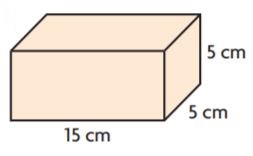
Answer: 350 cm²

Explanation: Area of a rectangular prism is 2(wl+hl+hw) = 2(15×5+ 5×5+ 15×5)
= 2(75+25+75)
= 2(175)
= 350 cm²

Problem Solving + Applications

Question 5.
A cereal box is shaped like a rectangular prism. The box is 20 cm long by 5 cm wide by 30 cm high. What is the surface area of the cereal box?

Answer: 1700 cm²

Explanation: The length of the box is 20 cm, the wide is 5 cm and the height is 30 cm. So surface area of the cereal box is 2(wl+hl+hw)= 2(20×5+30×20+30×5)
= 2(100+600+150)
= 2(850)
= 1700 cm²

Question 6.
Darren is painting a wooden block as part of his art project. The block is a rectangular prism that is 12 cm long by 9 cm wide by 5 cm high. Describe the rectangles that make up the net for the prism.

Answer:

Question 7.
In Exercise 6, what is the surface area, in square meters, that Darren has to paint?

Answer: 416 cm²

Explanation: Surface area = 2(wl+hl+hw)
= 2(9×12+5×12+ 5×9)
= 2(108+60+45)
= 2(213)
= 416 cm²

What’s the Error? – Page No. 606

Question 8.
Emilio is designing the packaging for a new MP3 player. The box for the MP3 player is 5 cm by 3 cm by 2 cm. Emilio needs to find the surface area of the box.
Look at how Emilio solved the problem. Find his error.
STEP 1: Draw a net.

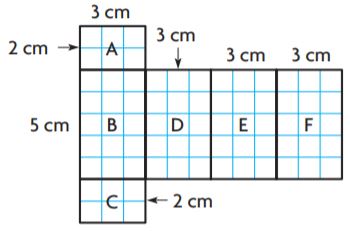
STEP 2: Find the areas of all the faces and add them.
Face A: 3 × 2 = 6 cm².
Face B: 3 × 5 = 15 cm².
Face C: 3 × 2 = 6 cm².
Face D: 3 × 5 = 15 cm².
Face E: 3 × 5 = 15 cm².
Face F: 3 × 5 = 15 cm².
The surface area is 6 + 15 + 6 + 15 + 15 + 15 = 72 cm².
Correct the error. Find the surface area of the prism.

Answer: Emilio drew the net incorrectly Face D and F should have been 2 cm by 5 cm, not 3 cm by 5 cm

Explanation:
Face A: 3×2= 6 cm²
Face B: 3×5= 15 cm²
Face C: 3×2= 6 cm²
Face D: 2×5= 10 cm²
Face E: 3×5= 15 cm²
Face F: 2×5= 10 cm²
So, the surface area of the prism area is 6+15+6+10+15+10= 62 cm^2.

Question 9.
For numbers 9a–9d, select True or False for each statement.

9a. The area of face A is 10 cm².
9b. The area of face B is 10 cm².
9c. The area of face C is 40 cm².
9d. The surface area of the prism is 66 cm².

9a. The area of face A is 10 cm².

Answer: True

Explanation: The area of face A is 2×5= 10 cm².

9b. The area of face B is 10 cm².

Answer: False

Explanation: The area of face B is 2×8= 16  cm².

9c. The area of face C is 40 cm².

Answer: The area of face C is 8×5= 40 cm².

9d. The surface area of the prism is 66 cm².

Answer: 160 cm².

Explanation: The surface area of the prism is
= 2×10+2×10+2×40
= 20+20+80
= 160 cm².

Explore Surface Area Using Nets – Page No. 607

Use the net to find the surface area of the rectangular prism.

Question 1.

_______ square units

Answer: 52 square units.

Explanation:
The area of face A is 6 squares.
The area of face B is 8 squares.
The area of face C is 6 squares.
The area of face D is 12 squares.
The area of face E is 8 squares.
The area of face F is 12 squares.
The surface area is 6+8+6+12+8+12= 52 square units.

Question 2.

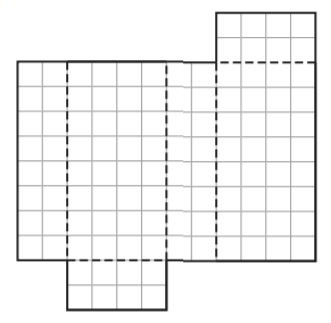
_______ square units

Answer: 112 square units.

Explanation:
The area of face A is 16 squares.
The area of face B is 8 squares.
The area of face C is 32 squares.
The area of face D is 16 squares.
The area of face E is 32 squares.
The area of face F is 8 squares.
The surface area is 112 square units.

Question 3.

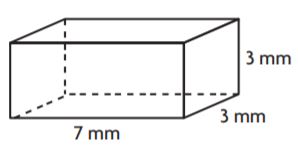
Answer: 102 mm²

Explanation: Area= 2(wl+hl+hw)
= 2(3×7+3×7+3×3)
= 2(21+21+9)
= 2(51)
= 102 mm²

Question 4.

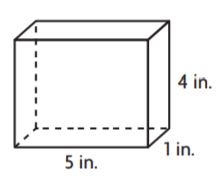
_______ in.²

Answer: 58 in.²

Explanation: Area= 2(wl+hl+hw)
= 2(5×1+ 4×1+ 4×5)
= 2(5+4+20)
= 2(29)
= 58 in.²

Question 5.

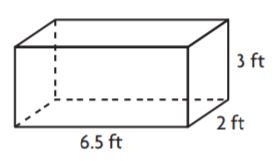
_______ ft²

Answer: 77 ft²

Explanation: Area= 2(wl+hl+hw)
= 2(6.5×2+3×2+3×6.5)
= 2(13+6+19.5)
= 2(38.5)
= 77 ft²

Problem Solving

Question 6.
Jeremiah is covering a cereal box with fabric for a school project. If the box is 6 inches long by 2 inches wide by 14 inches high, how much surface area does Jeremiah have to cover?
_______ in.²

Answer: 248 in.²

Explanation: Surface area of a cereal box is 2(wl+hl+hw)
= 2(2×6+14×6+14×2)
= 2(12+84+28)
= 2(124)
= 248 in.²

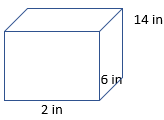
Question 7.
Tia is making a case for her calculator. It is a rectangular prism that will be 3.5 inches long by 1 inch wide by 10 inches high. How much material (surface area) will she need to make the case?
_______ in.²

Answer: 97 in.²

Explanation: Surface Area= 2(wl+hl+hw)
= 2(1×3.5+ 10×3.5+ 10×1)
= 2(3.5+35+10)
= 2(48.5)
= 97 in.²

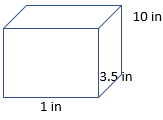
Question 8.
Explain in your own words how to find the surface area of a rectangular prism.

Answer: To find the surface area we must know the width, length, and height of the prism and then we can apply the formula which is
Surface area= 2(width ×length)+ 2(length×height)+ 2(height×width)
= 2(width ×length+ length×height+ 2(height×width)

Lesson Check – Page No. 608

Question 1.
Gabriela drew a net of a rectangular prism on centimeter grid paper. If the prism is 7 cm long by 10 cm wide by 8 cm high, how many grid squares does the net cover?
_______ cm²

Answer: 412 cm^2.

Explanation: Surface area is 2(wl+hl+hw)
= 2(10×7+8×7+8×10)
= 2(70+56+80)
= 2(206)
= 412 cm^2.

Question 2.
Ben bought a cell phone that came in a box shaped like a rectangular prism. The box is 5 inches long by 3 inches wide by 2 inches high. What is the surface area of the box?
_______ in.²

Answer: 62 in.²

Explanation: Surface area is 2(wl+hl+hw)
= 2(3×5+2×5+2×3)
= 2(15+10+6)
= 2(31)
= 62 in.²

Spiral Review

Question 3.
Katrin wrote the inequality x + 56 < 533. What is the solution of the inequality?

Answer: X<477.

Explanation: X+56<533
= X<533-56
= X<477.

Question 4.
The table shows the number of mixed CDs y that Jason makes in x hours.

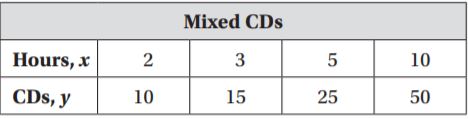
Which equation describes the pattern in the table?

Answer: y= 5x

Explanation:
y/x = 10/2= 15/4= 3
y= 5x
The pattern is y is x multipled by 5.

Question 5.
A square measuring 9 inches by 9 inches is cut from a corner of a square measuring 15 inches by 15 inches. What is the area of the L-shaped figure that is formed?
_______ in.²

Answer: 144 in.²

Explanation: The area of a square A= a², so we will find the area of each square.
Area= 9²
= 9×9
= 81 in.²
And the area of another square is
A= 15²
= 15×15
= 225 in.²
So the area of L shaped figure is 225-81= 144 in.²

Question 6.
Boxes of Clancy’s Energy Bars are rectangular prisms. How many lateral faces does each box have?

Answer: 4

Explanation: As Lateral faces are not included in the bases, so rectangular prism has 4.

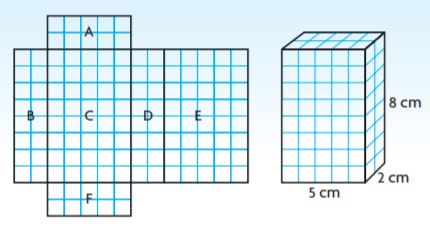
Share and Show – Page No. 611

Use a net to find the surface area.

Question 1.

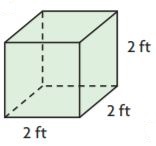
_______ ft²

Answer: 24 ft²

Explanation: The area of each face is 2 ft×2 ft= 4 ft and the number of faces is 6, so surface area is 6×4= 24 ft²

Question 2.

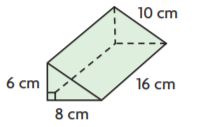
Answer: 432 cm²

Explanation:
The area of face A is 16×6= 96 cm²
The area of face B is 16×8= 128 cm²
The area of face C and D is 1/2 × 6×8= 24 cm²
The area of face E is 16×10= 160 cm²
The surface 96+128+2×24+160= 432 cm²

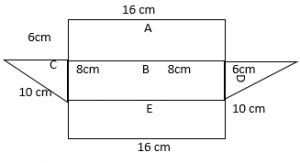
Question 3.

_______ in.²

Answer: 155.5 in.²

Explanation:

The area of face A and E is  8 ½ × 3½
= 17/2 × 7/2
= 119/4
= 29.75 in.²
The area of face B and F is 8 ½×4
= 17 ½ × 4
= 34 in.²
The area of face C and D is 3 ½×4
7/2 × 4= 14 in.²
The surface area is 2×29.75+2×34+2×14
= 59.5+68+28
= 155.5 in.²

On Your Own

Use a net to find the surface area.

Question 4.

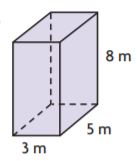
_______ m²

Answer:

Explanation:

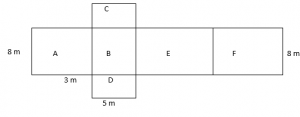
The area of face A and E is 8×3= 24 m²
The area of face B and F is 8×5= 40 m²
The area of face C and D is 3×5= 15 m²
The surface area is 2×24+2×40+2×15
= 48+80+30
= 158 m²

Question 5.

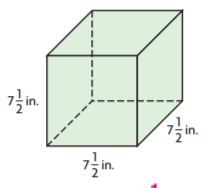
_______ \(\frac{□}{□}\) in.²

Answer:

Explanation:

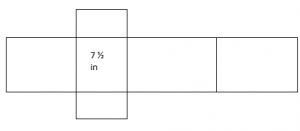
The area of each face is 7 1/2 × 7 1/2
= 15/2 × 15/2
= 225/4 in.²
The no.of faces are 6 and the surface area is 6× 225/4
= 675/4
= 337 1/2 in.²

Question 6.
Attend to Precision Calculate the surface area of the cube in Exercise 5 using the formula S = 6s². Show your work.

Answer: 337 1/2 in.²

Explanation: As S= s²
= 6(7 1/2)²
= 6(15/2)²
= 6(225/4)
= 675/2
= 337 1/2 in.²

Unlock the Problem – Page No. 612

Question 7.
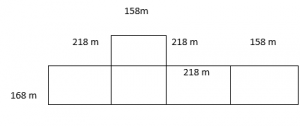
The Vehicle Assembly Building at Kennedy Space Center is a rectangular prism. It is 218 m long, 158 m wide, and 160 m tall. There are four 139 m tall doors in the building, averaging 29 m in width. What is the building’s outside surface area when the doors are open?
a. Draw each face of the building, not including the floor.

Answer:

Question 7.
b. What are the dimensions of the 4 walls?

Answer: The 2 walls measure 218 m ×160 m and 2 walls measure by 158 m×160 m.

Question 7.
c. What are the dimensions of the roof?

Answer: The dimensions of the roof are 218 m×158 m.

Question 7.
d. Find the building’s surface area (not including the floor) when the doors are closed.
_______ m²

Answer: 1,54,764 m²

Explanation:
The area of two walls is 218×160= 34,880 m²
The area of the other two walls is 158×160= 25,280 m²
The area of the roof 158×218= 34,444 m²
The surface area is 2× 34,880+ 2× 25,280+ 34,444
= 69,760+ 50,560+ 34,444
= 1,54,764 m²

Question 7.
e. Find the area of the four doors.
_______ m²

Answer: 16,124 m²

Explanation: Area of a door is 139×29 = 4031 m²
And the area of 4 doors is 4×4031= 16,124 m²

Question 7.
f. Find the building’s surface area (not including the floor) when the doors are open.
_______ m²

Answer: 1,38,640 m²

Explanation: The building’s surface area (not including the floor) when the doors are open is
1,54,764 – 16,124= 1,38,640 m²

Question 8.
A rectangular prism is 1 \(\frac{1}{2}\) ft long, \(\frac{2}{3}\) ft wide, and \(\frac{5}{6}\) ft high. What is the surface area of the prism in square inches?
_______ in.²

Answer: 808 in.²

Explanation: The area of two faces is 1 1/2× 5/6
= 3/2 × 5/6
= 5/4 cm²
The area of two faces is 2/3 × 5/6
= 5/9 ft²
The area of two faces is 1 1/2× 2/3
= 3/2 × 2/3
= 1 ft²
The surface area of the prism is 2(wl+hl+hw)
= 2(5/4 + 5/9 + 1)
= 2( 1.25+0.55+1)
= 2.5+1.1+2
= 5.61 ft²
As 1 square foot = 144 square inches
so 5.61×144 = 807.84
= 808 in.²

Question 9.
A gift box is a rectangular prism. The box measures 8 inches by 10 inches by 3 inches. What is its surface area?
_______ in.²

Answer: 268 in.²

Explanation:
The area of face A and Face E is 8×10= 80 in.²
The area of face B and Face F is 8×3= 24 in.²
The area of face C and Face D is 10×3= 30 in.²
The surface area is 2×80+2×24+2×30
= 160+48+60
= 268 in.²

Surface Area of Prisms – Page No. 613

Use a net to find the surface area.

Question 1.

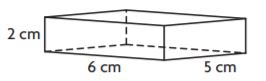
_______ cm²

Answer: 104 cm²

Explanation: Surface area= 2(wl+hl+hw)
= 2(6×5+2×5+2×6)
= 2(30+10+12)
= 2(52)
= 104 cm²

Question 2.

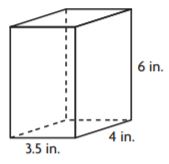
_______ in.²

Answer: 118 in.²

Explanation: Surface area= 2(wl+hl+hw)
= 2(3.5×4+6×4+6×3.5)
= 2(59)
= 118 in.²

Question 3.

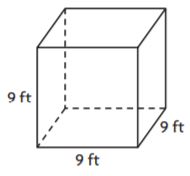
_______ ft²

Answer: 486 ft²

Explanation: Surface area= 2(wl+hl+hw)
= 2(9×9+9×9+9×9)
= 2(81+81+81)
= 2(243)
= 486 ft²

Question 4.

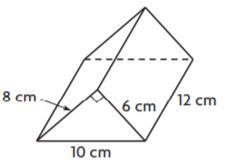
_______ cm²

Answer: 336 cm^2.

Explanation: Area = 1/2 bh
= 1/2 (6)(8)
= 3×8
= 24.
As there are 2 triangles, so 2×24= 48.
Surface Area= (wl+hl+hw)
= (6×12+8×12+12×10)
= 228
Total Surface area = 228+48
= 336 cm²

Problem Solving

Question 5.
A shoe box measures 15 in. by 7 in. by 4 \(\frac{1}{2}\) in. What is the surface area of the box?
_______ in.²

Answer: 408 in.²

Explanation:
The area of two faces is 15×7= 105 in.²
The area of two faces is 15× 4 1/2
= 15 × 9/2
= 15 × 4.5
= 67.5 in.²
The area of two faces is 7× 4 1/2
= 7× 9/2
= 7× 4.5
= 31.5 in.²
The surface area is 2×105+ 2×67.5+ 2×31.5
= 210+ 135+ 63
= 408 in.²

Question 6.
Vivian is working with a styrofoam cube for art class. The length of one side is 5 inches. How much surface area does Vivian have to work with?
_______ in.²

Answer: 150 in.²

Explanation:
The area of each face is 5×5= 25 in.²
The number of faces that styrofoam cube has is 6
So the surface area is 6×25= 150 in.²

Question 7.
Explain why a two-dimensional net is useful for finding the surface area of a three-dimensional figure.

Answer: Two-dimensional net is useful because by using a two-dimensional net you can calculate the surface area of each face and add them up to find the surface area of the three-dimensional figure.

Lesson Check – Page No. 614

Question 1.
What is the surface area of a cubic box that contains a baseball that has a diameter of 3 inches?
_______ in.²

Answer: 54 in.²

Explanation:
The area of each face is 3×3= 9 in.²
The number of faces for a cubic box is 6 in.²
The surface area of box that contains a baseball is 6×9= 54 in.²

Question 2.
A piece of wood used for construction is 2 inches by 4 inches by 24 inches. What is the surface area of the wood?
_______ in.²

Answer: 304 in.²

Explanation:
The area of two faces is 4×2= 8 in.²
The area of two faces is 2×24= 48 in.²
The area of two faces is 24×4= 96 in.²
So the surface area is 2×8+ 2×48+ 2×96
= 16+96+192
= 304 in.²

Spiral Review

Question 3.
Detergent costs $4 per box. Kendra graphs the equation that gives the cost y of buying x boxes of detergent. What is the equation?

Answer: Y= 4X.

Explanation: The total price Y and the price is equal to 4 × X, and X is the number of boxes that Kendra buys. So the equation is Y=4X.

Question 4.
A trapezoid with bases that measure 8 inches and 11 inches has a height of 3 inches. What is the area of the trapezoid?
_______ in.²

Answer: 28.5 in.²

Explanation:
Area of a trapezoid is 1/2(b1+b2)h
= 1/2(8+11)3
= 1/2(19)3
= 1/2 (57)
= 28.5 in.²

Question 5.
City Park is a right triangle with a base of 40 yd and a height of 25 yd. On a map, the park has a base of 40 in. and a height of 25 in. What is the ratio of the area of the triangle on the map to the area of City Park?

Answer: 1296:1.

Explanation:
Area= 1/2 bh
= 1/2 (40)(25)
= (20)(25)
= 500 yd²
So area of city park is 500 yd²
Area= 1/2 bh
= 1/2 (40)(25)
= (20)(25)
= 500 in²
So area on the map is 500 in
as 1 yd²= 1296 in²
So 500 in² = 500×1296
= 648,000
So, the ratio of the area of the triangle on the map to the area of City Park is 648,000:500
= 1296:1.

Question 6.
What is the surface area of the prism shown by the net?

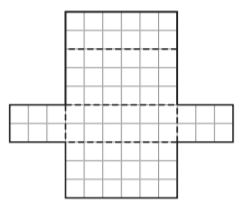
Answer: 72 square units.

Explanation:
The area of two faces is 18 squares
The area of two faces is 6 squares
The area of two faces is 12 squares
So the surface area is 2×18+ 2×6+ 2×12
= 72 square units.

Share and Show – Page No. 617

Question 1.
Use a net to find the surface area of the square pyramid.

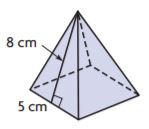
_______ cm²

Answer: 105 cm²

Explanation:

Area of the base 5×5= 25 ,
and area of one face is 1/2 × 5 × 8
= 5× 4
= 20 cm²
The surface area of a pyramid is 25+ 4×20
= 25+80
= 105 cm²

Question 2.
A triangular pyramid has a base with an area of 43 cm² and lateral faces with bases of 10 cm and heights of 8.6 cm. What is the surface area of the pyramid?
_______ cm²

Answer: 172 cm²

Explanation:
The area of one face is 1/2×10×8.6
= 5×8.6
= 43 cm²
The surface area of the pyramid is 43+3×43
= 43+ 129
= 172 cm²

Question 3.
A square pyramid has a base with a side length of 3 ft and lateral faces with heights of 2 ft. What is the lateral area of the pyramid?
_______ ft²

Answer: 12 ft²

Explanation:
The area of one face is 1/2×3×2= 3 ft²
The lateral area of the pyramid is 4×3= 12 ft²

On Your Own

Use a net to find the surface area of the square pyramid.

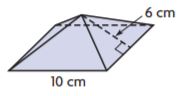
Question 4.

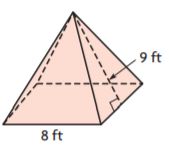
_______ ft²

Answer: 208 ft²

Explanation:

The area of the base is 8×8= 64
The area of one face is 1/2 ×8×9
= 36 ft²
The surface area of the pyramid is 64+4×36
= 64+144
= 208 ft²

Question 5.

_______ cm²

Answer: 220 cm²

Explanation:

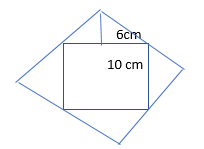
The area of base is 10×10= 100
The area of one place is 1/2×10×6
= 10×3
= 30
The surface area of the pyramid is 100+4×30
= 100+120
= 220 cm²

Question 6.

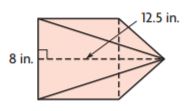
_______ in.²

Answer: 264 in.²

Explanation:

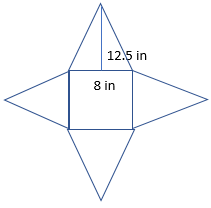
The area of the base is 8×8= 64
The area of one face is 1/2×8×12.5
= 4×12.5
= 50 in.²
The surface area of the pyramid is 64+ 4×50
= 64+200
= 264 in.²

Question 7.
The Pyramid Arena is located in Memphis, Tennessee. It is in the shape of a square pyramid, and the lateral faces are made almost completely of glass. The base has a side length of about 600 ft and the lateral faces have a height of about 440 ft. What is the total area of the glass in the Pyramid Arena?
_______ ft²

Answer: 5,28,000 ft²

Explanation:
The area of one face is 1/2×600×440= 1,32,000 ft²
The surface of tha lateral faces is 4× 1,32,000= 5,28,000 ft²
So, the total area of the glass in the arena is 5,28,000 ft²

Problem Solving + Applications – Page No. 618

Use the table for 8–9.

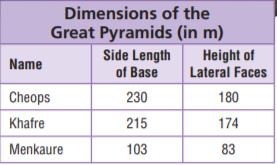
Question 8.
The Great Pyramids are located near Cairo, Egypt. They are all square pyramids, and their dimensions are shown in the table. What is the lateral area of the Pyramid of Cheops?
_______ m²

Answer: 82,800 m²

Explanation:
The area of one face is 1/2×230×180
= 230×90
= 20,700 m²
The lateral area of the pyramid of Cheops is 4×20,700= 82,800 m²

Question 9.
What is the difference between the surface areas of the Pyramid of Khafre and the Pyramid of Menkaure?
_______ m²

Answer: 93,338 m²

Explanation:
The area of the base is 215×215= 46,225
The area of one face is 1/2×215×174
= 215× 87
18,705 m²
The surface area of Pyramid Khafre is= 46,225+4×18,705
= 46,225+ 74820
= 121,045 m²
The area of the base 103×103= 10,609
The area of one face is 1/2×103×83
= 8549÷2
= 4274.4 m²
The surface area of the Pyramid of Menkaure is 10,609+4×4274.5
= 10,609+ 17,098
= 27,707 m²

The difference between the surface areas of the Pyramid of Khafre and the Pyramid of Menkaure
= 121,405-27,707
= 93,338 m²

Question 10.
Write an expression for the surface area of the square pyramid shown.

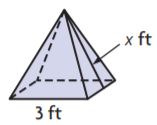
Answer: 6x+9 ft^2.

Explanation: The expression for the surface area of the square pyramid is 6x+9 ft^2.

Question 11.
Make Arguments A square pyramid has a base with a side length of 4 cm and triangular faces with a height of 7 cm. Esther calculated the surface area as (4 × 4) + 4(4 × 7) = 128 cm². Explain Esther’s error and find the correct surface area

Answer: 72 cm².

Explanation: Esther didn’t apply the formula correctly, she forgot to include 1/2 in the calculated surface area.
The correct surface area is (4×4)+4(1/2 ×4×7)
= 16+4(14)
= 16+56
= 72 cm².

Question 12.
Jose says the lateral area of the square pyramid is 260 in.². Do you agree or disagree with Jose? Use numbers and words to support your answer.

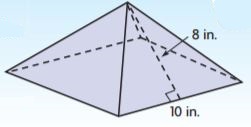
Answer: 160 in.²

Explanation: No, I disagree with Jose as he found surface area instead of the lateral area, so the lateral area is
4×1/2×10×8
= 2×10×8
= 160 in.²

Surface Area of Pyramids – Page No. 619

Use a net to find the surface area of the square pyramid.

Question 1.

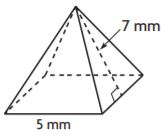
_______ mm²

Answer: 95 mm²

Explanation:

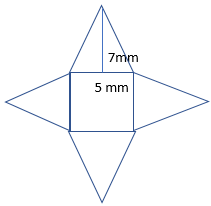
The area of the base is 5×5= 25 mm²
The area of one face is 1/2×5×7
= 35/2
= 17.5 mm²
The surface area is 25+4×17.5
= 25+4×17.5
= 25+70
= 95 mm²

Question 2.

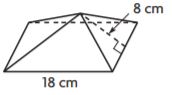
_______ cm²

Answer: 612 cm²

Explanation:

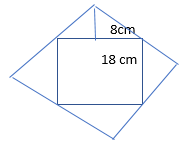
The area of the base is 18×18= 324 cm²
The area of one face is 1/2×18×8
= 18×4
=  72 cm²
The surface area is 324+4×72
= 25+4×17.5
= 25+70
= 612 cm²

Question 3.

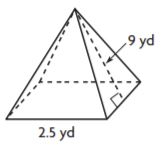
_______ yd²

Answer: 51.25 yd²

Explanation:

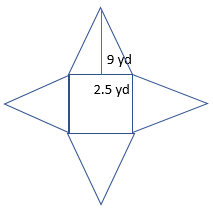
The area of the base is 2.5×2.5= 6.25  mm²
The area of one face is 1/2×2.5×9
= 22.5/2
= 11.25 yd²
The surface area is 25+4×17.5
= 6.25+4×11.25
= 6.25+45
= 51.25 yd²

Question 4.

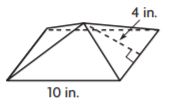
_______ in.²

Answer: 180 in²

Explanation:

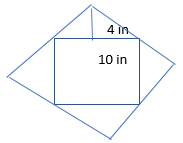
The area of the base is 10×10= 100 in²
The area of one face is 1/2×4×10
= 2×10
= 20 in²
The surface area is 100+4×20
= 100+4×20
= 100+80
= 180 in²

Problem Solving

Question 5.
Cho is building a sandcastle in the shape of a triangular pyramid. The area of the base is 7 square feet. Each side of the base has a length of 4 feet and the height of each face is 2 feet. What is the surface area of the pyramid?
_______ ft²

Answer: 19 ft²

Explanation:
The area of one face is 1/2×4×2= 4 ft²
The surface area of the triangular pyramid is 7+3×4
= 7+12
= 19 ft²

Question 6.
The top of a skyscraper is shaped like a square pyramid. Each side of the base has a length of 60 meters and the height of each triangle is 20 meters. What is the lateral area of the pyramid?
_______ m²

Answer: 2400 m²

Explanation:
The area of the one face is 1/2×60×20
= 600 m²
The lateral area of the pyramid is 4×600= 2400 m²

Question 7.
Write and solve a problem finding the lateral area of an object shaped like a square pyramid.

Answer: Mary has a triangular pyramid with a base of 10cm and a height of 15cm. What is the lateral area of the pyramid?

Explanation:
The area of one face is 1/2×10×15
= 5×15
= 75 cm²
The lateral area of the triangular pyramid is 3×75
= 225 cm²

Lesson Check – Page No. 620

Question 1.
A square pyramid has a base with a side length of 12 in. Each face has a height of 7 in. What is the surface area of the pyramid?
_______ in.²

Answer: 312 in.²

Explanation:
The area of the base is 12×12= 144 in.²
The area of one face is 1/2×12×7
= 6×7
= 42 in.²
The surface area of the square pyramid is 144+4×42
= 144+ 168
= 312 in.²

Question 2.
The faces of a triangular pyramid have a base of 5 cm and a height of 11 cm. What is the lateral area of the pyramid?
_______ cm²

Answer: 82.5 cm²

Explanation:
The area of one face is 1/2×5×11
= 55/2
= 27.5 cm²
The lateral area of the triangular pyramid is 3×27.5= 82.5 cm²

Spiral Review

Question 3.
What is the linear equation represented by the graph?

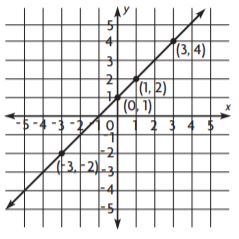
Answer: y=x+1.

Explanation: As the figure represents that every y value is 1 more than the corresponding x value, so the linear equation is y=x+1.

Question 4.
A regular octagon has sides measuring about 4 cm. If the octagon is divided into 8 congruent triangles, each has a height of 5 cm. What is the area of the octagon?
_______ cm²

Answer:

Explanation:
Area is 1/2bh
= 1/2× 4×5
= 2×5
= 10 cm²
So the area of each triangle is 10 cm²
and the area of the octagon is 8×10= 80 cm²

Question 5.
Carly draws quadrilateral JKLM with vertices J(−3, 3), K(3, 3), L(2, −1), and M(−2, −1). What is the best way to classify the quadrilateral?

Answer: It is a Trapezoid.

Explanation: It is a Trapezoid.

Question 6.
A rectangular prism has the dimensions 8 feet by 3 feet by 5 feet. What is the surface area of the prism?
_______ ft²

Answer: 158 ft²

Explanation:
The area of the two faces of the rectangular prism is 8×3= 24 ft²
The area of the two faces of the rectangular prism is 8×5= 40 ft²
The area of the two faces of the rectangular prism is 3×5= 15 ft²
The surface area of the rectangular prism is 2×24+2×40+2×15
= 48+80+30
= 158 ft²

Mid-Chapter Checkpoint – Vocabulary – Page No. 621

Choose the best term from the box to complete the sentence.

Question 1.
_____ is the sum of the areas of all the faces, or surfaces, of a solid figure.

Answer: Surface area is the sum of the areas of all the faces, or surfaces, of a solid figure.

Question 2.
A three-dimensional figure having length, width, and height is called a(n) _____.

Answer: A three-dimensional figure having length, width, and height is called a(n) solid figure.

Question 3.
The _____ of a solid figure is the sum of the areas of its lateral faces.

Answer: The lateral area of a solid figure is the sum of the areas of its lateral faces.

Concepts and Skills

Question 4.
Identify and draw a net for the solid figure.

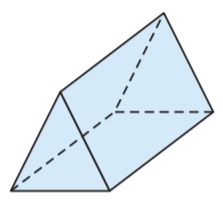
Answer: Triangular prism

Explanation:

Question 5.
Use a net to find the lateral area of the square pyramid.

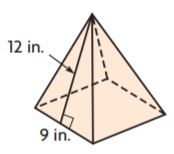
_______ in.²

Answer: 216 in.²

Explanation:

The area of one face is 1/2×9×12
= 9×6
= 54 in.²
The lateral area of the square pyramid is 4×54= 216 in.²

Question 6.
Use a net to find the surface area of the prism.

_______ cm²

Answer: 310 cm²

Explanation:

The area of face A and E is 10×5= 50 cm²
The area of face B and F is 10×7= 70 cm²
The area of face C and D is 7×5= 35 cm²
The surface area of the prism is 2×50+2×70+2×35
= 100+140+70
= 310 cm²

Page No. 622

Question 7.
A machine cuts nets from flat pieces of cardboard. The nets can be folded into triangular pyramids used as pieces in a board game. What shapes appear in the net? How many of each shape are there?

Answer: 4 triangles.

Explanation: There are 4 triangles.

Question 8.
Fran’s filing cabinet is 6 feet tall, 1 \(\frac{1}{3}\) feet wide, and 3 feet deep. She plans to paint all sides except the bottom of the cabinet. Find the area of the sides she intends to paint.
_______ ft²

Answer: 56 ft²

Explanation:
The two lateral face area is 6×1 1/3
= 6× 4/3
= 2×4
= 8 ft²
The area of the other two lateral faces is 6×3= 18
The area of the top and bottom is 3× 1 1/3
= 3× 4/3
= 4 ft²
The area of the sides she intends to paint is 2×8+2×18+4
= 16+36+4
= 56 ft²

Question 9.
A triangular pyramid has lateral faces with bases of 6 meters and heights of 9 meters. The area of the base of the pyramid is 15.6 square meters. What is the surface area of the pyramid?

Answer: 96.6 m²

Explanation:
The area of one face is 1/2× 6× 9
= 3×9
= 27 m²
The surface area of the triangular pyramid is 15.6+3×27
= 15.6+ 81
= 96.6 m²

Question 10.
What is the surface area of a storage box that measures 15 centimeters by 12 centimeters by 10 centimeters?
_______ cm²

Answer: 900 cm²

Explanation:
The area of two faces is 15×12= 180 cm²
The area of another two faces is 15×10= 150 cm²
The area of the other two faces is 10×12= 120 cm²
So surface area of the storage box is 2×180+2×150+2×120 cm²
= 360+300+240
= 900 cm²

Question 11.
A small refrigerator is a cube with a side length of 16 inches. Use the formula S = 6s² to find the surface area of the cube.
_______ in.²

Answer: 1,536 in.²

Explanation:
Area = s²
= 6×(16)²
= 6× 256
= 1,536 in.²

Share and Show – Page No. 625

Question 1.
A prism is filled with 38 cubes with a side length of \(\frac{1}{2}\) unit. What is the volume of the prism in cubic units?
_______ \(\frac{□}{□}\) cubic units

Answer: 4.75 cubic units

Explanation:
The volume of the cube is S³
The volume of a cube with S= (1/2)³
= 1/2×1/2×1/2
= 1/8
= 0.125 cubic units
As there are 38 cubes so 38×0.125= 4.75 cubic units.

Question 2.
A prism is filled with 58 cubes with a side length of \(\frac{1}{2}\) unit. What is the volume of the prism in cubic units?
_______ \(\frac{□}{□}\) cubic units

Answer: 7.25 cubic units.

Explanation:
The volume of the cube is S³
The volume of a cube with S= (1/2)³
= 1/2×1/2×1/2
= 1/8
= 0.125 cubic units
As there are 58 cubes so 58×0.125= 7.25 cubic units.

Find the volume of the rectangular prism.

Question 3.

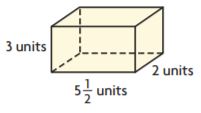
_______ cubic units

Answer: 33 cubic units.

Explanation:
The volume of the rectangular prism is= Width×Height×Length
= 5 1/2 ×3×2
= 11/2 ×3×2
= 33 cubic units.

Question 4.

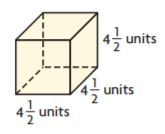
_______ \(\frac{□}{□}\) cubic units

Answer: 91 1/8 cubic units.

Explanation:
The volume of the rectangular prism is= Width×Height×Length
= 4 1/2 ×4 1/2×4 1/2
= 9/2 ×9/2×9/2
= 729/8
= 91 1/8 cubic units.

Question 5.
Theodore wants to put three flowering plants in his window box. The window box is shaped like a rectangular prism that is 30.5 in. long, 6 in. wide, and 6 in. deep. The three plants need a total of 1,200 in.³ of potting soil to grow well. Is the box large enough? Explain.

Answer: No, the box is not large enough as the three plants need a total of 1,200 in.³ and here volume is 1,098 in.³

Explanation:
Volume= Width×Height×Length
= 30.5×6×6
= 1,098 in.³

Question 6.
Explain how use the formula V = l × w × h to verify that a cube with a side length of \(\frac{1}{2}\) unit has a volume of \(\frac{1}{8}\) of a cubic unit.

Answer: 1/8 cubic units

Explanation:
As length, width and height is 1/2′ so
Volume = Width×Height×Length
= 1/2 × 1/2 × 1/2
= 1/8 cubic units

Problem Solving + Applications – Page No. 626

Use the diagram for 7–10.

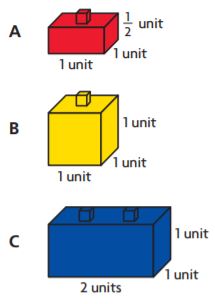
Question 7.
Karyn is using a set of building blocks shaped like rectangular prisms to make a model. The three types of blocks she has are shown at right. What is the volume of an A block? (Do not include the pegs on top.)
\(\frac{□}{□}\) cubic units

Answer: 1/2 cubic units

Explanation: Volume = Width×Height×Length
= 1× 1/2 ×1
= 1/2 cubic units

Question 8.
How many A blocks would you need to take up the same amount of space as a C block?
_______ A blocks

Answer: No of blocks required to take up the same amount of space as a C block is 4 A blocks.

Explanation: Volume = Width×Height×Length
= 1×2×1
= 2 cubic unit
No of blocks required to take up the same amount of space as a C block is 1/2 ÷2
= 2×2
= 4 A blocks

Question 9.
Karyn puts a B block, two C blocks, and three A blocks together. What is the total volume of these blocks?
_______ \(\frac{□}{□}\) cubic units

Answer: 6 1/2 cubic units

Explanation: The volume of A block is
Volume = Width×Height×Length
= 1×1 ×1/2
= 1/2 cubic units.
As Karyn puts three A blocks together, so 3× 1/2= 3/2 cubic units.
The volume of B block is
Volume = Width×Height×Length
= 1×1 × 1
= 1 cubic units.
As Karyn puts only one B, so 1 cubic unit.
The volume of C block is
Volume = Width×Height×Length
= 2×1×1
= 2 cubic units.
As Karyn puts two C blocks together, so 2× 2= 4 cubic units.
So, the total volume of these blocks is 3/2 + 1+ 4
= 3/2+5
= 13/2
= 6 1/2 cubic units

Question 10.
Karyn uses the blocks to make a prism that is 2 units long, 3 units wide, and 1 \(\frac{1}{2}\) units high. The prism is made of two C blocks, two B blocks, and some A blocks. What is the total volume of A blocks used?
_______ cubic units

Answer: 3 cubic units.

Explanation:
Volume = Width×Height×Length
= 2×3×1 1/2
= 2×3× 3/2
= 9 cubic units.
The total volume of A block used is 9-(2×2)-(2×1)
= 9- 4- 2
= 9-6
= 3 cubic units.

Question 11.
Verify the Reasoning of Others Jo says that you can use V = l × w × h or V = h × w × l to find the volume of a rectangular prism. Does Jo’s statement make sense? Explain.

Answer: Yes

Explanation: Yes, Jo’s statement makes sense because by the commutative property we can change the order of the variables of length, width, height and both will produce the same result.

Question 12.
A box measures 5 units by 3 units by 2 \(\frac{1}{2}\) units. For numbers 12a–12b, select True or False for the statement.
12a. The greatest number of cubes with a side length of \(\frac{1}{2}\) unit that can be packed inside the box is 300.
12b. The volume of the box is 37 \(\frac{1}{2}\) cubic units.
12a. __________
12b. __________

Answer:
12a True.
12b True.

Explanation: The volume of the cube is S³
The volume of a cube with S= (1/2)³
= 1/2×1/2×1/2
= 1/8 cubic units
As there are 300 cubes so 300× 1/8= 75/2
= 37 1/2 cubic units.

Fractions and Volume – Page No. 627

Find the volume of the rectangular prism.

Question 1.

_______ \(\frac{□}{□}\) cubic units

Answer: 6 3/4 cubic units

Explanation: Volume = Width×Height×Length
= 3× 1 1/2× 1 1/2
= 3× 3/2 × 3/2
= 27/4
= 6 3/4 cubic units

Question 2.

_______ \(\frac{□}{□}\) cubic units

Answer: 22 1/2 cubic units

Explanation: Volume = Width×Height×Length
= 5×1× 4 1/2
= 5× 9/2
= 45/2
= 22 1/2 cubic units

Question 3.

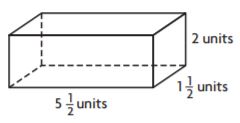
_______ \(\frac{□}{□}\) cubic units

Answer: 16 1/2 cubic units.

Explanation: Volume = Width×Height×Length
= 5 1/2× 1 1/2× 2
= 11/2×3/2×2
= 33/2
= 16 1/2 cubic units.

Question 4.

_______ \(\frac{□}{□}\) cubic units

Answer: 28 1/8 cubic units.

Explanation: Volume = Width×Height×Length
= 2 1/2× 2 1/2 × 4 1/2
= 5/2 × 5/2 × 9/2
= 225/8
= 28 1/8 cubic units.

Problem Solving

Question 5.
Miguel is pouring liquid into a container that is 4 \(\frac{1}{2}\) inches long by 3 \(\frac{1}{2}\) inches wide by 2 inches high. How many cubic inches of liquid will fit in the container?
_______ \(\frac{□}{□}\) in.³

Answer: 31 1/2 cubic units

Explanation: Volume = Width×Height×Length
= 4 1/2 × 3 1/2 ×2
= 9/2 × 7/2 × 2
= 63/2
= 31 1/2 cubic units

Question 6.
A shipping crate is shaped like a rectangular prism. It is 5 \(\frac{1}{2}\) feet long by 3 feet wide by 3 feet high. What is the volume of the crate?
_______ \(\frac{□}{□}\) ft³

Answer: 49 1/2 ft³

Explanation: Volume = Width×Height×Length
= 5 1/2 × 3 × 3
= 11/2 ×9
= 99/2
= 49 1/2 ft³

Question 7.
How many cubes with a side length of \(\frac{1}{4}\) unit would it take to make a unit cube? Explain how you determined your answer.

Answer: There will be 4×4×4= 64 cubes and 1/4 unit in the unit cube.

Explanation:
As the unit cube has a 1 unit length, 1 unit wide, and 1 unit height
So length 4 cubes = 4× 1/4= 1 unit
width 4 cubes = 4× 1/4= 1 unit
height 4 cubes = 4× 1/4= 1 unit
So there will be 4×4×4= 64 cubes and 1/4 unit in the unit cube.

Lesson Check – Page No. 628

Question 1.
A rectangular prism is 4 units by 2 \(\frac{1}{2}\) units by 1 \(\frac{1}{2}\) units. How many cubes with a side length of \(\frac{1}{2}\) unit will completely fill the prism?

Answer: 120 cubes

Explanation:
No of cubes with a side length of 1/2 unit is
Length 8 cubes= 8× 1/2= 4 units
Width 5 cubes= 5× 1/2= 5/2= 2 1/2 units
Height 3 cubes= 3× 1/2= 3/2= 1 1/2 units
So there are 8×5×3= 120 cubes in the prism.

Question 2.
A rectangular prism is filled with 196 cubes with \(\frac{1}{2}\)-unit side lengths. What is the volume of the prism in cubic units?
_______ \(\frac{□}{□}\) cubic units

Answer: 24 1/2 cubic units.

Explanation: As it takes 8 cubes with a side length of 1/2 to form a unit cube, so the volume of the prism in the cubic units is 196÷8= 24 1/2 cubic units.

Spiral Review

Question 3.
A parallelogram-shaped piece of stained glass has a base measuring 2 \(\frac{1}{2}\) inches and a height of 1 \(\frac{1}{4}\) inches. What is the area of the piece of stained glass?
_______ \(\frac{□}{□}\) in.²

Answer: 3 1/8 in.²

Explanation: Area of a parallelogram = base×height
= 2 1/2 × 1 1/4
= 5/2 × 5/4
= 25/8
= 3 1/8 in.²

Question 4.
A flag for the sports club is a rectangle measuring 20 inches by 32 inches. Within the rectangle is a yellow square with a side length of 6 inches. What is the area of the flag that is not part of the yellow square?
_______ in.²

Answer: 604 in.²

Explanation: Area of a flag= Length×width
= 20×32
= 640 in.²
Area of the yellow square= S²
= 6
= 36 in.²
So the area of the flag that is not a part of the yellow square is 640-36= 604 in.²

Question 5.
What is the surface area of the rectangular prism shown by the net?

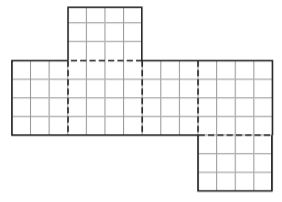
_______ square units

Answer: 80 square units

Explanation:
Area of two faces is 12 squares
Area of other two faces is 16 squares
Area of another two faces is 12 squares
So the surface area is 2×12+2×16+2×12
= 24+32+24
= 80 square units

Question 6.
What is the surface area of the square pyramid?

_______ cm²

Answer: 161 cm²

Explanation: The area of the base is 7×7= 49 cm²
And the area of one face is 1/2 × 7× 8
= 7×4
= 28 cm²
The surface area of the square pyramid is 49+4×28
= 49+112
= 161 cm²

Share and Show – Page No. 631

Find the volume.

Question 1.

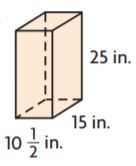
_______ \(\frac{□}{□}\) in.³

Answer: 3,937 1/2 in.³

Explanation: Volume= Length× wide× heght
= 10 1/2 ×15 × 25
= 11/2 × 15 × 25
= 4,125/2
= 3,937 1/2 in.³

Question 2.

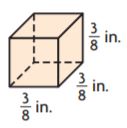
_______ \(\frac{□}{□}\) in.³

Answer: 27/512 in.³

Explanation: Volume= Length× wide× height
=3/8 ×3/8 × 3/8
= 27/512 in.³

On Your Own

Find the volume of the prism.

Question 3.

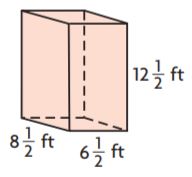
_______ \(\frac{□}{□}\) in.³

Answer: 690 5/8in.³

Explanation: Volume= Length× wide× height
= 8 1/2 × 6 1/2 × 12 1/2
= 17/2 × 13/2× 25/2
= 5525/2
= 690 5/8in.³

Question 4.

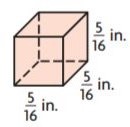
_______ \(\frac{□}{□}\) in.³

Answer: 125/4096 in.³

Explanation: Volume= Length× wide× height
= 5/16 ×5/16 × 5/16
= 125/4096 in.³

Question 5.

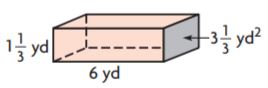
_______ yd³

Answer: 20 yd³

Explanation:
Area= 3 1/3 yd²
So Area= wide×height
3 1/3= w × 1 1/3
10/3= w× 4/3
w= 10/3 × 3/4
w= 5/2
w= 2.5 yd
Volume= Length×width×height
= 6× 2.5× 1 1/3
= 6×2.5× 4/3
= 2×2.5×4
= 20 yd³

Question 6.
Wayne’s gym locker is a rectangular prism with a width and height of 14 \(\frac{1}{2}\) inches. The length is 8 inches greater than the width. What is the volume of the locker?
_______ \(\frac{□}{□}\) in.³

Answer: 4,730 5/8 in.³

Explanation: As length is 8 inches greater than width, so 14 1/2+ 8
= 29/2+8
= 45/2
= 22 1/2 in
Then volume= Length×width×height
= 22 1/2 × 14 1/2 × 14 1/2
= 45/2× 29/2× 29/2
= 37845/8
= 4,730 5/8 in.³

Question 7.
Abraham has a toy box that is in the shape of a rectangular prism.

The volume is _____.
_______ \(\frac{□}{□}\) ft³

Answer: 33 3/4 ft³

Explanation: Volume of rectangular prism is= Length×width×height
= 4 1/2× 2 1/2× 3
= 9/2 × 5/2× 3
= 135/3
= 33 3/4 ft³

Aquariums – Page No. 632

Large public aquariums like the Tennessee Aquarium in Chattanooga have a wide variety of freshwater and saltwater fish species from around the world. The fish are kept in tanks of various sizes.
The table shows information about several tanks in the aquarium. Each tank is a rectangular prism.

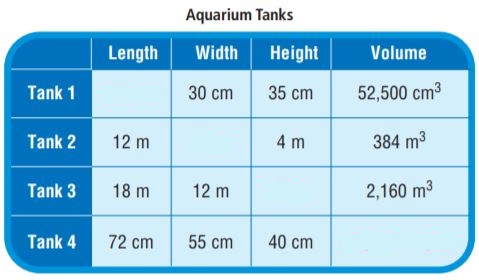
Find the length of Tank 1.
V = l w h
52,500 = l × 30 × 35
\(\frac{52,500}{1,050}\) = l
50 = l
So, the length of Tank 1 is 50 cm.

Solve.

Question 8.
Find the width of Tank 2 and the height of Tank 3.

Answer: Width of Tank 2= 8m, Height of the Tank 3= 10 m

Explanation:
The volume of Tank 2= 384 m³
so V= LWH
384=  12×W×4
W= 384/48
W= 8 m
So the width of Tank 2= 8m
The volume of Tank 3= 2160 m
So V= LWH
2160= 18×12×H
H= 2160/216
H= 10 m
So the height of the Tank 3= 10 m

Question 9.
To keep the fish healthy, there should be the correct ratio of water to fish in the tank. One recommended ratio is 9 L of water for every 2 fish. Find the volume of Tank 4. Then use the equivalencies 1 cm³ = 1 mL and 1,000 mL = 1 L to find how many fish can be safely kept in Tank 4.

Answer: 35 Fishes

Explanation:
Volume of Tank 4 = LWH
= 72×55×40
= 1,58,400 cm³
As 1 cm³ = 1 mL and 1,000 mL = 1 L
1,58,400 cm³ = 1,58,400 mL and 1,58,400 mL = 158.4 L
So tank can keep safely (158.4÷ 9)×2
= (17.6)× 2 = 35.2
= 35 Fishes

Question 10.
Use Reasoning Give another set of dimensions for a tank that would have the same volume as Tank 2. Explain how you found your answer.

Answer: Another set of dimensions for a tank that would have the same volume as Tank 2 is 8m by 8m by 6m.
So when we multiply the product will be 384

Volume of Rectangular Prisms – Page No. 633

Find the volume.

Question 1.

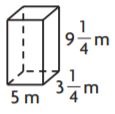
_______ \(\frac{□}{□}\) m³

Answer: 150 5/16 m³

Explanation: Volume= Length×width×height
= 5× 3 1/4× 9 1/4
= 5× 13/4 × 37/4
= 2405/16
= 150 5/16 m³

Question 2.

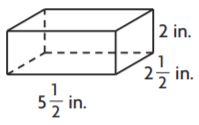
_______ \(\frac{□}{□}\) in.³

Answer: 27 1/2 in.³

Explanation: Volume= Length×width×height
= 5 1/2 × 2 1/2 × 2
= 11/2 × 5/2 × 2
= 55/2
= 27 1/2 in.³

Question 3.

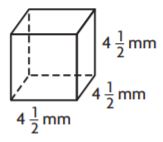
_______ \(\frac{□}{□}\) mm³

Answer: 91 1/8 mm³

Explanation: Volume= Length×width×height
= 4 1/2 × 4 1/2 × 4 1/2
= 9/2 × 9/2 × 9/2
= 729/8
= 91 1/8 mm³

Question 4.

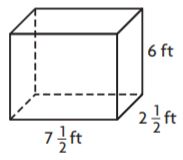
_______ \(\frac{□}{□}\) ft³

Answer: 112 1/2 ft³

Explanation: Volume= Length×width×height
= 7 1/2 × 2 1/2 × 6
= 15/2 × 5/2 × 6
= 225/2
= 112 1/2 ft³

Question 5.

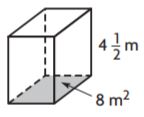
_______ m³

Answer: 36 m³

Explanation:
The area of shaded face is Length × width= 8 m²
Volume of the prism= Length×width×height
= 8 × 4 1/2
= 8 × 9/2
= 4 × 9
= 36 m³

Question 6.

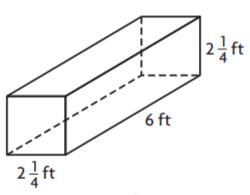
_______ \(\frac{□}{□}\) ft³

Answer: 30 3/8 ft³

Explanation: Volume of the prism= Length×width×height
= 2 1/4 × 6 × 2 1/4
= 9/4 × 6 × 9/4
= 243/8
= 30 3/8 ft³

Problem Solving

Question 7.
A cereal box is a rectangular prism that is 8 inches long and 2 \(\frac{1}{2}\) inches wide. The volume of the box is 200 in.³. What is the height of the box?
_______ in.

Answer: H= 10 in

Explanation: As volume = 200 in.³. So
V= LWH
200= 8 × 2 1/2 × H
200= 8 × 5/2 × H
200= 20 × H
H= 10 in

Question 8.
A stack of paper is 8 \(\frac{1}{2}\) in. long by 11 in. wide by 4 in. high. What is the volume of the stack of paper?
_______ in.³

Answer: 374 in.³

Explanation: The volume of the stack of paper= LWH
= 8 1/2 × 11 × 4
= 17/2 × 11 × 4
= 374 in.³

Question 9.
Explain how you can find the side length of a rectangular prism if you are given the volume and the two other measurements. Does this process change if one of the measurements includes a fraction?

Answer: We can find the side length of a rectangular prism if you are given the volume and the two other measurements by dividing the value of the volume by the product of the values of width and height of the prism. And the process doesn’t change if one of the measurements include a fraction.

Lesson Check – Page No. 634

Question 1.
A kitchen sink is a rectangular prism with a length of 19 \(\frac{7}{8}\) inches, a width of 14 \(\frac{3}{4}\) inches, and height of 10 inches. Estimate the volume of the sink.

Answer: 3,000 in.³

Explanation: Length = 19 7/8 as the number was close to 20 and width 14 3/4 which is close to 15 and height is 10
So Volume= LBH
= 20 × 15 × 10
= 3,000 in.³

Question 2.
A storage container is a rectangular prism that is 65 centimeters long and 40 centimeters wide. The volume of the container is 62,400 cubic centimeters. What is the height of the container?

Answer: H= 24 cm

Explanation: Volume of container= LBH
Volume= 62,400 cubic centimeters
62,400 = 65× 40 × H
62,400 = 2600 × H
H= 62,400/ 2600
H= 24 cm

Spiral Review

Question 3.
Carrie started at the southeast corner of Franklin Park, walked north 240 yards, turned and walked west 80 yards, and then turned and walked diagonally back to where she started. What is the area of the triangle enclosed by the path she walked?
_______ yd²

Answer: 9,600 yd²

Explanation:
Area of triangle= 1/2 bh
= 1/2 × 240 × 80
= 240 × 40
= 9,600 yd²

Question 4.
The dimensions of a rectangular garage are 100 times the dimensions of a floor plan of the garage. The area of the floor plan is 8 square inches. What is the area of the garage?

Answer: 80,000 in²

Explanation: As 1 in²= 10,000 in², so area of the floor plan 8 in
= 8×10000
= 80,000 in²

Question 5.
Shiloh wants to create a paper-mâché box shaped like a rectangular prism. If the box will be 4 inches by 5 inches by 8 inches, how much paper does she need to cover the box?

Answer: 184 in²

Explanation: Area of the rectangular prism= 2(wl+hl+hw)
= 2(4×5 + 5×8 + 8×4)
= 2(20+40+32)
= 2(92)
= 184 in²

Question 6.
A box is filled with 220 cubes with a side length of \(\frac{1}{2}\) unit. What is the volume of the box in cubic units?
_______ \(\frac{□}{□}\) cubic units

Answer: 27.5 cubic units.

Explanation: The volume of a cube side is (1/2)³ = 1/8
So 220 cubes= 220× 1/8
= 27.5 cubic units.

Share and Show – Page No. 637

Question 1.
An aquarium tank in the shape of a rectangular prism is 60 cm long, 30 cm wide, and 24 cm high. The top of the tank is open, and the glass used to make the tank is 1 cm thick. How much water can the tank hold?
_______ cm³

Answer: So tank can hold 37,352 cm³

Explanation: As Volume= LBH
Let’s find the inner dimensions of the tank, so 60-2 × 30-2 × 24-1
= 58×28×23
= 37,352 cm³

Question 2.
What if, to provide greater strength, the glass bottom were increased to a thickness of 4 cm? How much less water would the tank hold?
_______ cm³

Answer: 4,872 cm³

Explanation: As the glass bottom was increased to a thickness of 4 cm, 60-2 × 30-2 × 24-4
= 58×28×20
= 32,480 cm³
So the tank can hold 37,352- 32,480= 4,872 cm³

Question 3.
An aquarium tank in the shape of a rectangular prism is 40 cm long, 26 cm wide, and 24 cm high. If the top of the tank is open, how much tinting is needed to cover the glass on the tank? Identify the measure you used to solve the problem.
_______ cm³

Answer: 4,208 cm³  tinting needed to cover the glass on the tank.

Explanation:
The lateral area of the two faces is 26×24= 624 cm²
The lateral area of the other two faces is 40×24= 960 cm²
And the area of the top and bottom is 40×26= 1040 cm²
So the surface area of the tank without the top is 2×624 + 2×960 + 1040
= 1,248+1,920+1,040
= 4,208 cm³

Question 4.
The Louvre Museum in Paris, France, has a square pyramid made of glass in its central courtyard. The four triangular faces of the pyramid have bases of 35 meters and heights of 27.8 meters. What is the area of glass used for the four triangular faces of the pyramid?

Answer: 1946 m²

Explanation: The area of one face is 1/2 × 35 × 27.8= 486.5 m²
And the area of glass used for the four triangular faces of the pyramid is 4×486.5= 1946 m²

On Your Own – Page No. 638

Question 5.
A rectangular prism-shaped block of wood measures 3 m by 1 \(\frac{1}{2}\) m by 1 \(\frac{1}{2}\) m. How much of the block must a carpenter carve away to obtain a prism that measures 2 m by \(\frac{1}{2}\) m by \(\frac{1}{2}\) m?
_______ \(\frac{□}{□}\) m³

Answer: 6 1/4 m³

Explanation: The volume of the original block= LWH
= 3 × 1 1/2 × 1 1/2
= 3× 3/2 × 3/2
= 27/4
= 6 3/4 m²
And volume of carpenter carve is 2× 1/2 × 1/2
= 1/2 m²
So, the carpenter must carve 27/4 – 1/2
= 25/2
= 6 1/4 m³

Question 6.
The carpenter (Problem 5) varnished the outside of the smaller piece of wood, all except for the bottom, which measures \(\frac{1}{2}\) m by \(\frac{1}{2}\) m. Varnish costs $2.00 per square meter. What was the cost of varnishing the wood?
$ _______

Answer: $8.50

Explanation: The area of two lateral faces are 2×1/2= 1 m²
The area of the other two lateral faces are 2×1/2= 1 m²
The area of the top and bottom is 1/2×1/2= 1/4 m²
And the surface area is 2×1 + 2×1 + 1/4
= 2+2+1/4
= 17/4
= 4.25 m²
And the cost of vanishing the wood is $2.00× 4.25= $8.50

Question 7.
A wax candle is in the shape of a cube with a side length of 2 \(\frac{1}{2}\) in. What volume of wax is needed to make the candle?
_______ \(\frac{□}{□}\) in.³

Answer:

Explanation: The Volume of wax is needed to make the candle is= LWH
= 2 1/2 × 2 1/2 × 2 1/2
= 5/2 × 5/2 × 5/2
= 125/8
= 15 5/8 in.³

Question 8.
Describe A rectangular prism-shaped box measures 6 cm by 5 cm by 4 cm. A cube-shaped box has a side length of 2 cm. How many of the cube-shaped boxes will fit into the rectangular prismshaped box? Describe how you found your answer.

Answer: 12 cube-shaped boxes

Explanation: As 6 small boxes can fit on the base i.e 6 cm by 5 cm, as height is 4cm there can be a second layer of 6 small boxes. So, there will be a total of 12 cube-shaped boxes and will fit into a rectangular prism-shaped box

Question 9.
Justin is covering the outside of an open shoe box with colorful paper for a class project. The shoe box is 30 cm long, 20 cm wide, and 13 cm high. How many square centimeters of paper are needed to cover the outside of the open shoe box? Explain your strategy
_______ cm²

Answer: 1,900 cm²

Explanation:
The area of the two lateral faces of the shoebox is 20×13= 260 cm²
The area of another two lateral faces of the shoebox is 30×13= 390 cm²
The area of the top and bottom is 30×20= 600 cm²
So, the surface area of the shoebox without the top is 2×260 + 2× 390 + 600
= 520+780+600
= 1,900 cm²

Problem Solving Geometric Measurements – Page No. 639

Read each problem and solve.

Question 1.
The outside of an aquarium tank is 50 cm long, 50 cm wide, and 30 cm high. It is open at the top. The glass used to make the tank is 1 cm thick. How much water can the tank hold?
_______ cm³

Answer: So water tank can hold 66,816 cm³

Explanation: The volume of inner dimensions of the aquarium is 50-2 × 50-2 × 30-1
= 48×48×29
= 66,816 cm³
So water tank can hold 66,816 cm³

Question 2.
Arnie keeps his pet snake in an open-topped glass cage. The outside of the cage is 73 cm long, 60 cm wide, and 38 cm high. The glass used to make the cage is 0.5 cm thick. What is the inside volume of the cage?
_______ cm³

Answer: The volume of the cage is 1,59,300 cm³

Explanation: The volume of inner dimensions is 73-1 × 60-1 × 38-0.5
= 72×59×37.5
= 1,59,300 cm³
So, the volume of the cage is 1,59,300 cm³

Question 3.
A display number cube measures 20 in. on a side. The sides are numbered 1–6. The odd-numbered sides are covered in blue fabric and the even-numbered sides are covered in red fabric. How much red fabric was used?
_______ in.²

Answer: 1200 in.²

Explanation: The area of each side of a cube is 20×20= 400 in.², as there are 3 even-numbered sides on the cube. So there will be
3×400= 1200 in.²

Question 4.
The caps on the tops of staircase posts are shaped like square pyramids. The side length of the base of each cap is 4 inches. The height of the face of each cap is 5 inches. What is the surface area of the caps for two posts?
_______ in.²

Answer: 112 in.²

Explanation: The area of the base is 4×4= 16 in.²
The area of one face is 1/2×5×4= 10 in.²
The surface area of one cap is 16+4×10
= 16+40
= 56 in.²
And the surface area of the caps for two posts is 2×56= 112 in.²

Question 5.
A water irrigation tank is shaped like a cube and has a side length of 2 \(\frac{1}{2}\) feet. How many cubic feet of water are needed to completely fill the tank?
_______ \(\frac{□}{□}\) ft³

Answer: 15 5/8 ft³

Explanation: Volume= LWH
= 2 1/2 × 2 1/2 × 2 1/2
= 5/2 × 5/2 × 5/2
= 125/8
= 15 5/8 ft³

Question 6.
Write and solve a problem for which you use part of the formula for the surface area of a triangular prism.

Answer: In a triangular prism, the triangular end has a base of 5cm and the height is 8 cm. The length of each side is 4 cm and the height of the prism is 10 cm. What is the lateral area of this triangular prism?

Explanation: The area of two triangular faces is 1/2 × 5 × 8
= 5×4
= 20 cm²
The area of two rectangular faces is 4×10= 40 cm²
The lateral area is 2×20+2×40
= 40+80
= 120 cm²

Lesson Check – Page No. 640

Question 1.
Maria wants to know how much wax she will need to fill a candle mold shaped like a rectangular prism. What measure should she find?

Answer: Maria needs to find the volume of the mold.

Question 2.
The outside of a closed glass display case measures 22 inches by 15 inches by \(\frac{1}{2}\) inches. The glass is 12 inch thick. How much air is contained in the case?
_______ in.³

Answer: 3381 in.³

Explanation: The inner dimensions are 22-1× 15-1 × 12- 1/2
= 21 ×14×23/2
= 3381 in.³

Spiral Review

Question 3.
A trapezoid with bases that measure 5 centimeters and 7 centimeters has a height of 4.5 centimeters. What is the area of the trapezoid?
_______ cm²

Answer: 27 cm²

Explanation: Area of trapezoid= 1/2 ×(7+5)×4.5
= 6×4.5
= 27 cm²

Question 4.
Sierra has plotted two vertices of a rectangle at (3, 2) and (8, 2). What is the length of the side of the rectangle?
_______ units

Answer: 5 units.

Explanation: The length of the side of the rectangle is 8-3= 5 units.

Question 5.
What is the surface area of the square pyramid?

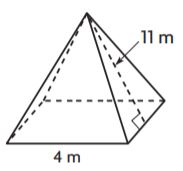
_______ m²

Answer: 104 m²

Explanation: The area of the base 4×4= 16
The area of the one face is 1/2 × 4 × 11
= 2×11
= 22 m²
The surface area of the square pyramid is 16+4×22
= 16+88
= 104 m²

Question 6.
A shipping company has a rule that all packages must be rectangular prisms with a volume of no more than 9 cubic feet. What is the maximum measure for the height of a box that has a width of 1.5 feet and a length of 3 feet?
_______ feet

Answer: 2 feet.

Explanation: As given volume = 9 cubic feet
So 1.5×3×H < 9
4.5×H < 9
H< 9/4.5
and H<2
So maximum measure for the height of the box is 2 feet.

Chapter 11 Review/Test – Page No. 641

Question 1.
Elaine makes a rectangular pyramid from paper.
The base is a _____. The lateral faces are _____.
The base is a ___________ .
The lateral faces are ___________ .

Answer:
The base is a rectangle.
The lateral faces are triangles.

Question 2.
Darrell paints all sides except the bottom of the box shown below.

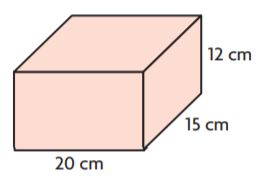
Select the expressions that show how to find the surface area that Darrell painted. Mark all that apply.
Options:
a. 240 + 240 + 180 + 180 + 300 + 300
b. 2(20 × 12) + 2(15 × 12) + (20 × 15)
c. (20 × 12) + (20 × 12) + (15 × 12) + (15 × 12) + (20 × 15)
d. 20 × 15 × 12

Answer: b,c

Explanation: The expressions that show how to find the surface area is 2(20 × 12) + 2(15 × 12) + (20 × 15), (20 × 12) + (20 × 12) + (15 × 12) + (15 × 12) + (20 × 15)

Question 3.
A prism is filled with 44 cubes with \(\frac{1}{2}\)-unit side lengths. What is the volume of the prism in cubic units?
_______ \(\frac{□}{□}\) cubic unit

Answer:

Explanation:
The volume of a cube with S= (1/2)³
= 1/2×1/2×1/2
= 1/8
= 0.125 cubic units
As there are 44 cubes so 44×0.125=5.5 cubic units.

Question 4.
A triangular pyramid has a base with an area of 11.3 square meters, and lateral faces with bases of 5.1 meters and heights of 9 meters. Write an expression that can be used to find the surface area of the triangular pyramid.

Answer: 11.3+ 3 × 1/2+ 5.1×9

Explanation: The expression that can be used to find the surface area of the triangular pyramid is 11.3+ 3 × 1/2+ 5.1×9

Page No. 642

Question 5.
Jeremy makes a paperweight for his mother in the shape of a square pyramid. The base of the pyramid has a side length of 4 centimeters, and the lateral faces have heights of 5 centimeters. After he finishes, he realizes that the paperweight is too small and decides to make another one. To make the second pyramid, he doubles the length of the base in the first pyramid.
For numbers 5a–5c, choose Yes or No to indicate whether the statement is correct.
5a. The surface area of the second pyramid is 144 cm².
5b. The surface area doubled from the first pyramid to the second pyramid.
5c. The lateral area doubled from the first pyramid to the second pyramid.
5a. ___________
5b. ___________
5c. ___________

Answer:
5a. True.
5b. False
5c. True.

Explanation:
The area of the base is 4×4= 16 cm².
The area of one face is 1/2×4×5
= 2×5
= 10 cm².
The surface area of the First pyramid is 16+ 4×10
= 16+40
= 56 cm².
The area of the base is 8×8= 64
The area of one face is 1/2×8×5
= 4×5
= 20 cm².
The surface area od the second pyramid is 64+ 4×20
= 64+80
= 144 cm².

Question 6.
Identify the figure shown and find its surface area. Explain how you found your answer.

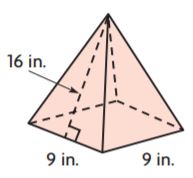
Answer: 369 in²

Explanation:
The area of the base is 9×9= 81 in²
The area of one face is 1//2 × 16× 9
= 8×9
= 72 in²
The surface area of a square pyramid is 81+ 4× 72
= 81+ 288
= 369 in²

Question 7.
Dominique has a box of sewing buttons that is in the shape of a rectangular prism.
The volume of the box is 2 \(\frac{1}{2}\) in. × 3 \(\frac{1}{2}\) in. × _____ = _____.

Answer: 17.5 in³

Explanation: The volume of the box is 2 1/2 × 3 1/2 × 2
= 5/2 × 7/2 × 2
= 5/2 × 7
= 35/2
= 17.5 in³

Page No. 643

Question 8.
Emily has a decorative box that is shaped like a cube with a height of 5 inches. What is the surface area of the box?
_______ in.²

Answer: 150 in.²

Explanation: Surface area of the box is 6 a²
So 6 × 5²
= 6×5×5²
= 150 in.²

Question 9.
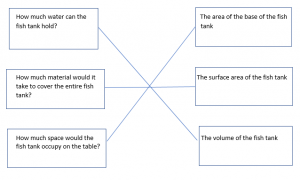
Albert recently purchased a fish tank for his home. Match each question with the geometric measure that would be most appropriate for each scenario.

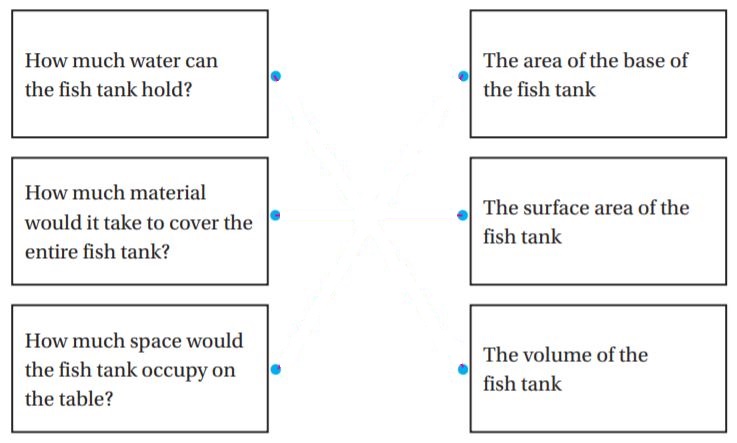
Answer:

Question 10.
Select the expressions that show the volume of the rectangular prism. Mark all that apply.
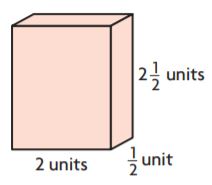Options:
a. 2(2 units × 2 \(\frac{1}{2 }\) units) + 2(2 units × \(\frac{1}{2}\) unit) + 2(\(\frac{1}{2}\) unit × 2 \(\frac{1}{2}\) units)
b. 2(2 units × \(\frac{1}{2}\) unit) + 4(2 units × 2 \(\frac{1}{2}\) units)
c. 2 units × \(\frac{1}{2}\) unit × 2 \(\frac{1}{2}\) units
d. 2.5 cubic units

Answer: c, d

Explanation: 2 units ×1/2 unit × 2 1/2 units and 2.5 cubic units

Page No. 644

Question 11.
For numbers 11a–11d, select True or False for the statement.

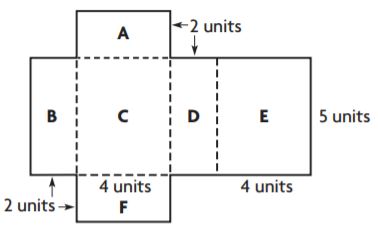
11a. The area of face A is 8 square units.
11b. The area of face B is 10 square units.
11c. The area of face C is 8 square units.
11d. The surface area of the prism is 56 square units.
11a. ___________
11b. ___________
11c. ___________
11d. ___________

Answer:
11a. True.
11b. True.
11c. False.
11d. False.

Explanation:
The area of the face A is 4×2= 8 square units
The area of the face B is 5×2= 10 square units
The area of the face C is 5×4= 20 square units
So the surface area is 2×8+2×10+2×20
= 16+20+40
= 76 square units

Question 12.
Stella received a package in the shape of a rectangular prism. The box has a length of 2 \(\frac{1}{2}\) feet, a width of 1 \(\frac{1}{2}\) feet, and a height of 4 feet.
Part A
Stella wants to cover the box with wrapping paper. How much paper will she need? Explain how you found your answer

Answer: 39.5 ft²

Explanation:
The area of two lateral faces is 4 × 2 1/2
= 4 × 5/2
= 2×5
= 10 ft²
The area of another two lateral faces is 4 × 1 1/2
= 4 × 3/2
= 2×3
= 6 ft²
The area of the top and bottom is 2 1/2 × 1 1/2
= 5/2 × 3/2
= 15/4
= 3 3/4 ft²
So Stella need 2×10+ 2×6 + 2 × 15/4
= 20+ 12+15/2
= 20+12+7.5
= 39.5 ft²

Question 12.
Part B
Can the box hold 16 cubic feet of packing peanuts? Explain how you know

Answer: The box cannot hold 16 cubic feet of the packing peanuts

Explanation: Volume = LWH
= 2 1/2 ×1 1/2 × 4
= 5/2 × 3/2 ×4
= 5×3
= 15 ft³
So the box cannot hold 16 cubic feet of the packing peanuts.

Page No. 645

Question 13.
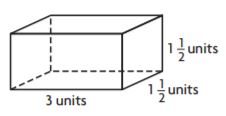
A box measures 6 units by \(\frac{1}{2}\) unit by 2 \(\frac{1}{2}\) units.
For numbers 13a–13b, select True or False for the statement.
13a. The greatest number of cubes with a side length of \(\frac{1}{2}\) unit that can be packed inside the box is 60.
13b. The volume of the box is 7 \(\frac{1}{2}\) cubic units.
13a. ___________
13b. ___________

Answer:
13a. True
13b. True.

Explanation:
Length is 12 × 1/2= 6 units
Width is 1× 1/2= 1/2 units
Height is 5× 1/2= 5/2 units
So, the greatest number of cubes with a side length of 1/2 unit that can be packed inside the box is 12×1×5= 60
The volume of the cube is S³
The volume of a cube with S= (1/2)³
= 1/2×1/2×1/2
= 1/8
= 0.125 cubic units
As there are 60 cubes so 60×0.125= 7.5cubic units.

Question 14.
Bella says the lateral area of the square pyramid is 1,224 in.². Do you agree or disagree with Bella? Use numbers and words to support your answer. If you disagree with Bella, find the correct answer.

Answer: 900 in²

Explanation:
Area= 4× 1/2 bh
= 4× 1/2 × 18 × 25
= 2× 18 × 25
=  900 in²
So lateral area is 900 in², so I disagree

Question 15.
Lourdes is decorating a toy box for her sister. She will use self-adhesive paper to cover all of the exterior sides except for the bottom of the box. The toy box is 4 feet long, 3 feet wide, and 2 feet high. How many square feet of adhesive paper will Lourdes use to cover the box?
_______ ft²

Answer: 40 ft²

Explanation:
The area of two lateral faces is 4×2= 8 ft²
The area of another two lateral faces is 3×2= 6 ft²
The area of the top and bottom is 4×3= 12 ft²
So Lourdes uses to cover the box is 2×8 + 2×6 + 12
= 16+12+12
= 40 ft²

Question 16.
Gary wants to build a shed shaped like a rectangular prism in his backyard. He goes to the store and looks at several different options. The table shows the dimensions and volumes of four different sheds. Use the formula V = l × w × h to complete the table.

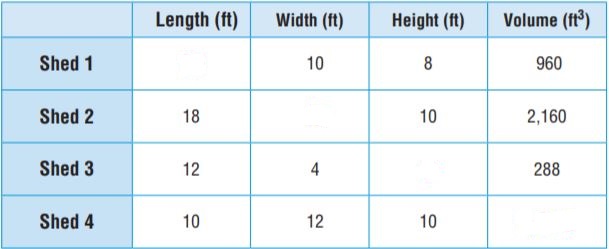
Answer:
Length of shed 1= 12 ft
Width of shed 2= 12 ft
Height of shed 3= 6 ft
Volume of shed 4= 1200 ft³

Explanation: Volume= LWH
Volume of shed1= 960 ft
So 960= L×10×8
960= 80×L
L= 960/80
L= 12 ft
Volume of shed2= 2160 ft
So 2160= 18×W×10
960= 180×W
W= 2160/180
W= 12 ft
Volume of shed3= 288 ft
So 288= 12×4×H
288= 48×H
H= 288/48
W= 6 ft
Volume of shed2= 10×12×10
So V= 10×12×10
V= 1200 ft³

Page No. 646

Question 17.
Tina cut open a cube-shaped microwave box to see the net. How many square faces does this box have?
_______ square faces

Answer: The box has 6 square faces.

Question 18.
Charles is painting a treasure box in the shape of a rectangular prism.
Which nets can be used to represent Charles’ treasure box? Mark all that apply.
Options:
a.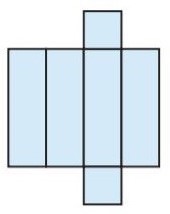
b.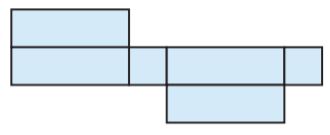
c.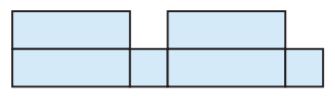
d.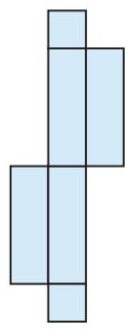

Answer: a and b can be used to represent Charle’s treasure box.

Question 19.
Julianna is lining the inside of a basket with fabric. The basket is in the shape of a rectangular prism that is 29 cm long, 19 cm wide, and 10 cm high. How much fabric is needed to line the inside of the basket if the basket does not have a top? Explain your strategy.
_______ cm²

Answer: 1511 cm²

Explanation: The surface area= 2(WL+HL+HW)
The surface area of the entire basket= 2(19×29)+2(10×29)+2(10×19)
= 2(551)+2(290)+2(190)
= 1102+580+380
= 2,062 cm²
The surface area of the top is 29×19= 551
So Julianna needs 2062-551= 1511 cm²